Improving the Chamberlin Digital State Variable Filter

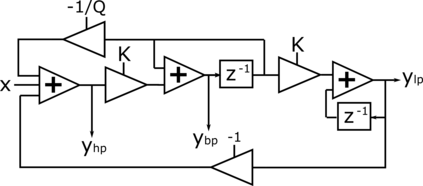
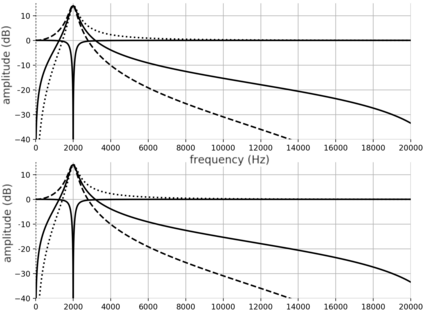
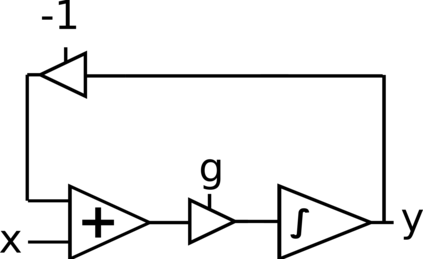
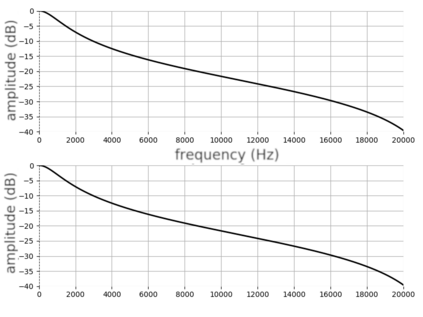
The state variable filter configuration is a classic analogue design which has been employed in many electronic music applications. A digital implementation of this filter was put forward by Chamberlin, which has been deployed in both software and hardware forms. While this has proven to be a straightforward and successful digital filter design, it suffers from some issues, which have already been identified in the literature. From a modified Chamberlin block diagram, we derive the transfer functions describing its three basic responses, highpass, bandpass, and lowpass. An analysis of these leads to the development of an improvement, which attempts to better shape the filter spectrum. From these new transfer functions, a set of filter equations is developed. Finally, the approach is compared to an alternative time-domain based re-organisation of update equations, which is shown to deliver a similar result.