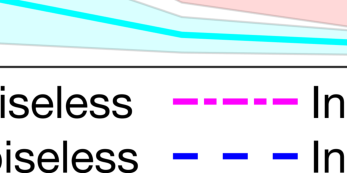
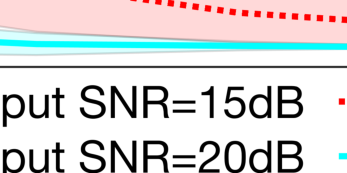
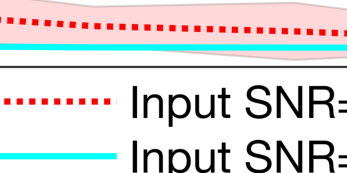
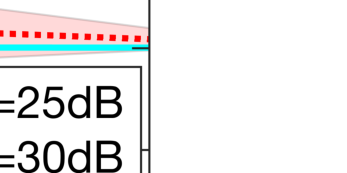
In several applications, including imaging of deformable objects while in motion, simultaneous localization and mapping, and unlabeled sensing, we encounter the problem of recovering a signal that is measured subject to unknown permutations. In this paper we take a fresh look at this problem through the lens of optimal transport (OT). In particular, we recognize that in most practical applications the unknown permutations are not arbitrary but some are more likely to occur than others. We exploit this by introducing a regularization function that promotes the more likely permutations in the solution. We show that, even though the general problem is not convex, an appropriate relaxation of the resulting regularized problem allows us to exploit the well-developed machinery of OT and develop a tractable algorithm.
翻译:在若干应用中,包括运动中变形物体的成像、同步定位和绘图以及无标签的感应,我们遇到了恢复一个信号的问题,该信号在测量时受到未知的变异性的影响。在本文件中,我们通过最佳运输(OT)的透镜重新审视这一问题。特别是,我们认识到,在大多数实际应用中,未知变异不是任意的,但有些变异比其他更可能发生。我们利用这一功能,引入一种正规化功能,促进解决办法中更可能的变异性。我们表明,尽管一般的问题不是相互交错,但由此产生的正常化问题得到适当缓解,使我们能够利用成熟的OT机器,并开发一种可移植的算法。