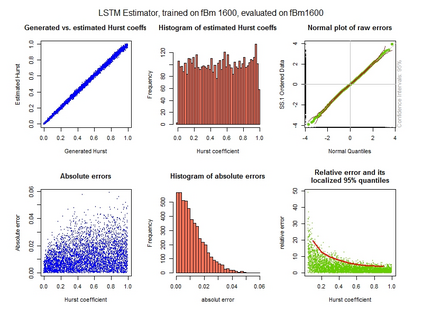
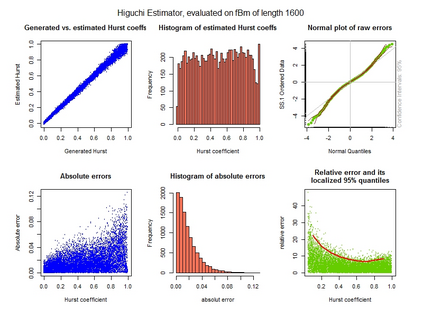
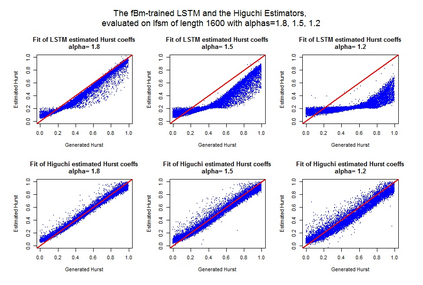
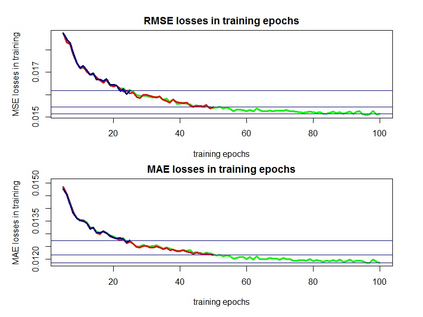
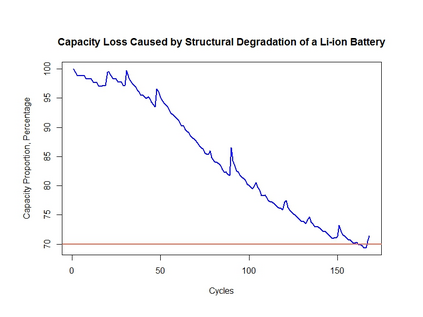
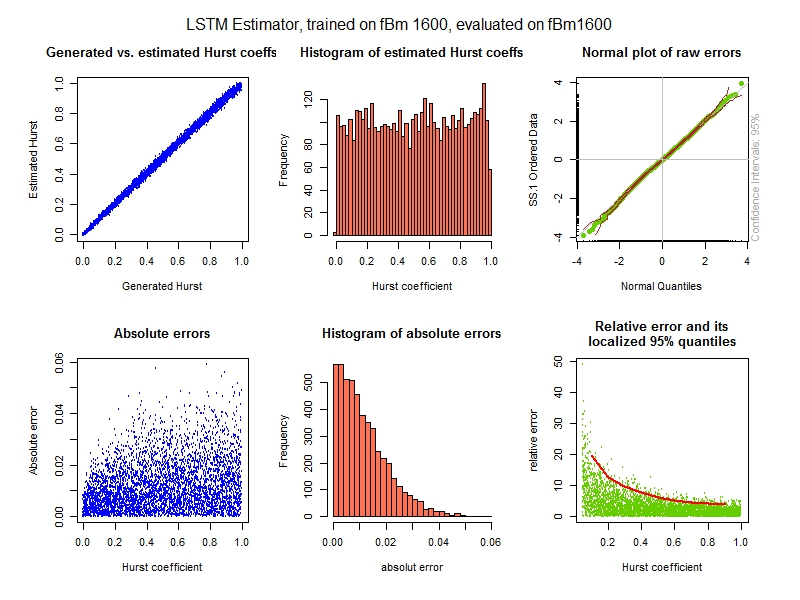
This research explores the reliability of deep learning, specifically Long Short-Term Memory (LSTM) networks, for estimating the Hurst parameter in fractional stochastic processes. The study focuses on three types of processes: fractional Brownian motion (fBm), fractional Ornstein-Uhlenbeck (fOU) process, and linear fractional stable motions (lfsm). The work involves a fast generation of extensive datasets for fBm and fOU to train the LSTM network on a large volume of data in a feasible time. The study analyses the accuracy of the LSTM network's Hurst parameter estimation regarding various performance measures like RMSE, MAE, MRE, and quantiles of the absolute and relative errors. It finds that LSTM outperforms the traditional statistical methods in the case of fBm and fOU processes; however, it has limited accuracy on lfsm processes. The research also delves into the implications of training length and valuation sequence length on the LSTM's performance. The methodology is applied by estimating the Hurst parameter in Li-ion battery degradation data and obtaining confidence bounds for the estimation. The study concludes that while deep learning methods show promise in parameter estimation of fractional processes, their effectiveness is contingent on the process type and the quality of training data.
翻译:暂无翻译