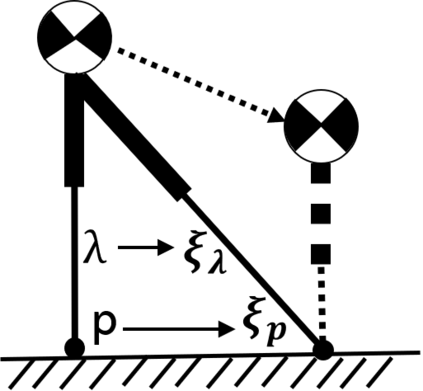
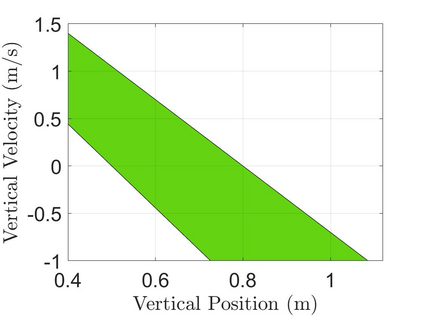
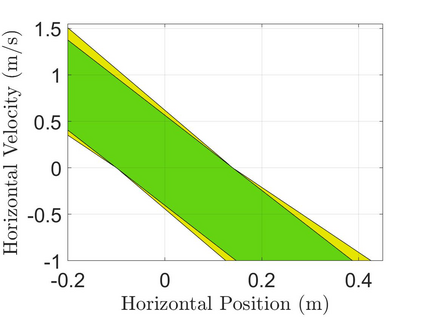
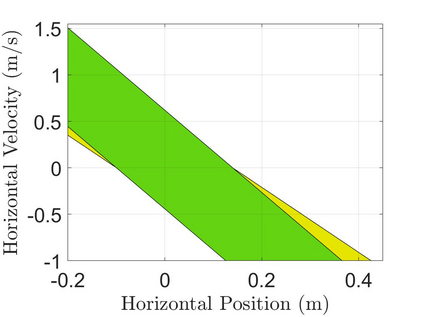
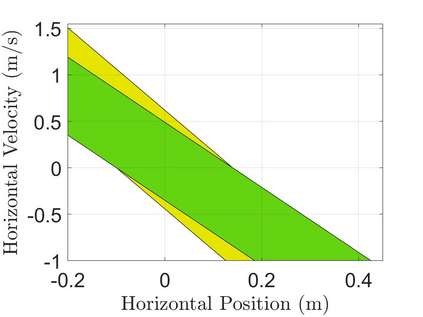
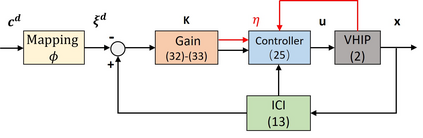
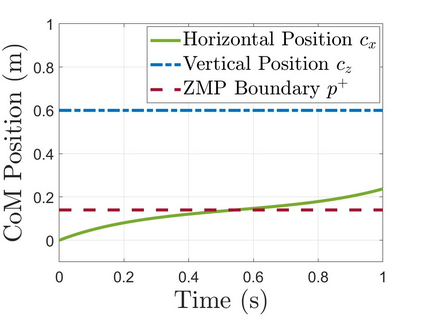
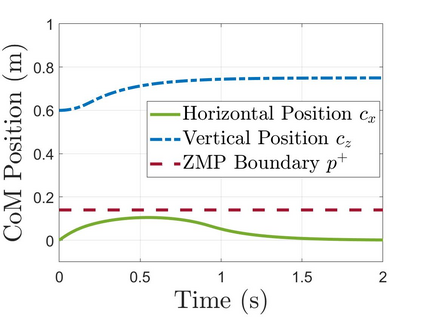
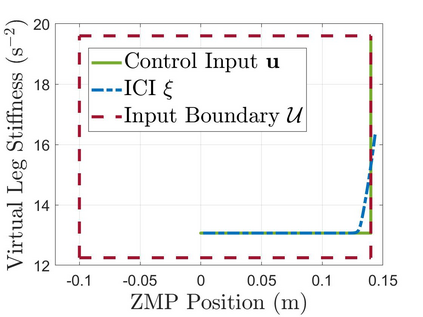
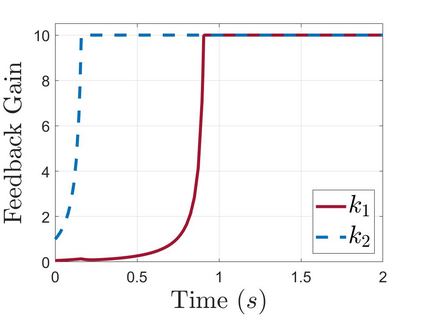
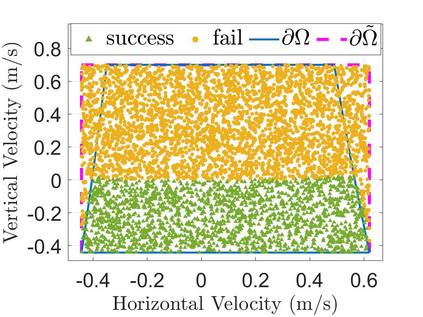
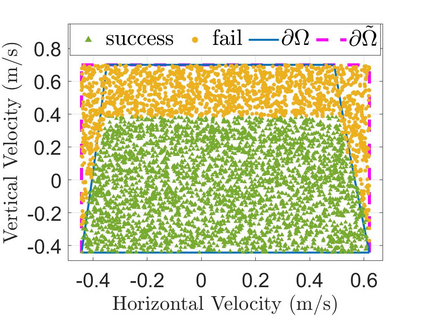
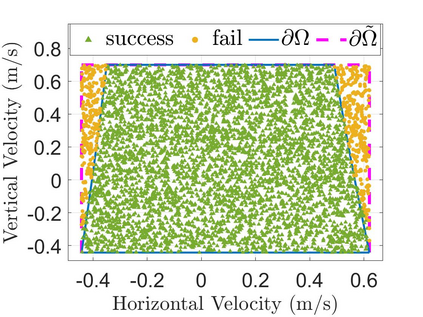
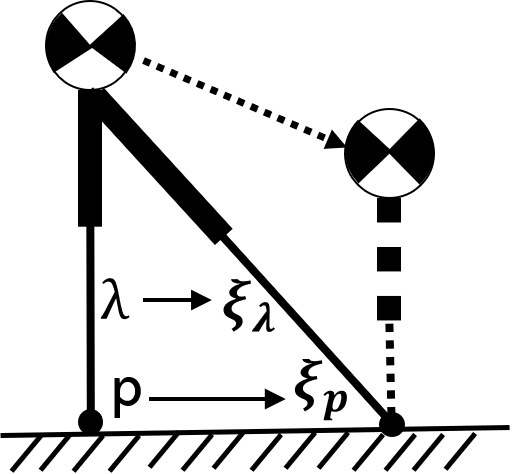
Balancing is a fundamental need for legged robots due to their unstable floating-base nature. Balance control has been thoroughly studied for simple models such as the linear inverted pendulum thanks to the concept of the instantaneous capture point (ICP), yet the constant center of mass height assumption limits the application. This paper explores balancing of the variable-height inverted pendulum (VHIP) model by introducing the \emph{instantaneous capture input} (ICI), an extension of the ICP based on its key properties. Namely, the ICI can be computed as a function of the state, and when this function is used as the control policy, the ICI is rendered stationary and the system will eventually come to a stop. This characterization induces an analytical region of capturable states for the VHIP, which can be used to conceptually guide where to step. To further address state and control constraints during recovery, we present and theoretically analyze an explicit ICI-based controller with online optimal feedback gains. Simulations demonstrate the validity of our controller for capturability maintenance compared to an approach based on the divergent component of motion.
翻译:由于悬浮基质的不稳定性,平衡是腿状机器人的基本需要。由于瞬时捕捉点的概念,对线性倒置钟盘等简单模型的平衡控制已经进行了彻底研究,但质量高度的常数中心假设限制了应用。本文探讨平衡可变高度倒置钟盘(VHIP)模型,引入了 emph{intantaneous 抓取输入 (ICI) (VHIP),这是比较方案基于关键特性的延伸。 也就是说, ICI 可以作为状态的函数来计算, 当该功能被用作控制政策时, ICI 变成静止的, 系统最终会停止 。 这个特征将引出一个分析性区域, 分析VHIP的可控制状态, 用于概念性指导前进的方向。 为了进一步解决恢复过程中的状态和控制限制, 我们提出并理论上分析一个明确的 ICI 控制器, 其在线最佳反馈收益。 模拟显示我们的控制器对可控性维护能力的有效性, 与基于不同运动部分的方法相比, 。