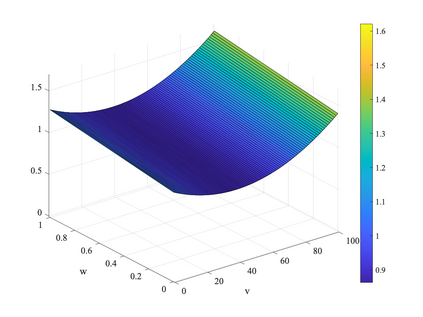
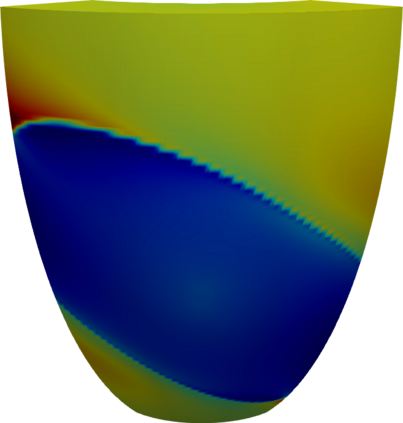
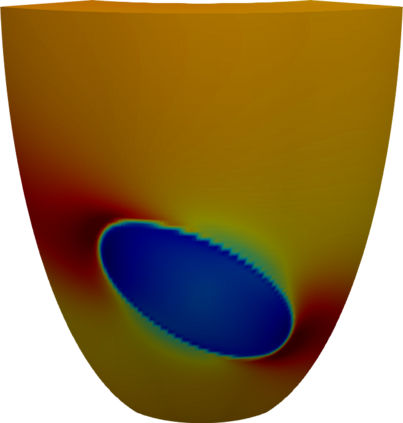
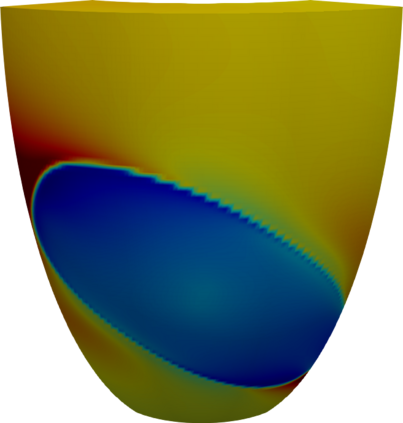
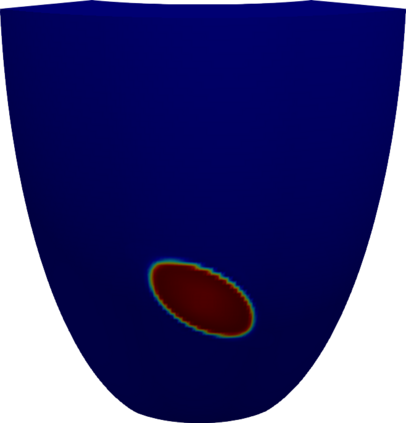
Two novel parallel Newton-Krylov Balancing Domain Decomposition by Constraints (BDDC) and Dual-Primal Finite Element Tearing and Interconnecting (FETI-DP) solvers are here constructed, analyzed and tested numerically for implicit time discretizations of the three-dimensional Bidomain system of equations. This model represents the most advanced mathematical description of the cardiac bioelectrical activity and it consists of a degenerate system of two non-linear reaction-diffusion partial differential equations (PDEs), coupled with a stiff system of ordinary differential equations (ODEs). A finite element discretization in space and a segregated implicit discretization in time, based on decoupling the PDEs from the ODEs, yields at each time step the solution of a non-linear algebraic system. The Jacobian linear system at each Newton iteration is solved by a Krylov method, accelerated by BDDC or FETI-DP preconditioners, both augmented with the recently introduced {\em deluxe} scaling of the dual variables. A polylogarithmic convergence rate bound is proven for the resulting parallel Bidomain solvers. Extensive numerical experiments on linux clusters up to two thousands processors confirm the theoretical estimates, showing that the proposed parallel solvers are scalable and quasi-optimal.
翻译:纽顿- 克利洛夫( Newton- Krylov) 平衡 Domain 由约束因素( BDDCC) 和 双极有限元素撕裂和互连( FETI- DP) 解析( FETI- DP) 解析在此构建、分析和用数字方式测试三维 Bidomain 等式系统隐含的时间分解。 这个模型代表了对心脏生物电动活动的最先进的数学描述,它由两种非线性反射扩散部分差异方程式( PDE) 的退化系统组成,加上一个硬性的普通差异方程式系统( ODEs ) 。 在将 PDEs与 ODEs脱钩的基础上,空间的有限元素分解和分离的隐性隐性分解( FETI- DP) 解解解解( FETI- DP) 解解解解解( FETI- DP) 解解解解解解解解解解解解解解( ) 解( FTILO) 解( Fiallodal) lodalationalationslationalizlational rolational modal rolationslations) roducol ad rolation rolationslations roducol cols( ) ) cols( ) ) comml) cols( ) ) cols( ) 和( ) )