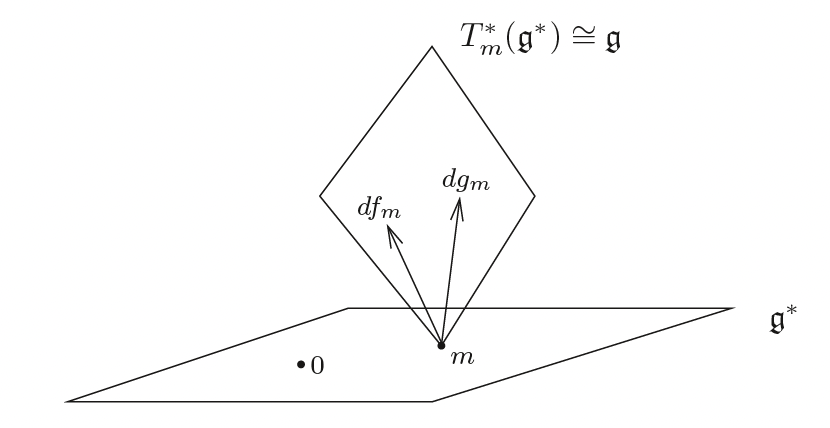
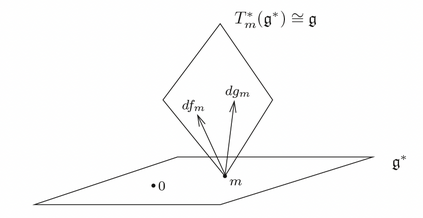
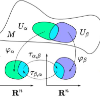
The study of diffeomorphism groups and their applications to problems in analysis and geometry has a long history. In geometric hydrodynamics, pioneered by V.~Arnold in the 1960s, one considers an ideal fluid flow as the geodesic motion on the infinite-dimensional group of volume-preserving diffeomorphisms of the fluid domain with respect to the metric defined by the kinetic energy. Similar considerations on the space of densities lead to a geometric description of optimal mass transport and the Kantorovich-Wasserstein metric. Likewise, information geometry associated with the Fisher-Rao metric and the Hellinger distance has an equally beautiful infinite-dimensional geometric description and can be regarded as a higher-order Sobolev analogue of optimal transportation. In this work we review various metrics on diffeomorphism groups relevant to this approach and introduce appropriate topology, smooth structures and dynamics on the corresponding infinite-dimensional manifolds. Our main goal is to demonstrate how, alongside topological hydrodynamics, Hamiltonian dynamics and optimal mass transport, information geometry with its elaborate toolbox has become yet another exciting field for applications of geometric analysis on diffeomorphism groups.
翻译:暂无翻译