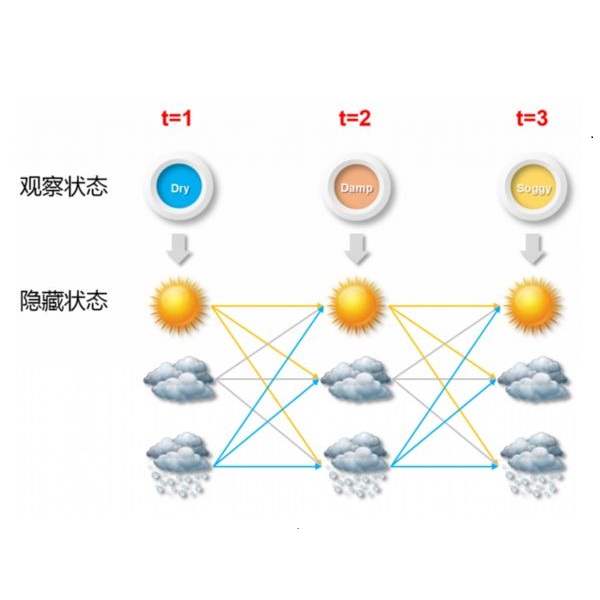
This paper presents algorithms for parallelization of inference in hidden Markov models (HMMs). In particular, we propose parallel backward-forward type of filtering and smoothing algorithm as well as parallel Viterbi-type maximum-a-posteriori (MAP) algorithm. We define associative elements and operators to pose these inference problems as parallel-prefix-sum computations in sum-product and max-product algorithms and parallelize them using parallel-scan algorithms. The advantage of the proposed algorithms is that they are computationally efficient in HMM inference problems with long time horizons. We empirically compare the performance of the proposed methods to classical methods on a highly parallel graphical processing unit (GPU).
翻译:本文介绍了隐藏的Markov 模型中平行推论的算法。 特别是,我们提出了平行的后向过滤和平滑算法,以及平行的Viterbi型最大- a- posteririi(MAP)算法。 我们将这些关联要素和操作者定义为在总和和和最大产品算法中平行的预留和计算,并使用平行扫描算法将它们平行。 提议的算法的优点是,在HMM 推论问题中,这些算法具有计算效率,具有较长的时间跨度。 我们用经验将拟议方法的性能与高度平行的图形处理单元(GPU)的经典方法作比较。