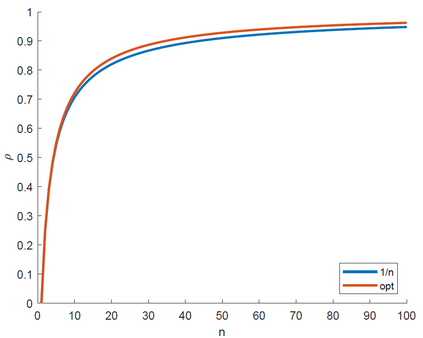
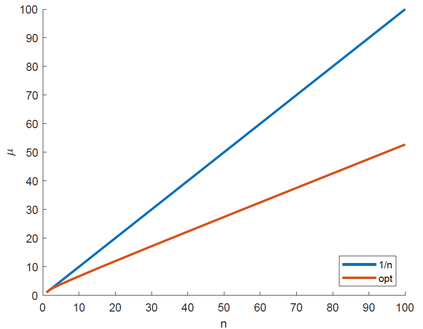
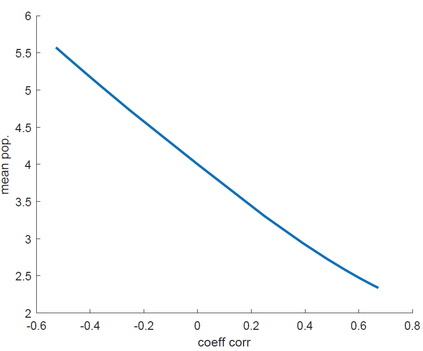
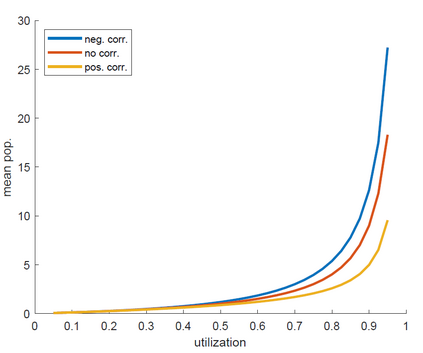
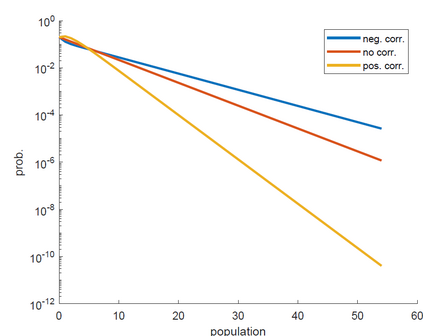
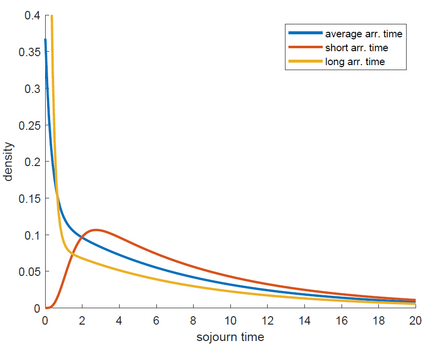
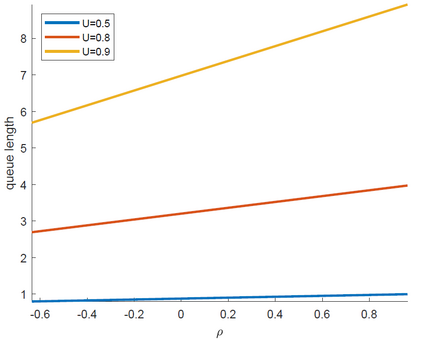
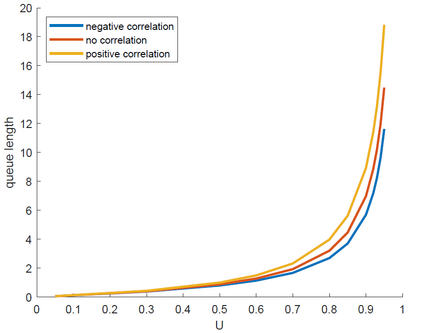
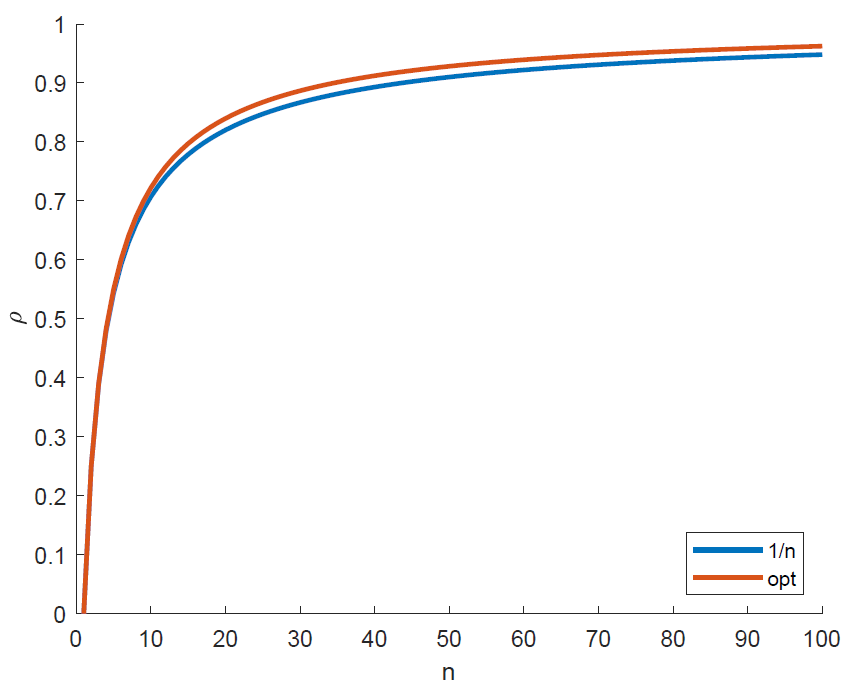
In this paper we present results for bivariate exponential distributions which are represented by phase type distributions. The paper extends results from previous publications [5, 14] on this topic by introducing new representations that require a smaller number of phases to reach some correlation coefficient and introduces different ways to describe correlation between exponentially distributed random variables. Furthermore, it is shown how Markovian Arrival Processes (MAPs) with exponential marginal distribution can be generated from the phase type representations of exponential distributions and how the results for exponential distributions can be applied to define correlated hyperexponential or Erlang distributions. As application examples we analyze two queueing models with correlated inter-arrival and service times.
翻译:在本文中,我们介绍了以阶段性分布方式表示的双变指数分布结果;本文件扩展了以前关于这一专题的出版物[5、14]的结果,引入了新的表述方式,要求较少的阶段达到某种相关系数,并采用不同方式描述指数分布随机变量之间的相互关系;此外,还展示了指数性分布分布的阶段性类型如何产生具有指数性边际分布的Markovian 抵达进程(MAPs),以及指数性分布结果如何用于界定相关超富裕分布或Erlang分布。作为应用实例,我们分析了两个与相关抵达时间和服务时间相关的排队模式。