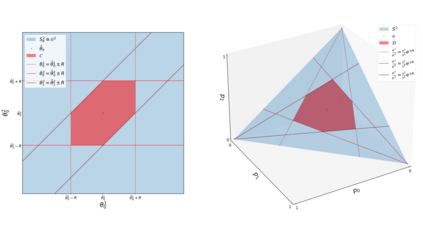
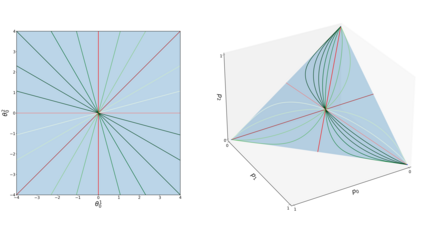
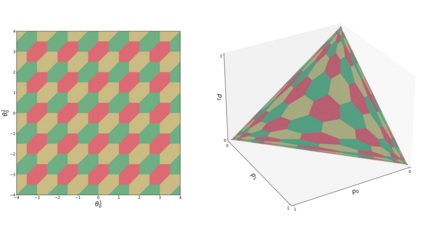
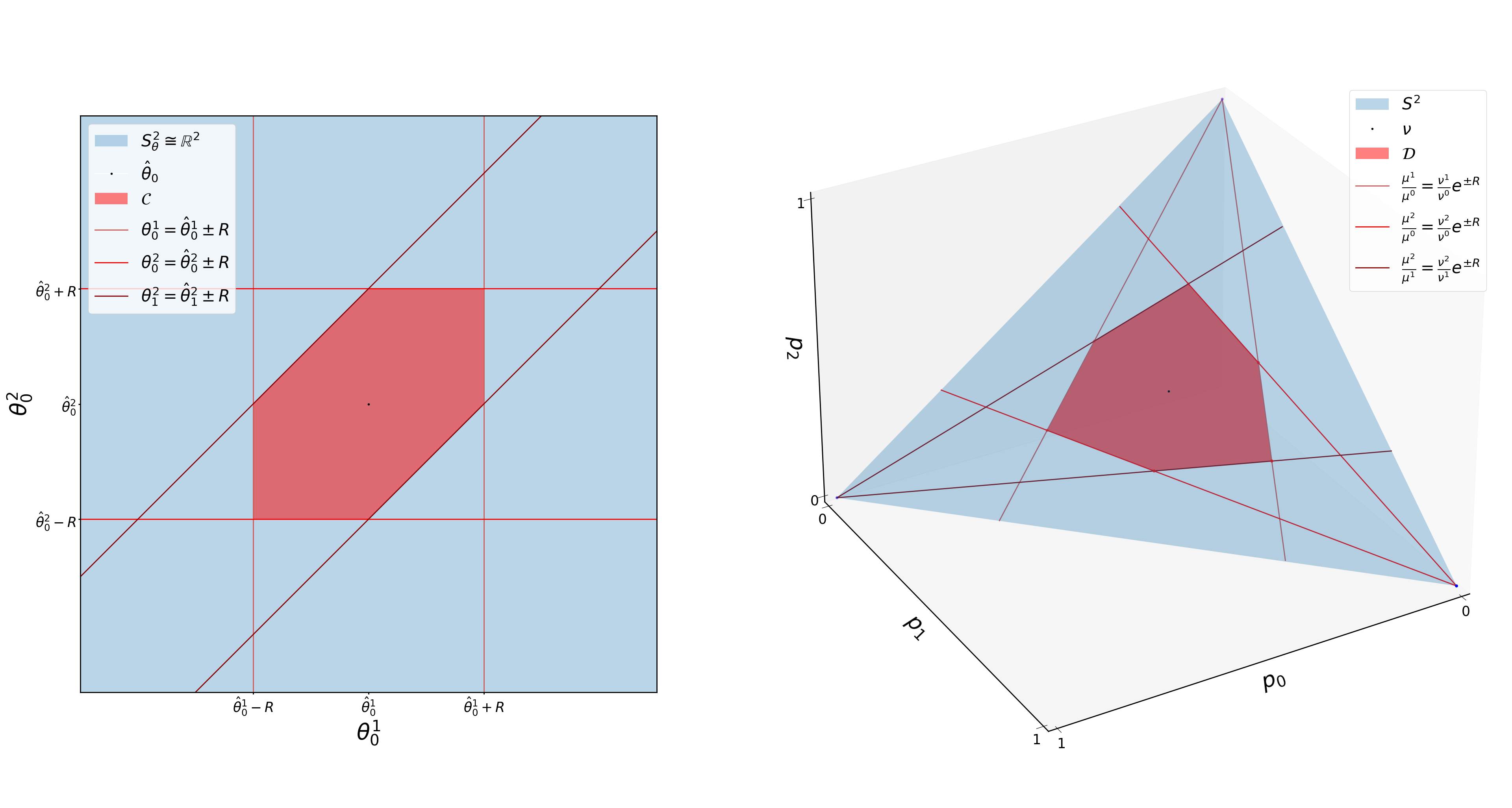
This paper gives a self-contained introduction to the Hilbert projective metric $\mathcal{H}$ and its fundamental properties, with a particular focus on the space of probability measures. We start by defining the Hilbert pseudo-metric on convex cones, focusing mainly on dual formulations of $\mathcal{H}$ . We show that linear operators on convex cones contract in the distance given by the hyperbolic tangent of $\mathcal{H}$, which in particular implies Birkhoff's classical contraction result for $\mathcal{H}$. Turning to spaces of probability measures, where $\mathcal{H}$ is a metric, we analyse the dual formulation of $\mathcal{H}$ in the general setting, and explore the geometry of the probability simplex under $\mathcal{H}$ in the special case of discrete probability measures. Throughout, we compare $\mathcal{H}$ with other distances between probability measures. In particular, we show how convergence in $\mathcal{H}$ implies convergence in total variation, $p$-Wasserstein distance, and any $f$-divergence. Furthermore, we derive a novel sharp bound for the total variation between two probability measures in terms of their Hilbert distance.
翻译:暂无翻译