On the Mathematics of Diffusion Models

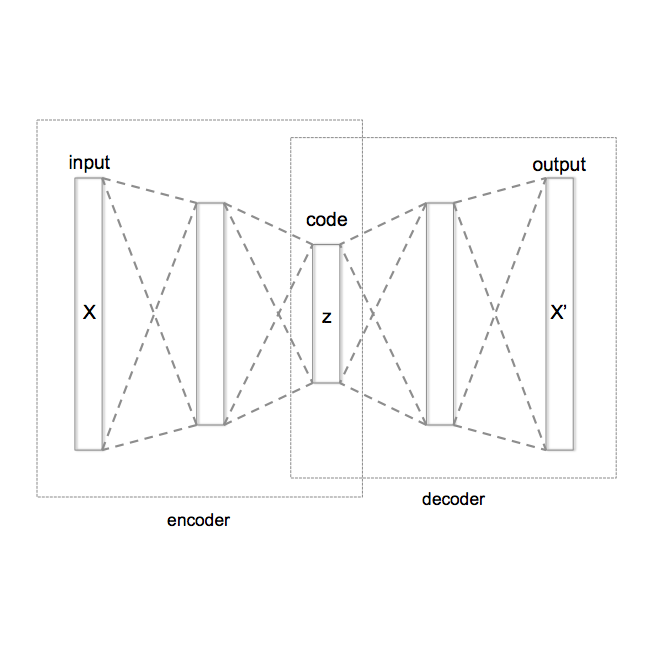
This paper attempts to present the stochastic differential equations of diffusion models in a manner that is accessible to a broad audience. The diffusion process is defined over a population density in R^d. Of particular interest is a population of images. In a diffusion model one first defines a diffusion process that takes a sample from the population and gradually adds noise until only noise remains. The fundamental idea is to sample from the population by a reverse-diffusion process mapping pure noise to a population sample. The diffusion process is defined independent of any ``interpretation'' but can be analyzed using the mathematics of variational auto-encoders (the ``VAE interpretation'') or the Fokker-Planck equation (the ``score-matching intgerpretation''). Both analyses yield reverse-diffusion methods involving the score function. The Fokker-Planck analysis yields a family of reverse-diffusion SDEs parameterized by any desired level of reverse-diffusion noise including zero (deterministic reverse-diffusion). The VAE analysis yields the reverse-diffusion SDE at the same noise level as the diffusion SDE. The VAE analysis also yields a useful expression for computing the population probabilities of a given point (image). This formula for the probability of a given point does not seem to follow naturally from the Fokker-Planck analysis. Much, but apparently not all, of the mathematics presented here can be found in the literature. Attributions are given at the end of the paper.