Root-max Problems, Hybrid Expansion-Contraction, and Quadratically Convergent Optimization of Passive Systems

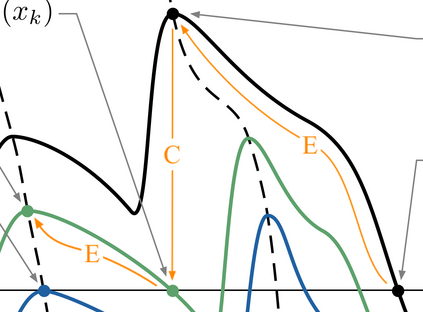
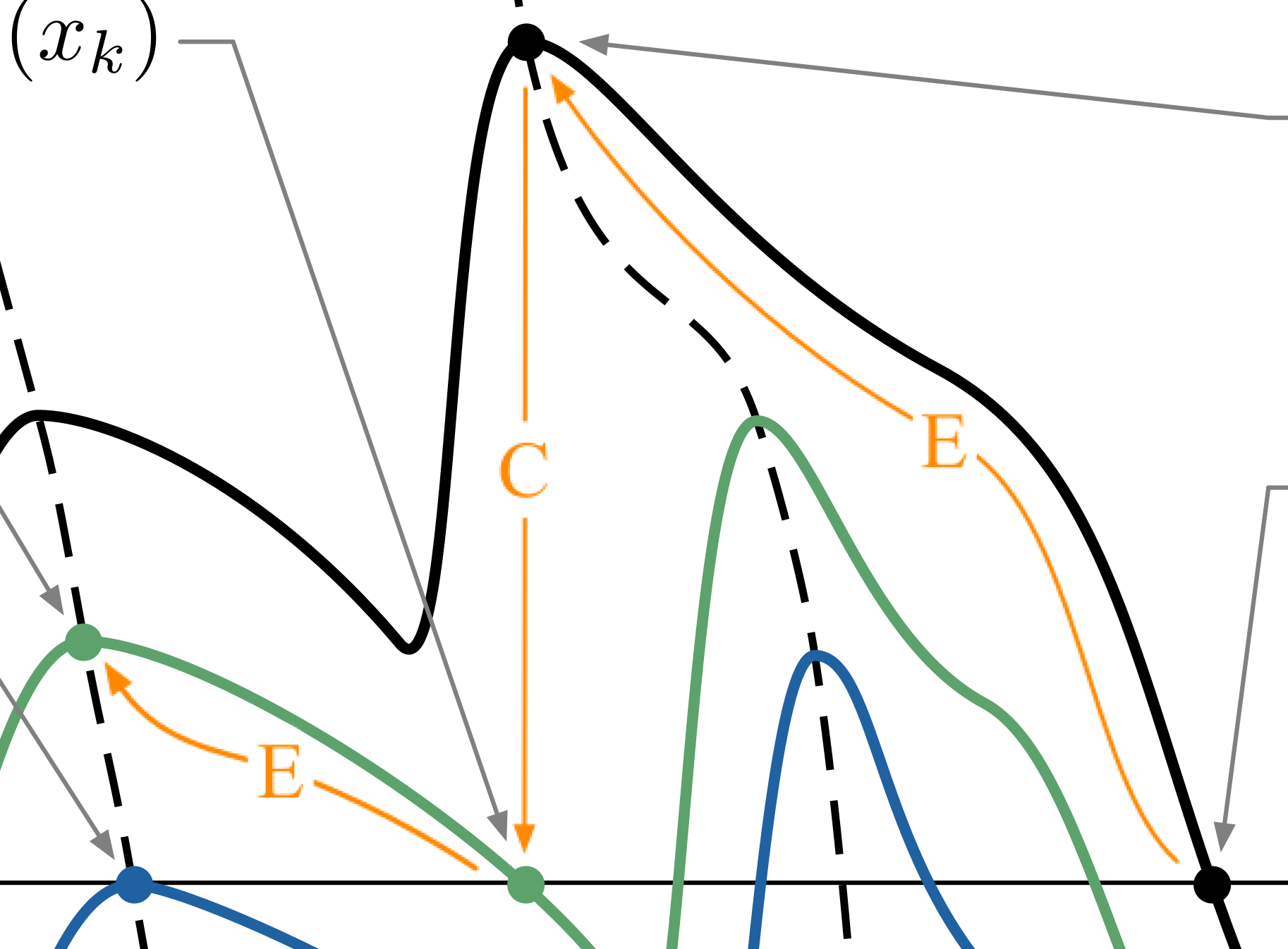
We present quadratically convergent algorithms to compute the extremal value of a real parameter for which a given rational transfer function of a linear time-invariant system is passive. This problem is formulated for both continuous-time and discrete-time systems and is linked to the problem of finding a realization of a rational transfer function such that its passivity radius is maximized. Our new methods make use of the Hybrid Expansion-Contraction algorithm, which we extend and generalize to the setting of what we call root-max problems.