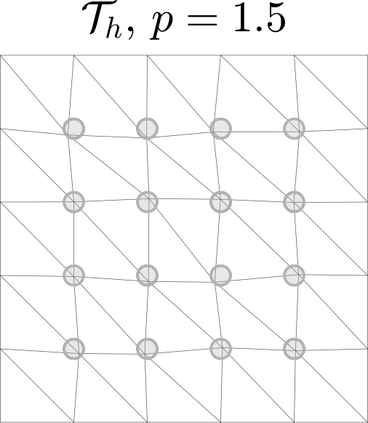
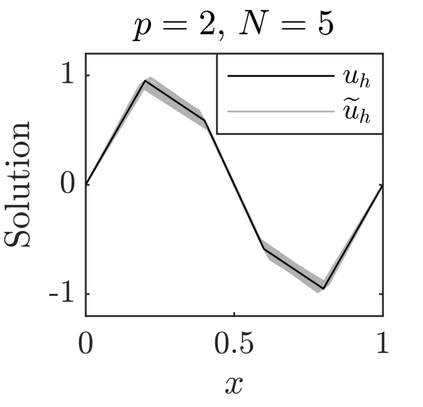
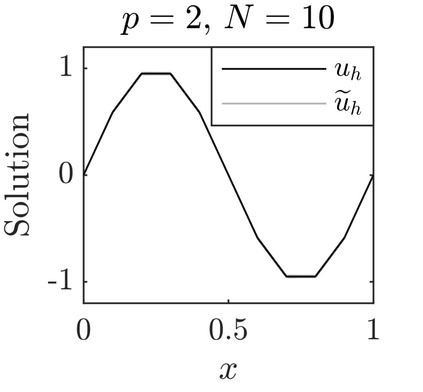
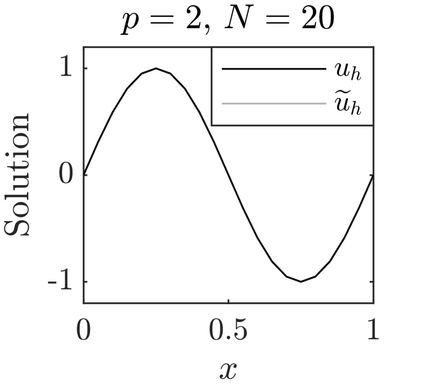
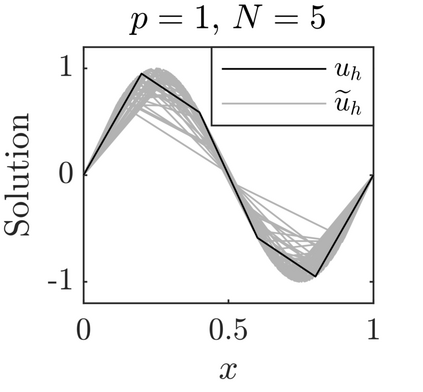
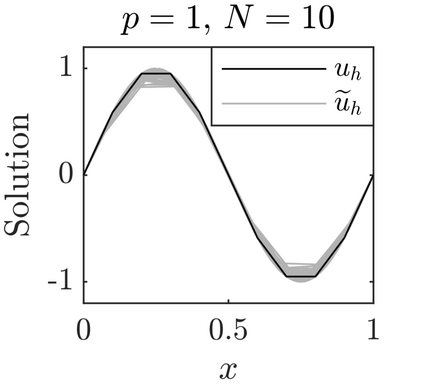
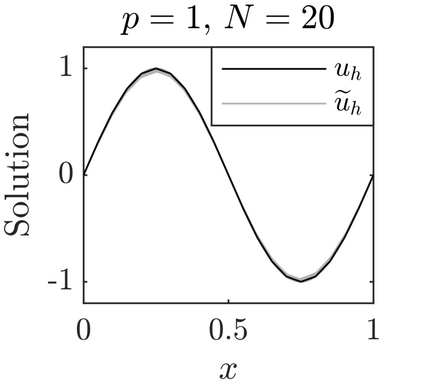
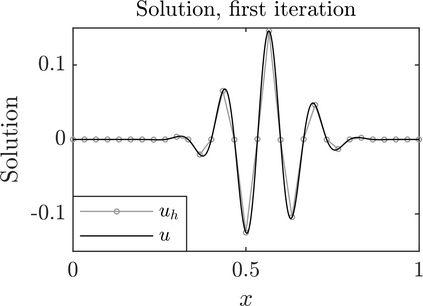
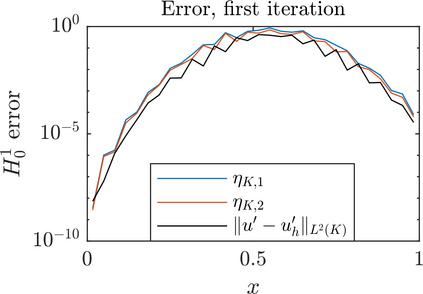
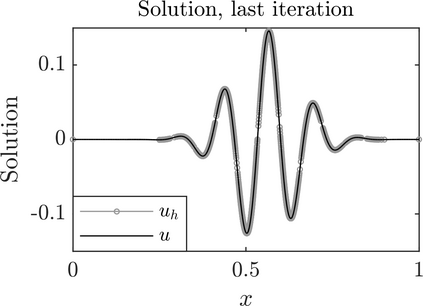
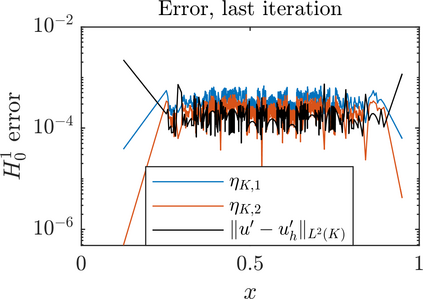
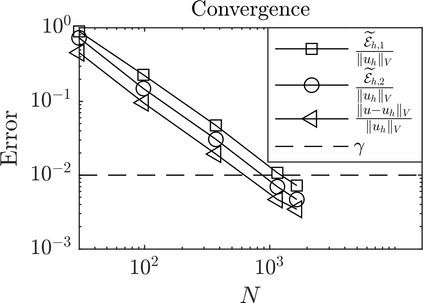
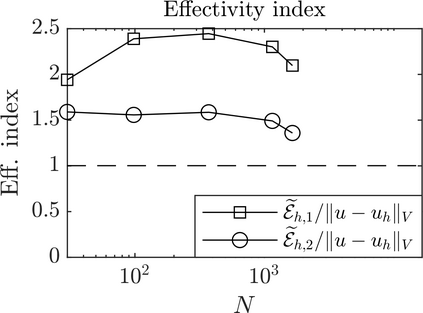
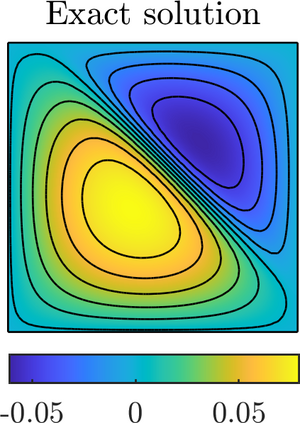
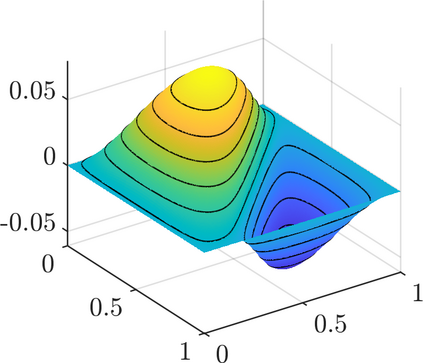
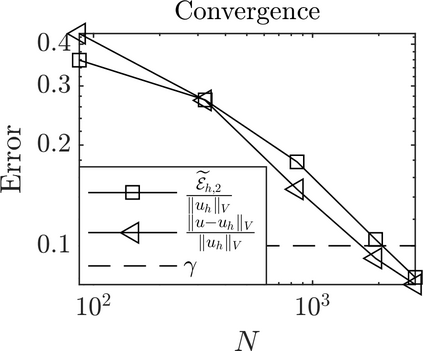
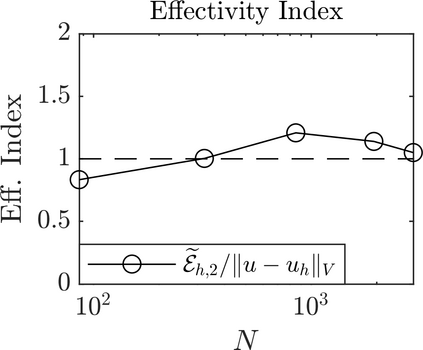
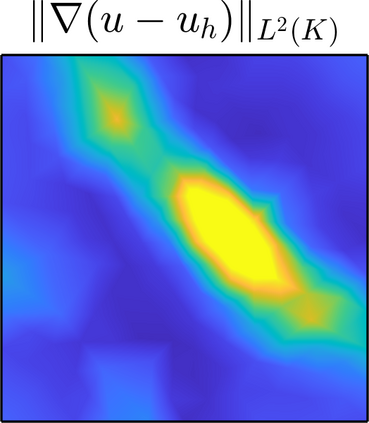
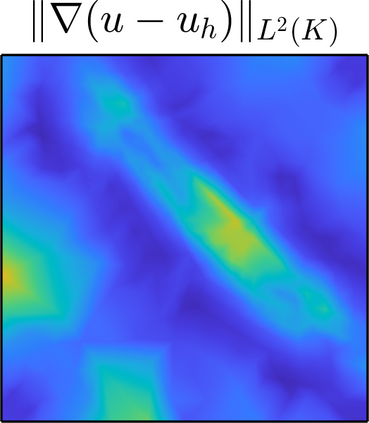
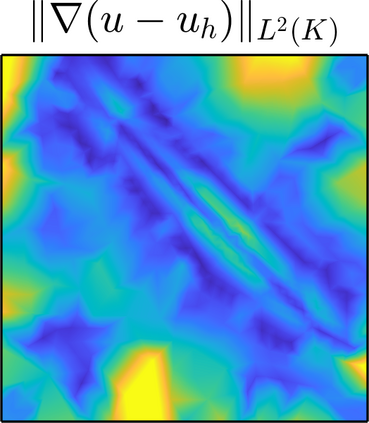
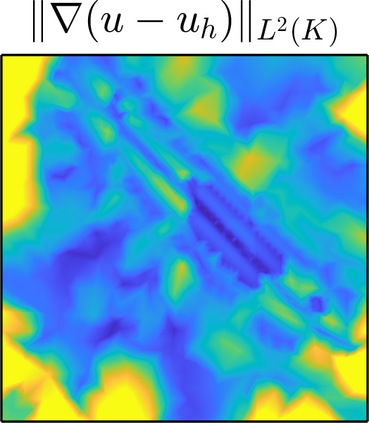
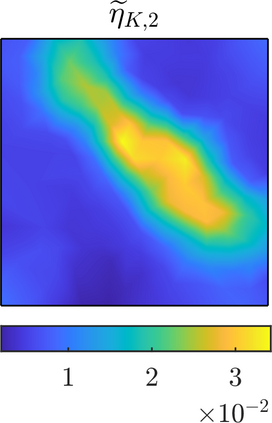
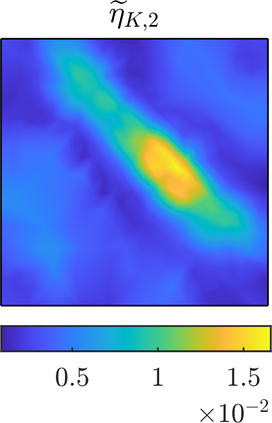
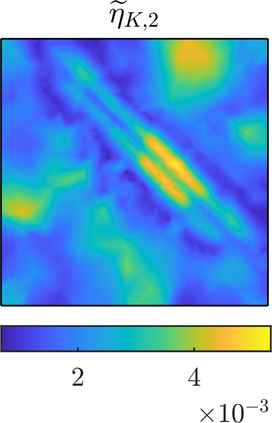
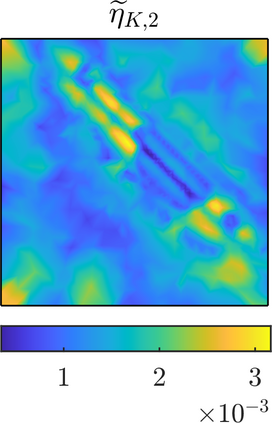
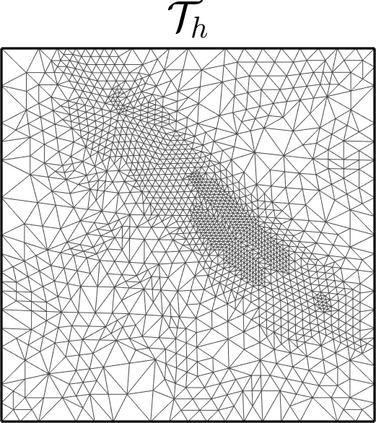
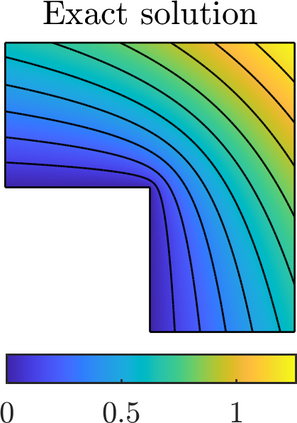
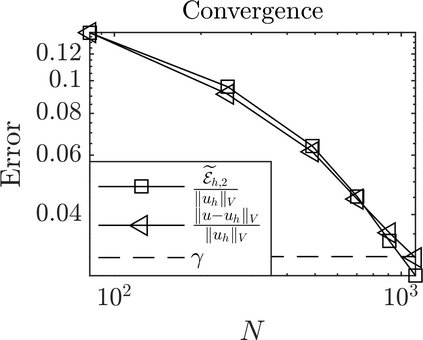
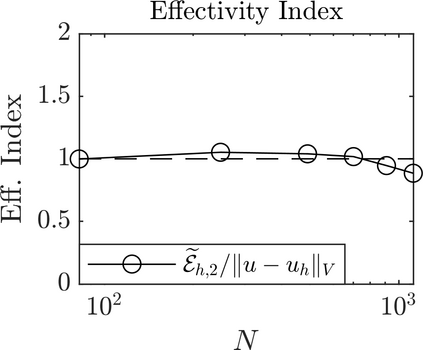
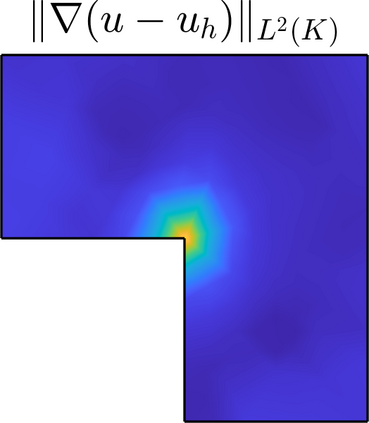
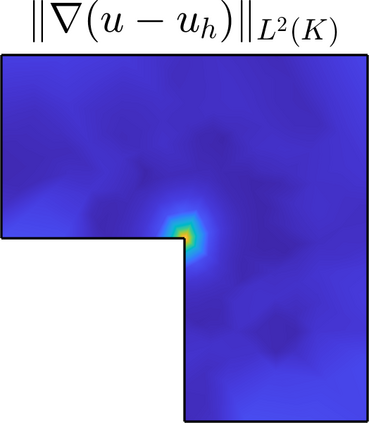
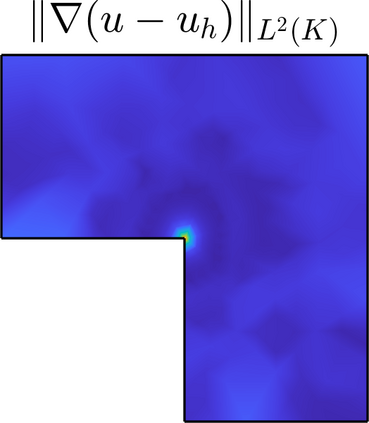
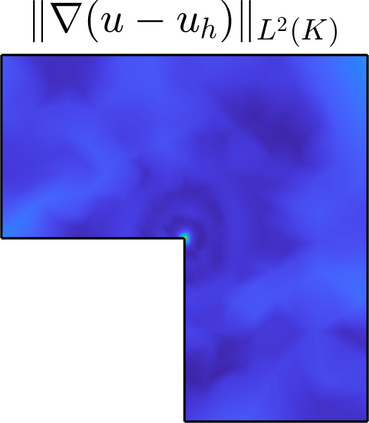
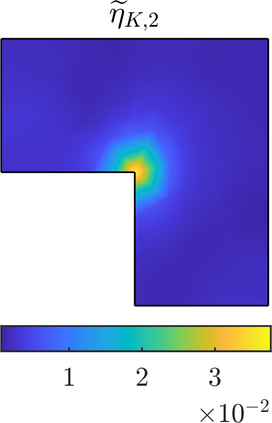
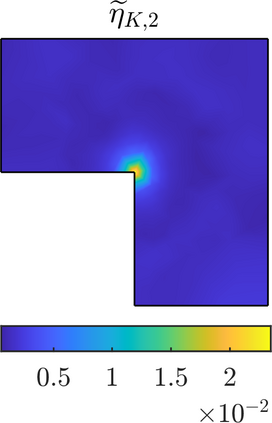
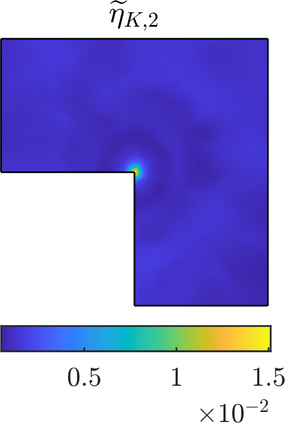
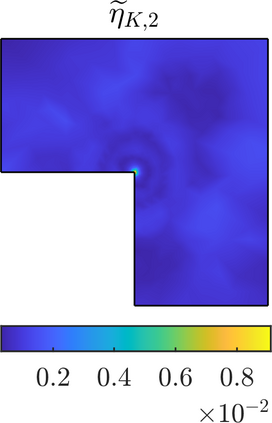
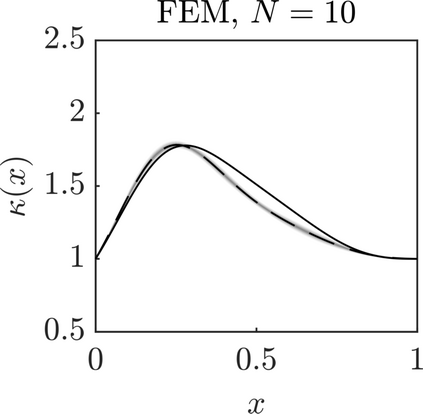
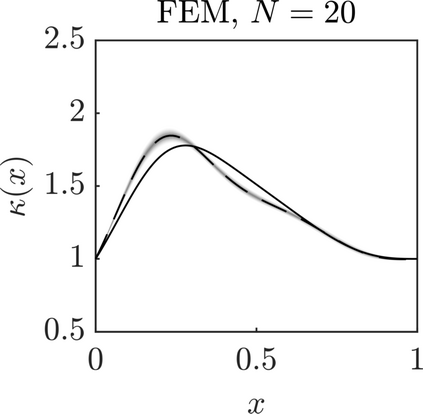
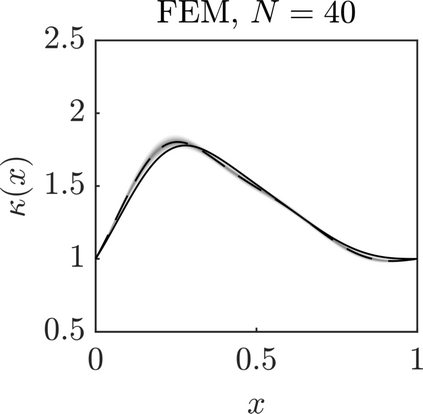
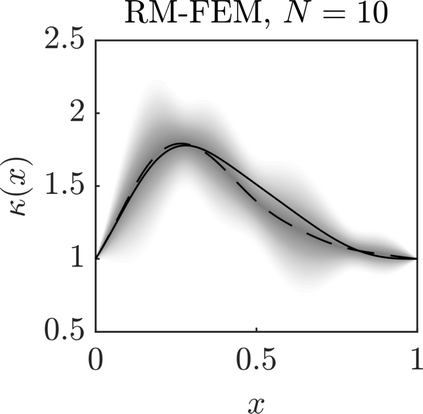
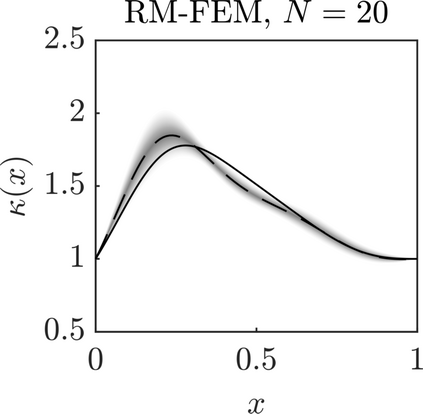
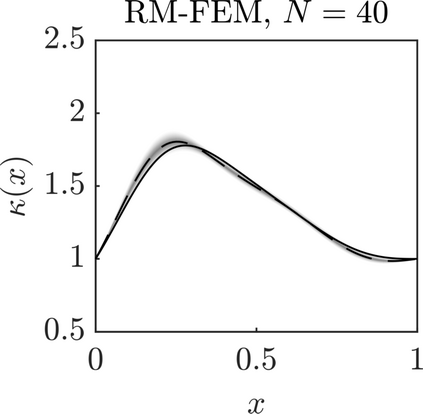
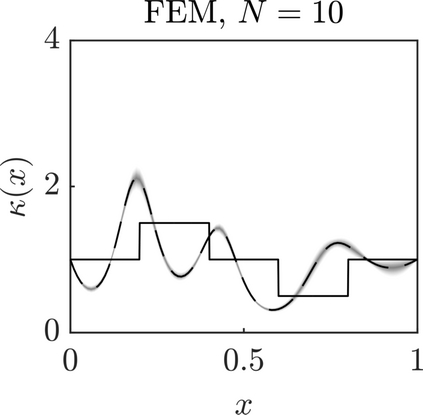
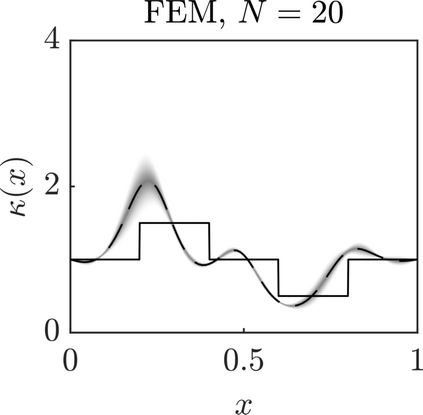
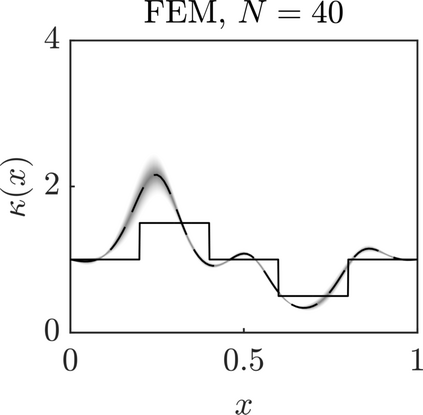
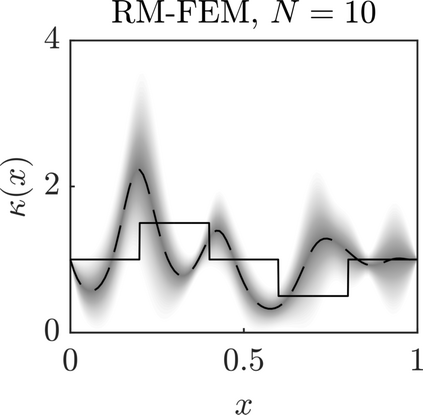
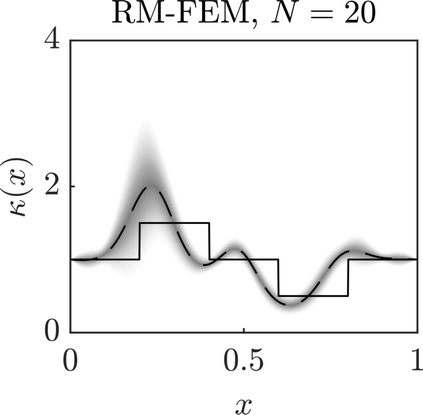
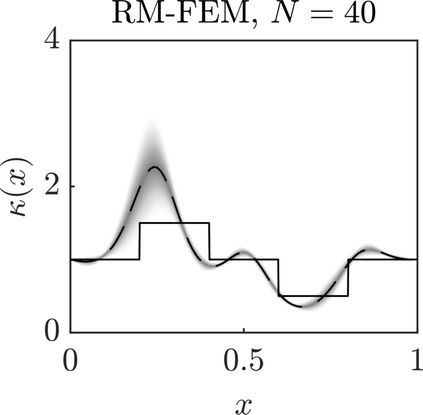
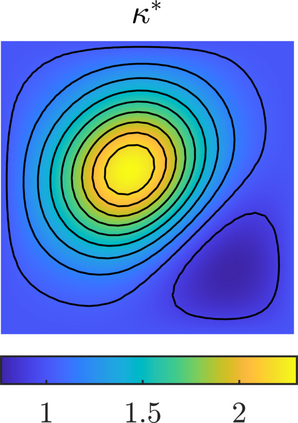
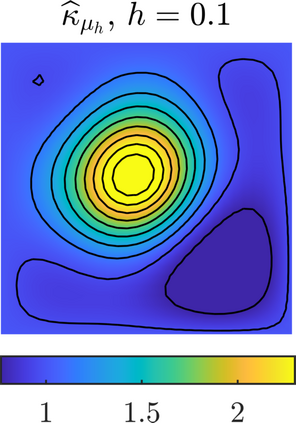
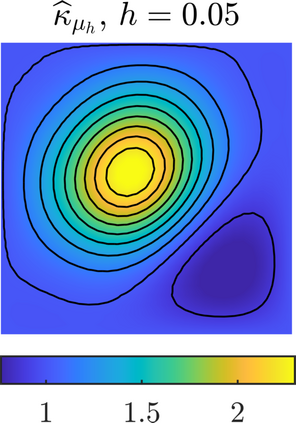
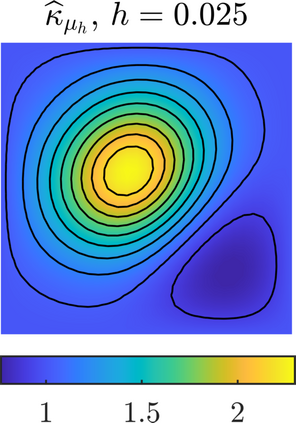
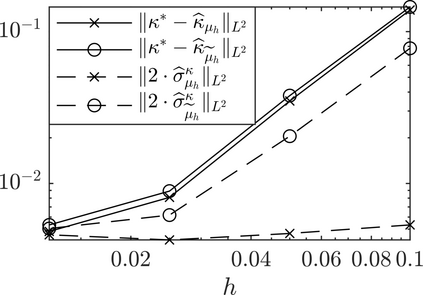
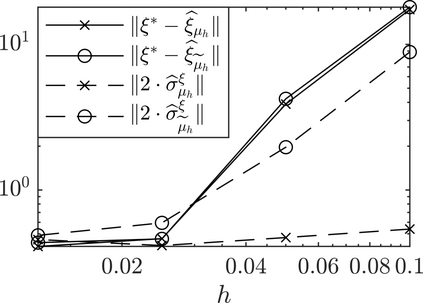
We present a novel probabilistic finite element method (FEM) for the solution and uncertainty quantification of elliptic partial differential equations based on random meshes, which we call random mesh FEM (RM-FEM). Our methodology allows to introduce a probability measure on standard piecewise linear FEM. We present a posteriori error estimators based uniquely on probabilistic information. A series of numerical experiments illustrates the potential of the RM-FEM for error estimation and validates our analysis. We furthermore demonstrate how employing the RM-FEM enhances the quality of the solution of Bayesian inverse problems, thus allowing a better quantification of numerical errors in pipelines of computations.
翻译:我们提出了一个新颖的概率有限要素法(FEM),用于解决和不确定地量化基于随机介质的椭圆部分差异方程,我们称之为随机网状FEM(RM-FEM),我们的方法允许对标准的单片线性FEM(RM-FEM)引入概率计量。我们提出了一个仅以概率信息为基础的事后误差估计器。一系列数字实验表明RM-FEM在估计误差方面的潜力,并验证我们的分析。我们进一步表明,使用RM-FEM如何提高巴伊西亚反面问题解决方案的质量,从而能够更好地量化计算管道中的数字错误。