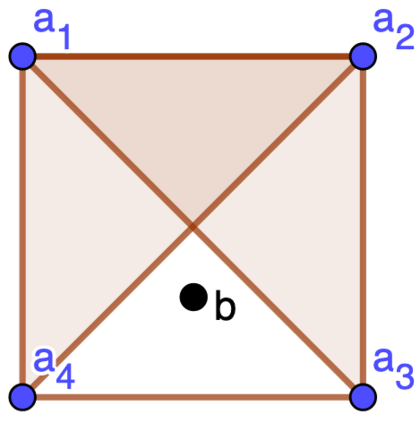
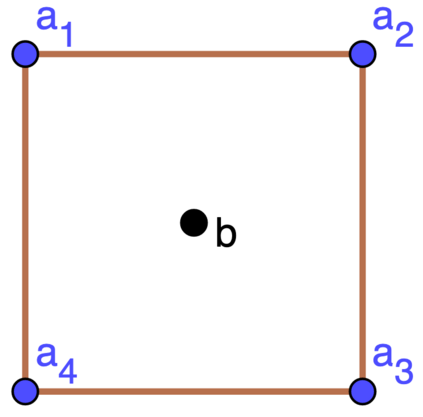
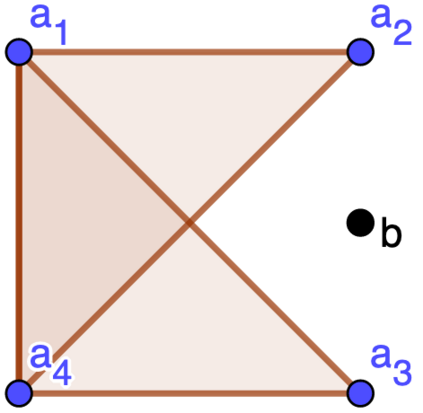
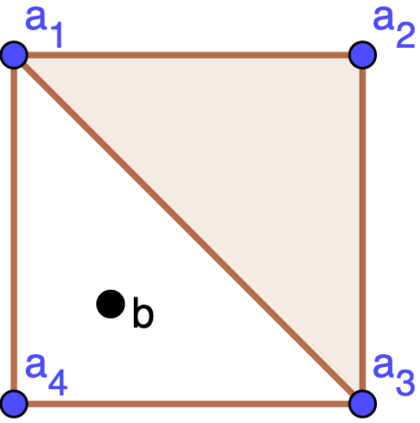
We establish connections between invariant theory and maximum likelihood estimation for discrete statistical models. We show that norm minimization over a torus orbit is equivalent to maximum likelihood estimation in log-linear models. We use notions of stability under a torus action to characterize the existence of the maximum likelihood estimate, and discuss connections to scaling algorithms.
翻译:我们建立了离散统计模型的不变理论和最大可能性估算之间的联系。 我们表明,对横滨轨道的常规最小化相当于日志线性模型中的最大可能性估算。 我们用横滨行动下的稳定概念来描述最大可能性估算的存在,并讨论与缩放算法的联系。
相关内容
专知会员服务
46+阅读 · 2020年2月23日
专知会员服务
41+阅读 · 2019年12月27日
Arxiv
0+阅读 · 2021年11月25日
Arxiv
0+阅读 · 2021年11月25日
Arxiv
0+阅读 · 2021年11月24日