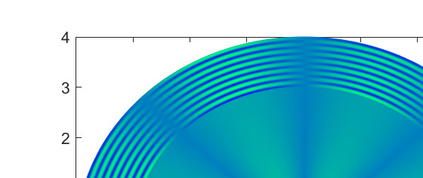
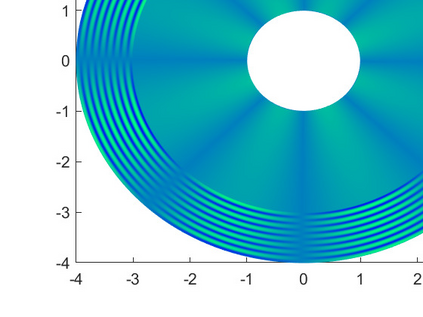
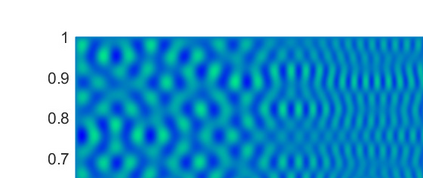
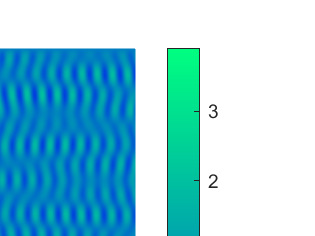
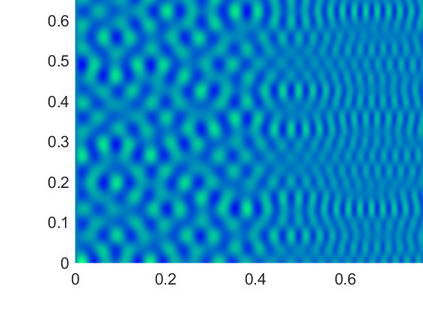
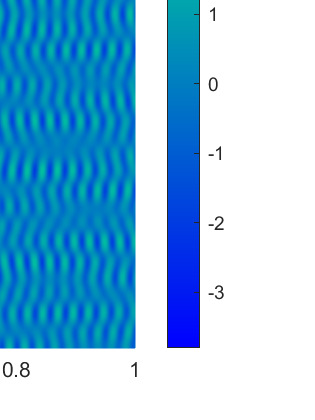
In this paper we introduce a new method called the Dirac Assisted Tree (DAT) method, which can handle 1D heterogeneous Helmholtz equations with arbitrarily large variable wave numbers. DAT breaks an original global problem into many parallel tree-structured small local problems, which are linked together to form a global solution by solving small linking problems. To solve the local problems in DAT, we propose a compact finite difference method (FDM) with arbitrarily high accuracy order and low numerical dispersion for piecewise smooth coefficients and variable wave numbers. This compact FDM is particularly appealing for DAT, because the local problems and their fluxes in DAT can be computed with high accuracy. DAT with such compact FDMs can solve heterogeneous Helmholtz equations with arbitrarily large variable wave numbers accurately by solving small linear systems - $4 \times 4$ matrices in the extreme case - with tridiagonal coefficient matrices in a parallel fashion. Several numerical examples are provided to illustrate the effectiveness of DAT using the $M$th order compact FDMs with $M=6,8$ for numerically solving heterogeneous Helmholtz equations with variable wave numbers. We shall also discuss how to solve some special 2D Helmholtz equations using DAT.
翻译:在本文中,我们引入了一种名为 Dirac 辅助树( DAT) 的新方法, 这个方法可以处理 1D 混杂的 Helmholtz 等式, 并任意使用大变化波数。 DAT 将最初的全球问题打破到许多平行的树结构小地方性问题, 这些问题通过解决小连接问题而联系在一起, 形成一个全球解决方案。 为了解决 DAT 的本地问题, 我们建议了一种简单精密的有限差异法( FMM ), 其精确度很高, 并且对于小相近的平滑系数和可变波数数字的差数分布性。 这个紧凑的 FDDM 特别吸引 DAT, 因为本地问题及其在 DAT 中的通量可以非常精确地计算。 DAT 与这种紧凑的 FDM 等式, 其本地问题及其通量可以以任意的大型波数解决 。 与小线性系统( 4 time 4 4 4 times 4$4$4$4$4$4$tgongon logal logs logs orgram orgram) 并同时使用三维系数矩阵矩阵矩阵矩阵矩阵矩阵矩阵。 。 。 提供几个数字解解解解解的FDM=6, 等式的FDM6, 解。