Piecewise polynomial regression of tame functions via integer programming

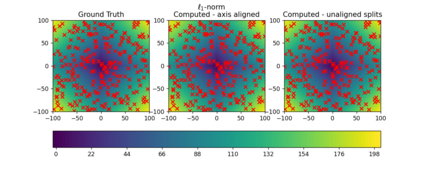
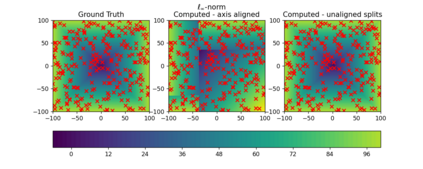
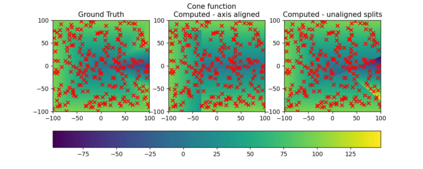
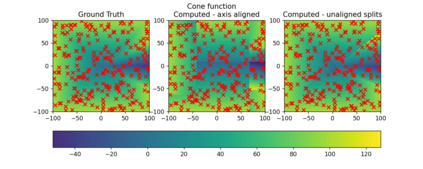
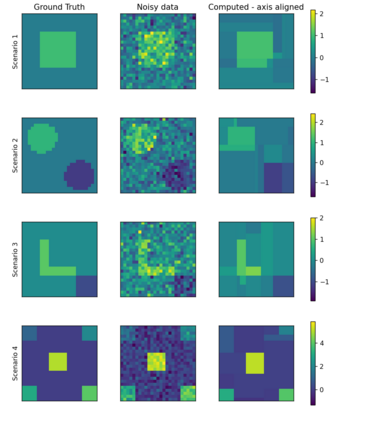
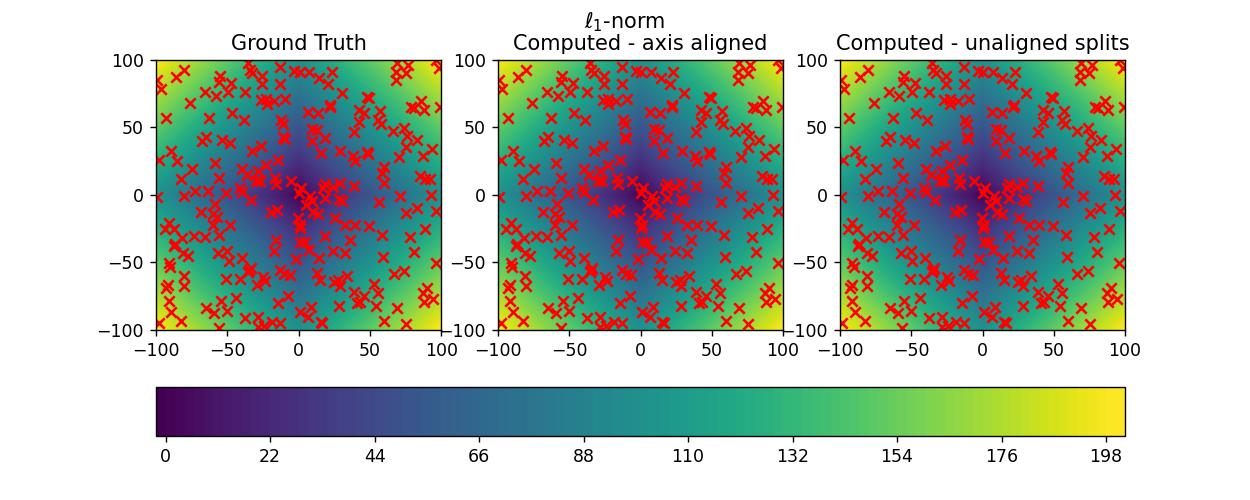
We consider the task of estimating functions belonging to a specific class of nonsmooth functions, namely so-called tame functions. These functions appear in a wide range of applications: training deep learning, value functions of mixed-integer programs, or wave functions of small molecules. We show that tame functions are approximable by piecewise polynomials on any full-dimensional cube. We then present the first ever mixed-integer programming formulation of piecewise polynomial regression. Together, these can be used to estimate tame functions. We demonstrate promising computational results.