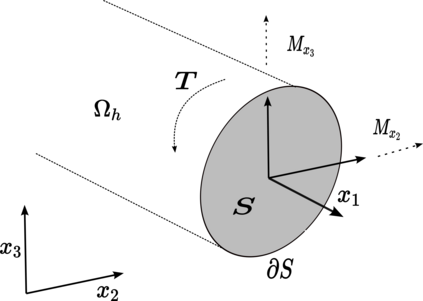
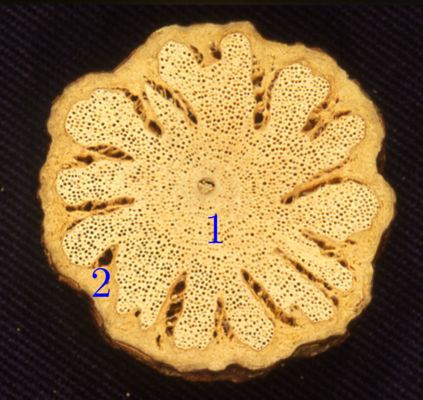
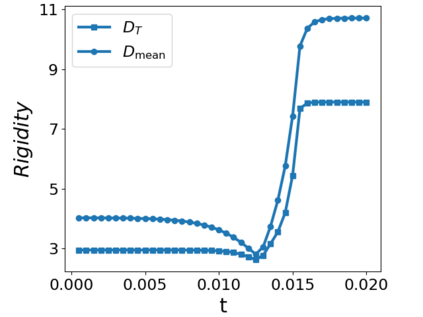
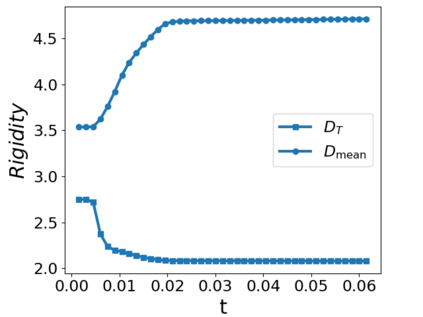
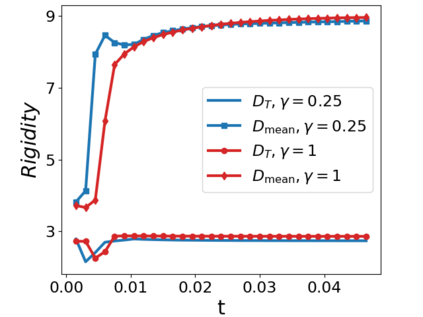
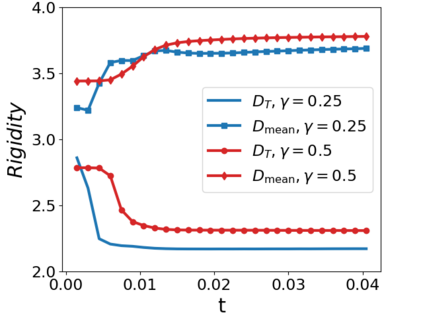
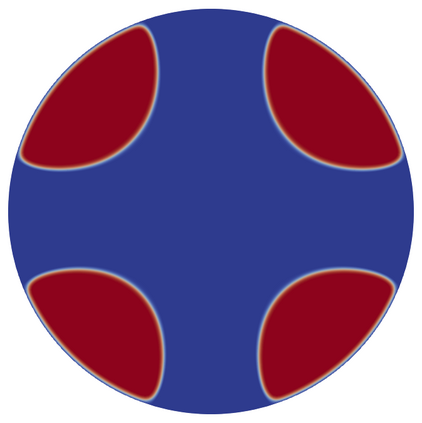
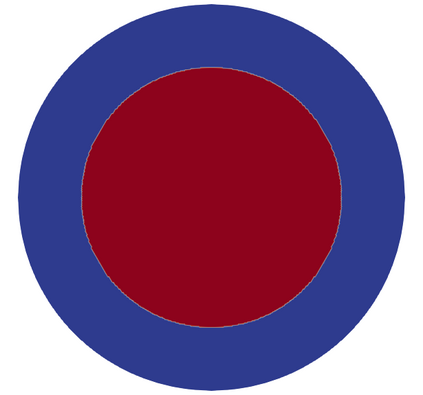
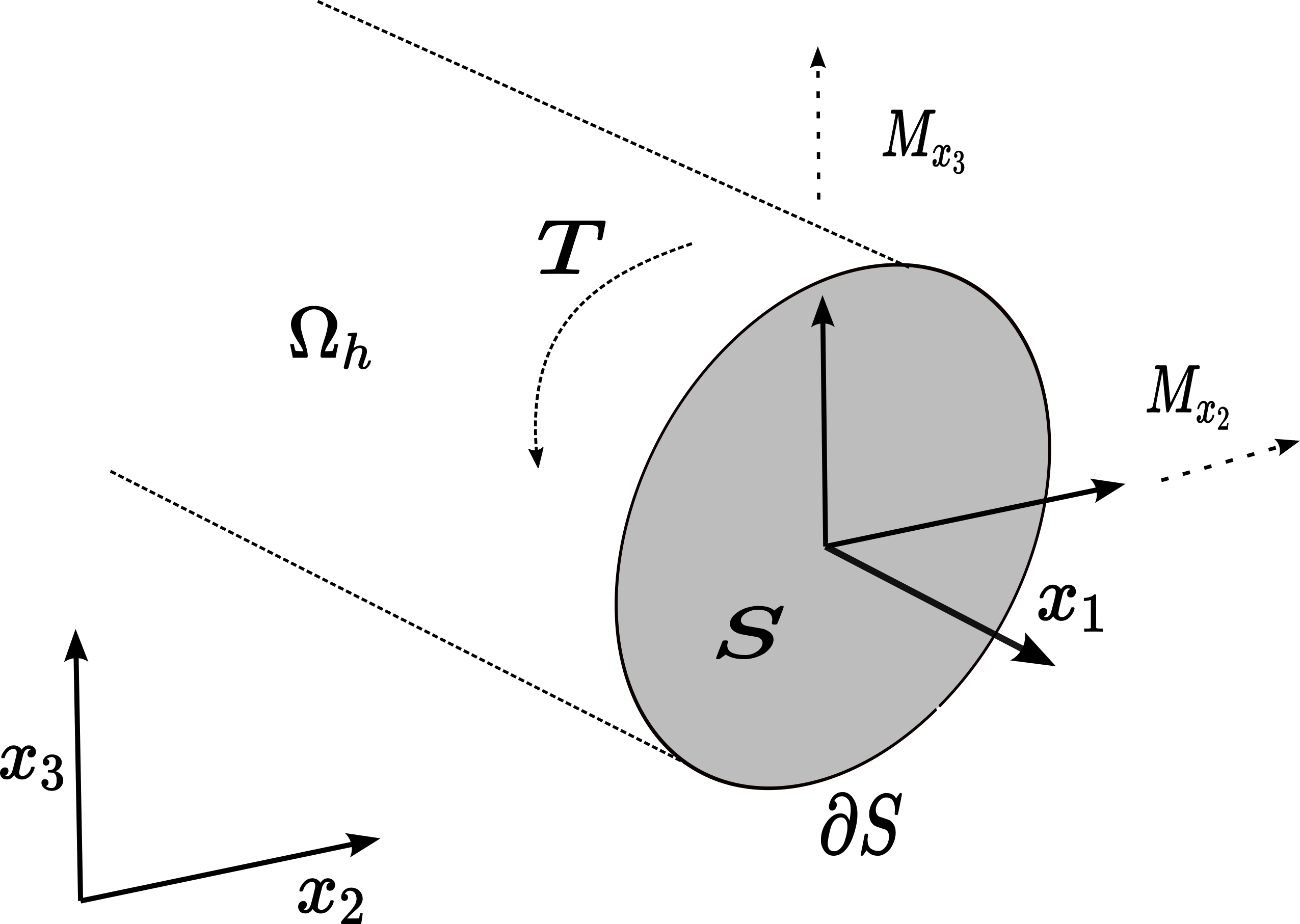
We derive a model for the optimization of the bending and torsional rigidities of non-homogeneous elastic rods. This is achieved by studying a sharp interface shape optimization problem with perimeter penalization, that treats both rigidities as objectives. We then formulate a phase field approximation of the optimization problem and show the convergence to the aforementioned sharp interface model via $\Gamma$-convergence. In the final part of this work we numerically approximate minimizers of the phase field problem by using a steepest descent approach and relate the resulting optimal shapes to the development of the morphology of plant stems.
翻译:暂无翻译
相关内容
专知会员服务
34+阅读 · 2020年1月15日
专知会员服务
36+阅读 · 2019年10月17日
Arxiv
0+阅读 · 2023年9月26日
Arxiv
0+阅读 · 2023年9月25日
Arxiv
0+阅读 · 2023年9月22日
Arxiv
0+阅读 · 2023年9月22日
Arxiv
0+阅读 · 2023年9月22日