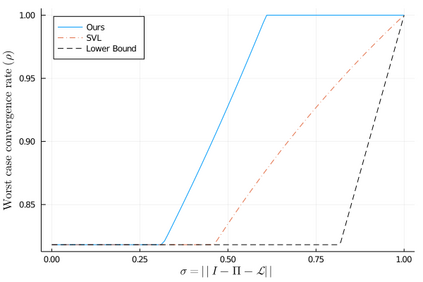
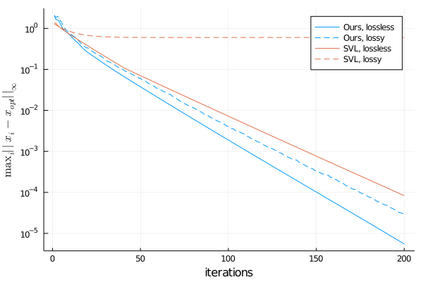
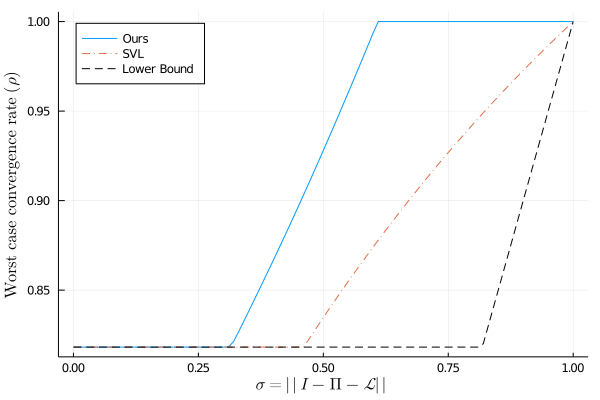
In this paper we describe a parameterized family of first-order distributed optimization algorithms that enable a network of agents to collaboratively calculate a decision variable that minimizes the sum of cost functions at each agent. These algorithms are self-healing in that their correctness is guaranteed even if they are initialized randomly, agents drop in or out of the network, local cost functions change, or communication packets are dropped. Our algorithms are the first single-Laplacian methods to exhibit all of these characteristics. We achieve self-healing by sacrificing internal stability, a fundamental trade-off for single-Laplacian methods.
翻译:在本文中,我们描述一阶分配优化算法的参数化组合,它使代理网络能够合作计算一个决定变量,最大限度地减少每个代理的成本功能的总和。这些算法是自我健康的,因为即使它们随机初始化,代理在网络中或网络外的下降,本地成本功能的变化,或通信包被丢弃,它们也能够保证其正确性。我们的算法是第一个展示所有这些特征的单拉拉西人方法。我们通过牺牲内部稳定实现自我平衡,这是单拉巴西人方法的基本权衡。