A note on graphs with purely imaginary per-spectrum

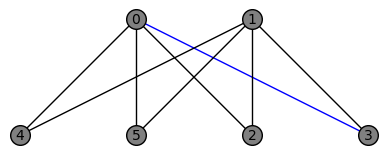
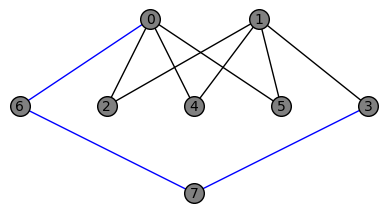
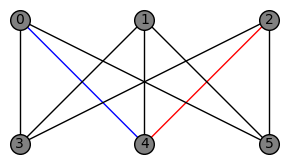
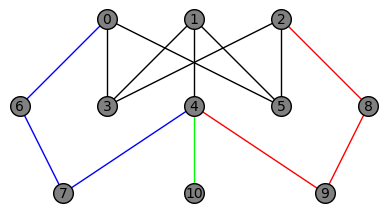
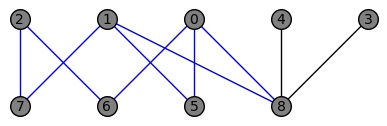
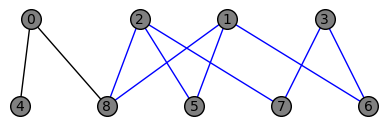
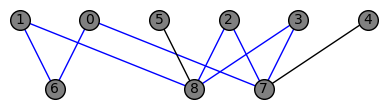
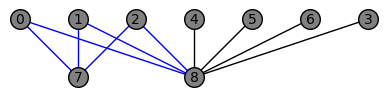
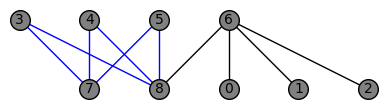
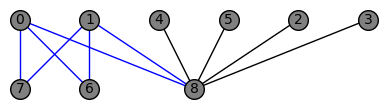
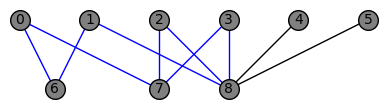
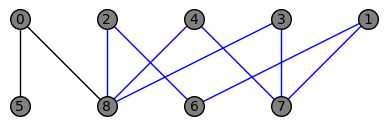
In 1983, Borowiecki and J\'o\'zwiak posed an open problem of characterizing graphs with purely imaginary per-spectrum. The most general result, although a partial solution, was given in 2004 by Yan and Zhang, who show that if a graph contains no subgraph which is an even subdivision of $K_{2,3}$, then it has purely imaginary per-spectrum. Zhang and Li in 2012 proved that such graphs are planar and admit a pfaffian orientation. In this article, we describe how to construct graphs with purely imaginary per-spectrum having a subgraph which is an even subdivision of $K_{2,3}$ (planar and nonplanar) using coalescence of rooted graphs.