Reply to Comment on "TVOR: Finding Discrete Total Variation Outliers among Histograms"

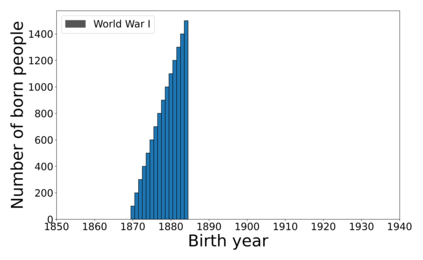
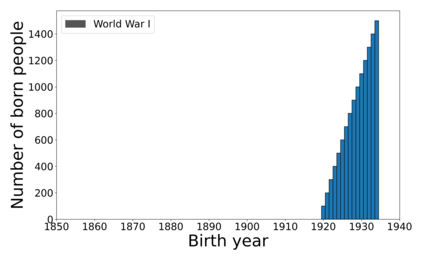
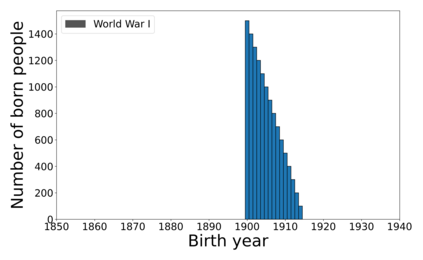
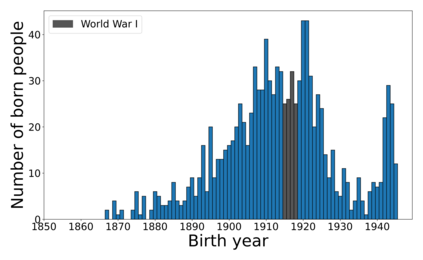
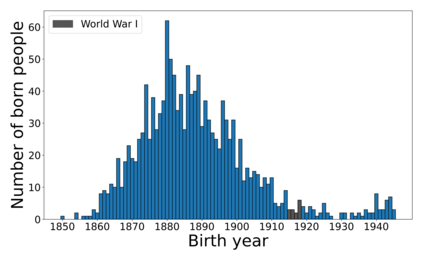
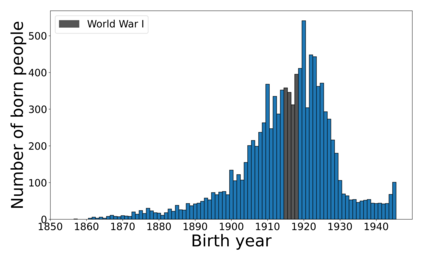
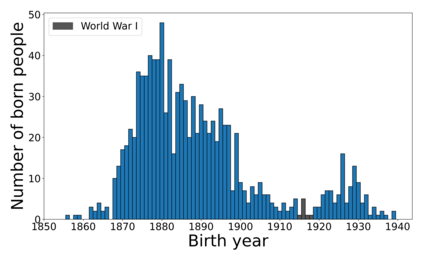
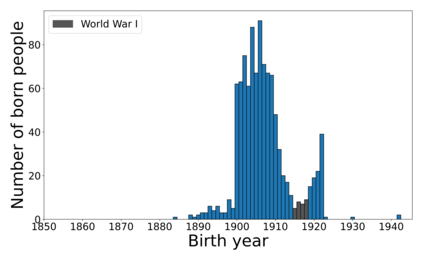
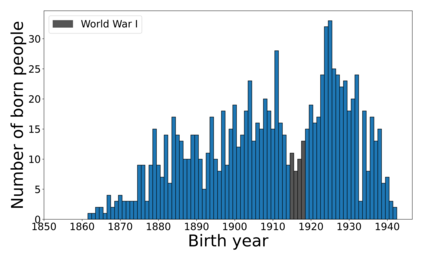
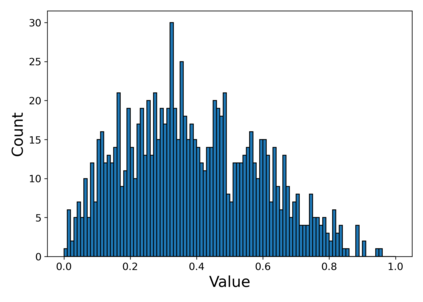
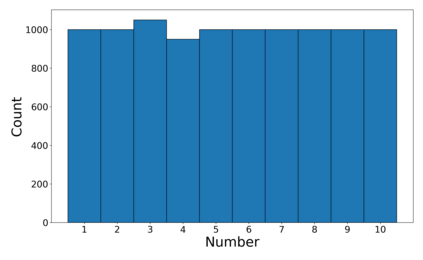
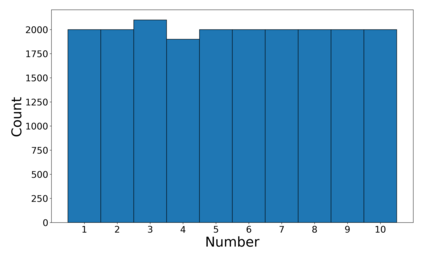
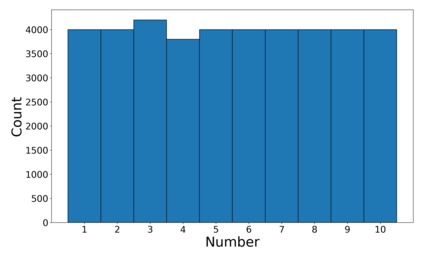
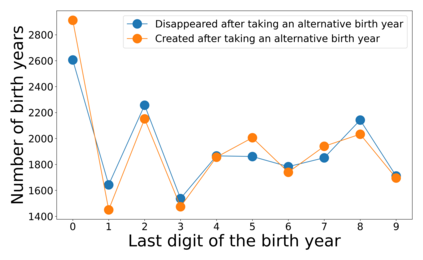
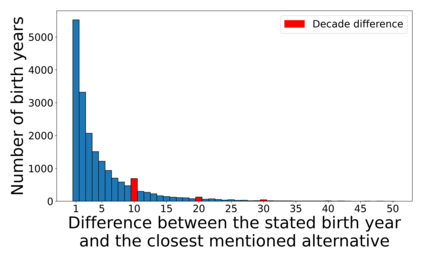
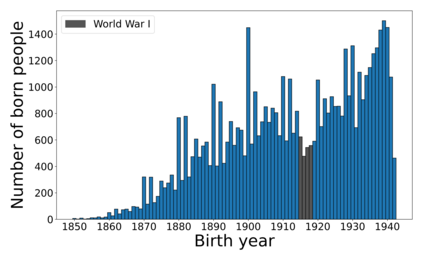
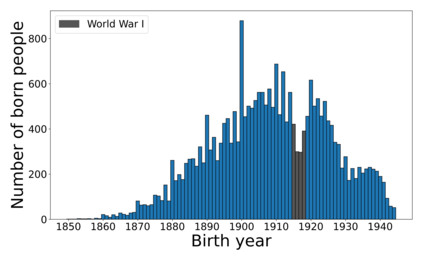
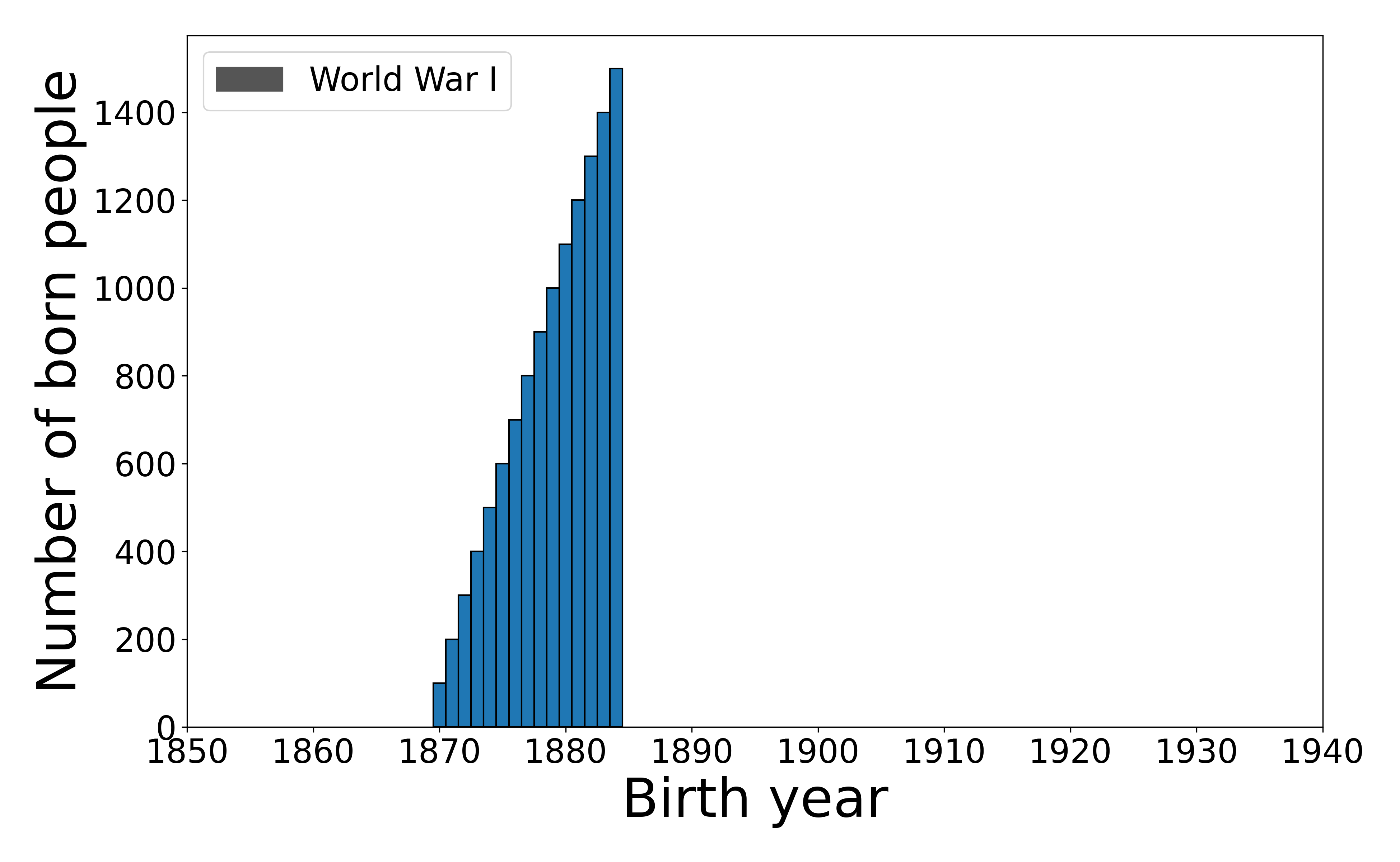
In this paper, we respond to a critique of one of our papers previously published in this journal, entitled "TVOR: Finding Discrete Total Variation Outliers among Histograms". Our paper proposes a method for smoothness outliers detection among histograms by using the relation between their discrete total variations (DTV) and their respective sample sizes. In this response, we demonstrate point by point that, contrary to its claims, the critique has not found any mistakes or problems in our paper, either in the used datasets, methodology, or in the obtained top outlier candidates. On the contrary, the critique's claims can easily be shown to be mathematically unfounded, to directly contradict the common statistical theorems, and to go against well established demographic terms. Exactly this is done in the reply here by providing both theoretical and experimental evidence. Additionally, due to critique's complaint, a more extensive research on top outlier candidate, i.e. the Jasenovac list is conducted and in order to clear any of the critique's doubts, new evidence of its problematic nature unseen in other lists are presented. This reply is accompanied by additional theoretical explanations, simulations, and experimental results that not only confirm the earlier findings, but also present new data. The source code is at https://github.com/DiscreteTotalVariation/TVOR.