List Decoding of 2-Interleaved Binary Alternant Codes

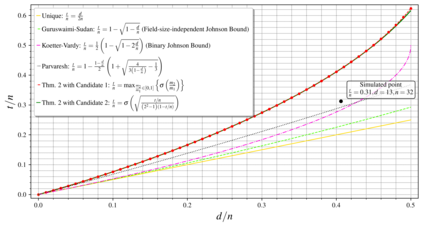
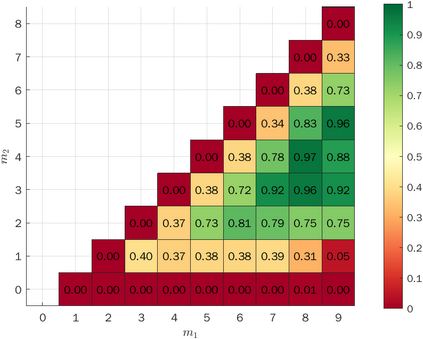
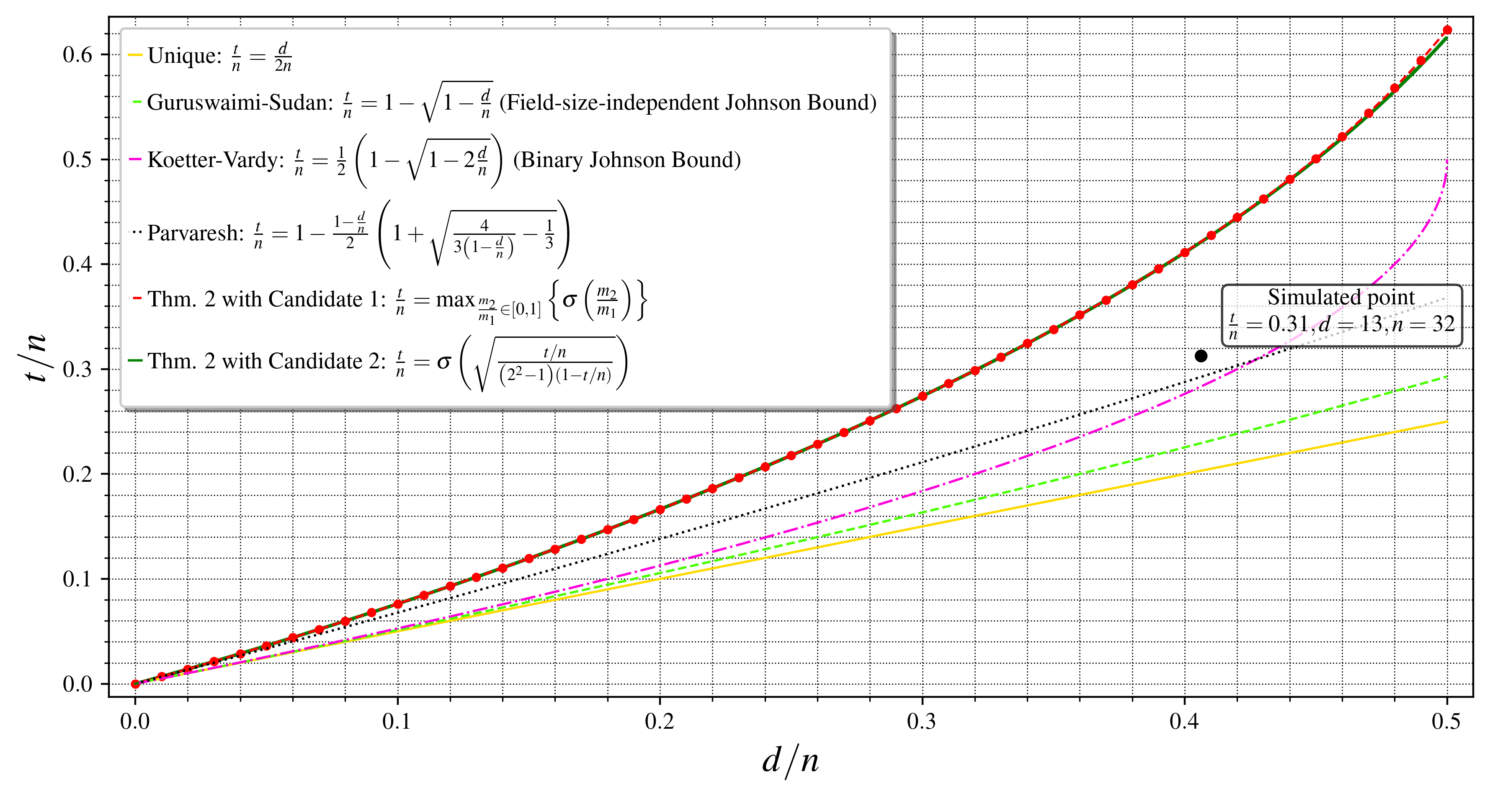
This paper is concerned with list decoding of $2$-interleaved binary alternant codes. The principle of the proposed algorithm is based on a combination of a list decoding algorithm for (interleaved) Reed-Solomon codes and an algorithm for (non-interleaved) alternant codes. The decoding radius exceeds the binary Johnson radius and the newly derived upper bound on the returned list size scales polynomially in the code parameters. Further, we provide simulation results on the probability of successful decoding by the proposed algorithm.