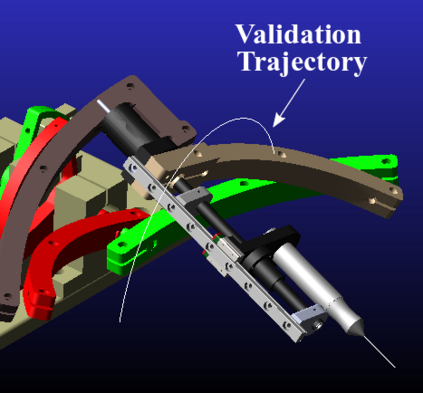
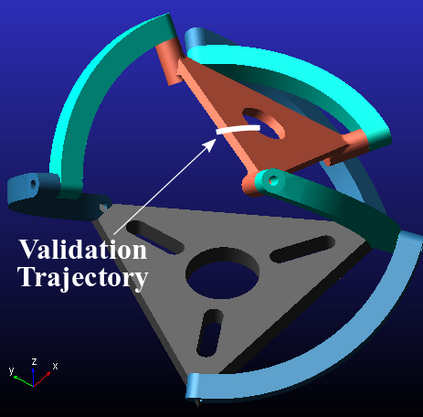
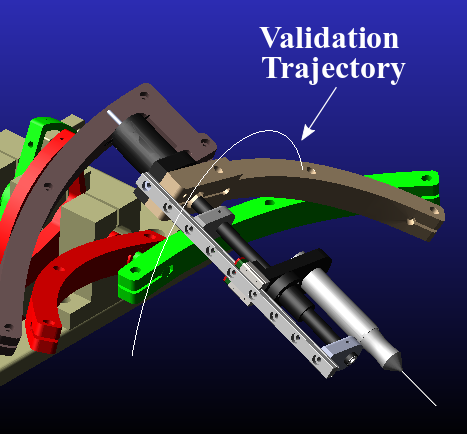
In this paper, derivation of different forms of dynamic formulation of spherical parallel robots (SPRs) is investigated. These formulations include the explicit dynamic forms, linear regressor, and Slotine-Li (SL) regressor, which are required for the design and implementation of the vast majority of model-based controllers and dynamic parameters identification schemes. To this end, the implicit dynamic of SPRs is first formulated using the principle of virtual work in task-space, and then by using an extension, their explicit dynamic formulation is derived. The dynamic equation is then analytically reformulated into linear and S-L regression form with respect to the inertial parameters, and by using the Gauss-Jordan procedure, it is reduced to a unique and closed-form structure. Finally, to illustrate the effectiveness of the proposed method, two different SPRs, namely, the ARAS-Diamond, and the 3-RRR, are examined as the case studies. The obtained results are verified by using the MSC-ADAMS software, and are shared to interested audience for public access.
翻译:本文调查了不同形式的球形平行机器人动态配制的衍生情况,这些配方包括明确的动态形式、线性递后器和Slotine-Li(SL)递后器,这是设计和实施绝大多数基于模型的控制器和动态参数识别办法所需要的。为此目的,SPR的隐含动态首先采用在任务空间进行虚拟工作的原则,然后通过使用扩展,得出其明确的动态配方。动态方程式随后根据惯性参数分析改制成线性形式和S-L回归形式,并且通过使用高斯-约旦程序,将之缩减为独特的封闭式结构。最后,为了说明拟议方法的有效性,两个不同的SPR,即ARAS-Diamond和3RRRR,作为案例研究加以审查。获得的结果通过使用MSC-ADMS软件加以核实,并提供给感兴趣的受众供公众查阅。