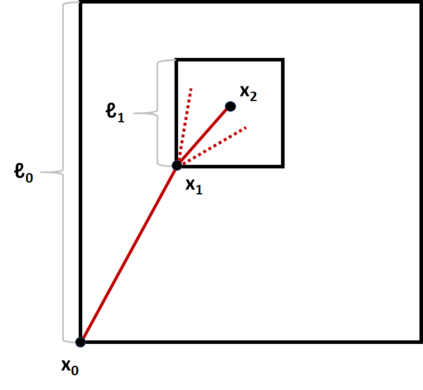
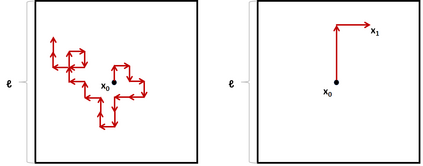
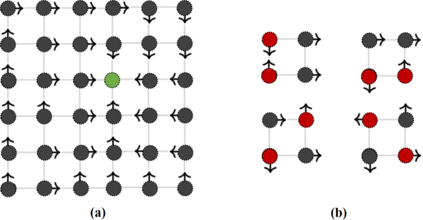
We consider the query complexity of finding a local minimum of a function defined on a graph, where at most $k$ rounds of interaction with the oracle are allowed. Rounds model parallel settings, where each query takes resources to complete and is executed on a separate processor. Thus the query complexity in $k$ rounds informs how many processors are needed to achieve a parallel time of $k$. We focus on the d-dimensional grid $[n]^d$, where the dimension $d$ is a constant, and consider two regimes for the number of rounds: constant and polynomial in n. We give algorithms and lower bounds that characterize the trade-off between the number of rounds of adaptivity and the query complexity of local search. When the number of rounds $k$ is constant, we show that the query complexity of local search in $k$ rounds is $\Theta\bigl(n^{\frac{d^{k+1} - d^k}{d^k - 1}}\bigl)$, for both deterministic and randomized algorithms. When the number of rounds is polynomial, i.e. $k = n^{\alpha}$ for $0 < \alpha < d/2$, the randomized query complexity is $\Theta\left(n^{d-1 - \frac{d-2}{d}\alpha}\right)$ for all $d \geq 5$. For $d=3$ and $d=4$, we show the same upper bound expression holds and give almost matching lower bounds. The local search analysis also enables us to characterize the query complexity of computing a Brouwer fixed point in rounds. Our proof technique for lower bounding the query complexity in rounds may be of independent interest as an alternative to the classical relational adversary method of Aaronson from the fully adaptive setting.
翻译:我们考虑在图形中找到一个本地最小值函数的查询复杂性, 该参数允许最多使用 $k$ 圆形平行设置。 圆形模型平行设置, 每个查询需要资源来完成, 并且由单独的处理器执行。 因此, $k$ 圆的查询复杂性可以告知需要多少个处理器来实现平行时间 $k$。 我们侧重于 d- 维格 $ 是常数的, 并考虑两个关于回合数数的系统 : 恒定 和 多元 。 我们给出了算法和下限 。 当回合数是 IMIL 、 MIQ_ 2 和 本地搜索的调序数 。 当回合数是 $_\ 美元 美元 时, 我们的本地搜索的查询复杂度是 美元 = d\ d\ d= dqd kd k - 1\\\ bigl), 用于确定和随机的算法。 当回合数是 MIL $. 美元 和 美元 美元 美元 美元 = 美元 美元 美元 美元 美元 美元 =\\\\\\\\\\\\\\\\\ 美元 美元 美元 计算法 美元 美元。 我们的计算法, 我们的本地的计算法, 我们的查询的查询法的查询的查询的查询的查询的查询的计算方法可能是的计算。