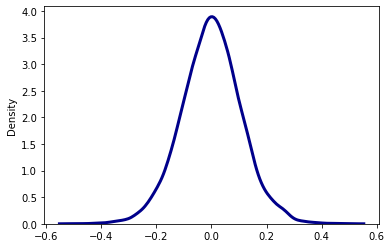
In this paper, we are testing the symmetry in the distribution of data observed on a random variable. We proposed test statistics using cumulative past and residual extropy of record values based on the characterization developed by Gupta and Chaudhary (2022) [5]. It is shown that the obtained estimator is consistent. Our proposed test has an advantage that we do not need to estimate the centre of symmetry. The empirical density, critical value and power of the proposed test statistics have been obtained. The test procedure has been implemented on six real-life data sets to verify its performance in identifying the symmetric nature. Simulations indicate our test performs better than the competitor tests.
翻译:在本文中,我们正在测试随机变量上观察到的数据分布的对称性,我们建议根据Gupta和Chaudhary(2022年)[5]的特征,使用记录值的累积过去和剩余极端体来计算累积记录值的测试统计数据,并表明获得的估算值是一致的。我们提议的测试的优点是,我们不需要估计对称中心。已经获得了拟议测试统计数据的经验密度、临界值和功率。测试程序已经对六个真实数据组进行,以核实其在确定对称性质方面的性能。模拟显示我们的测试表现优于竞争测试。