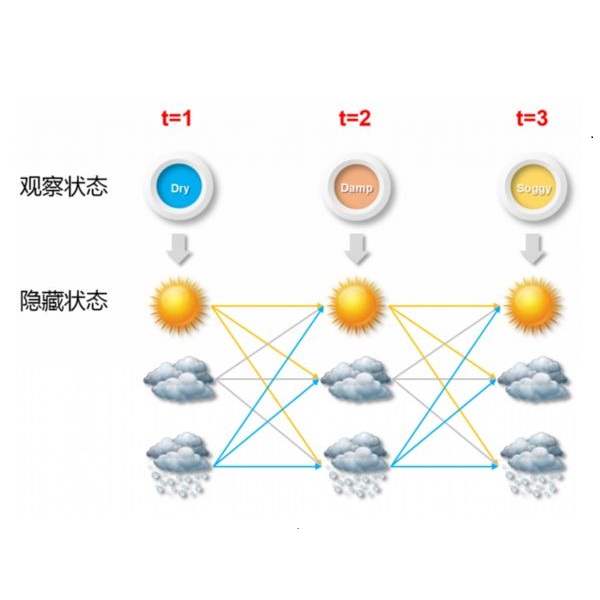
Finite state space hidden Markov models are flexible tools to model phenomena with complex time dependencies: any process distribution can be approximated by a hidden Markov model with enough hidden states.We consider the problem of estimating an unknown process distribution using nonparametric hidden Markov models in the misspecified setting, that is when the data-generating process may not be a hidden Markov model.We show that when the true distribution is exponentially mixing and satisfies a forgetting assumption, the maximum likelihood estimator recovers the best approximation of the true distribution. We prove a finite sample bound on the resulting error and show that it is optimal in the minimax sense--up to logarithmic factors--when the model is well specified.
翻译:最小状态隐藏的 Markov 模型是模拟具有复杂时间依赖性的现象的灵活工具: 任何过程分布都可以被隐藏的 Markov 模型和足够隐藏的状态相近。 我们考虑在错误指定的环境中使用非参数隐藏的 Markov 模型来估计未知的过程分布的问题, 也就是当数据生成过程可能不是隐藏的 Markov 模型的时候。 我们显示, 当真实分布是指数混合, 并且满足了遗忘的假设时, 最高可能性的天主将恢复真实分布的最佳近似值。 我们证明一个限定的样本, 被错误所包绑起来, 并显示在最小摩擦感到对数系数时, 它是最理想的。