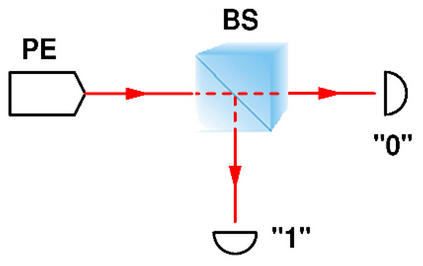
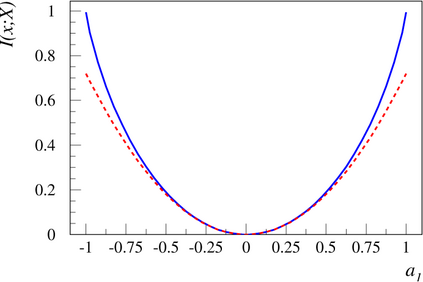
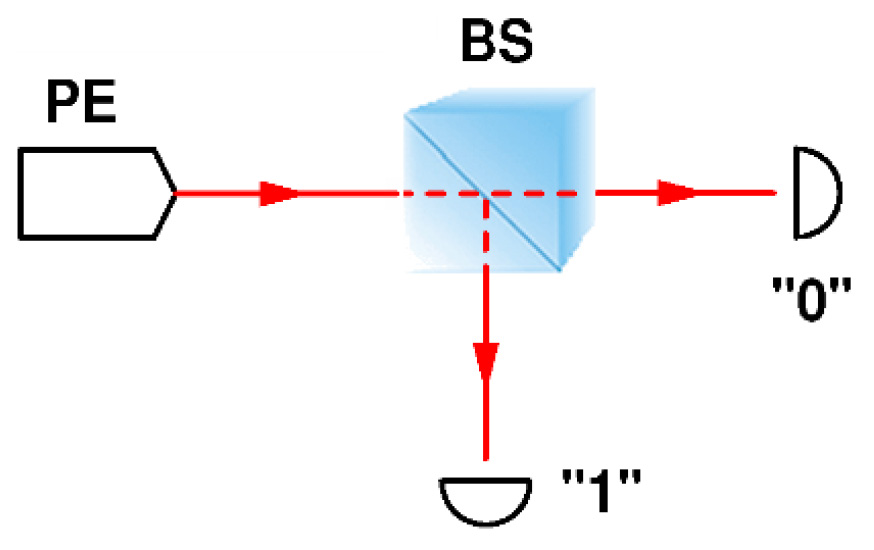
Ability to generate random numbers is an important resource for many applications ranging from scientific research to practical cryptography and quantum technologies. However, a widely accepted definition of random numbers, or randomness, has eluded researchers thus far. Without a definition, it is impossible to complete security proofs or make new industrial standards. Here, we propose an information-theory-based definition of randomness which, unlike state of the art, does not try to find desirable properties of generated numbers, but rather focus on the physical process of random number generation. We explain its intuitiveness, demonstrate its verifiability and further define randomness deviation as a measure of quality of the random number generating process or device.
翻译:生成随机数字的能力是许多应用的重要资源,从科学研究到实际加密和量子技术,但是,对于随机数字或随机性,迄今为止,一个广泛接受的定义一直受到研究人员的迷惑。没有定义,就不可能完成安全证明或制定新的工业标准。在这里,我们提出了一个基于信息、理论的随机性定义,该定义与最新技术不同,并不试图找到生成数字的可取属性,而是侧重于随机数字生成的物理过程。我们解释其直观性,展示其可核查性,并进一步界定随机偏差作为随机数字生成过程或装置质量的尺度。</s>