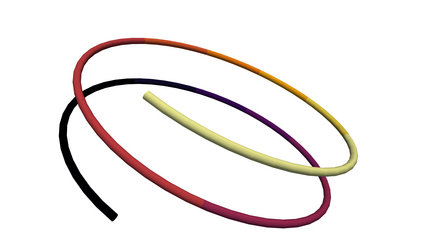
This paper presents a novel total Lagrangian cell-centred finite volume formulation of geometrically exact beams with arbitrary initial curvature undergoing large displacements and finite rotations. The choice of rotation parametrisation, the mathematical formulation of the beam kinematics, conjugate strain measures and the linearisation of the strong form of governing equations is described. The finite volume based discretisation of the computational domain and the governing equations for each computational volume are presented. The discretised integral form of the equilibrium equations are solved using a block-coupled Newton-Raphson solution procedure. The efficacy of the proposed methodology is presented by comparing the simulated numerical results with classic benchmark test cases available in the literature. The objectivity of strain measures for the current formulation and mesh convergence studies for both initially straight and curved beam configurations are also discussed.
翻译:本文介绍了一种新型的全局Lagrangian 以细胞为中心、 以细胞为核心、 以几何精确波束为单位的有限量配制, 其初始曲度在大规模迁移和有限旋转中具有任意性。 选择旋转平衡、 光束运动运动学的数学配方、 共振压力度量和强力方程式的线性化。 提出了计算域的有限量分解和每个计算体积的正方程。 平衡方程式的分解整体形式是用块组合式牛顿-拉夫森解决方案程序解决的。 拟议的方法的有效性是通过将模拟数字结果与文献中的典型基准测试案例进行比较来加以说明的。 还讨论了当前公式的强度计量的客观性,以及最初直线和弯曲波束组合的网形趋同研究的客观性。