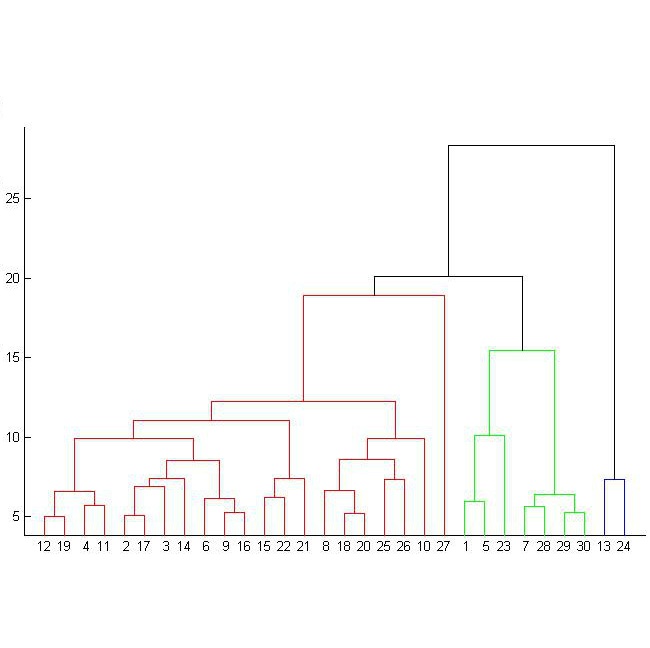
In this article, we define a new non-archimedean metric structure, called cophenetic metric, on persistent homology classes of all degrees. We then show that zeroth persistent homology together with the cophenetic metric and hierarchical clustering algorithms with a number of different metrics do deliver statistically verifiable commensurate topological information based on experimental results we obtained on different datasets. We also observe that the resulting clusters coming from cophenetic distance do shine in terms of different evaluation measures such as silhouette score and the Rand index. Moreover, since the cophenetic metric is defined for all homology degrees, one can now display the inter-relations of persistent homology classes in all degrees via rooted trees.
翻译:在本篇文章中,我们定义了一种新的非考古指标结构,称为同系物测量法,它针对不同程度的持久性同系物类别。然后我们表明,零持久性同系物加上带有若干不同指标的同系物指标和等级组合算法,确实根据我们从不同数据集获得的实验结果,提供了统计上可核实的相称的同系物学信息。我们还注意到,从同系物距离得出的组群,从诸如双影评分和Rand指数等不同评估措施来看,的确闪烁了光芒。此外,由于同系物指标是为所有同系物学学位确定的,因此现在人们可以通过根树显示不同程度的同系同系物类别之间的相互关系。