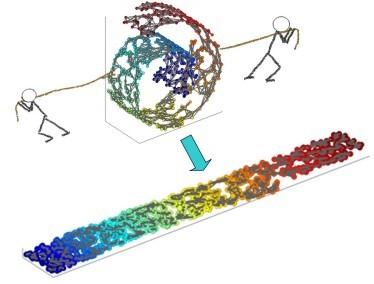
We demonstrate that locally linear embedding (LLE) inherently admits some unwanted results when no regularization is used, even for cases in which regularization is not supposed to be needed in the original algorithm. The existence of one special type of result, which we call ``projection pattern'', is mathematically proved in the situation that an exact local linear relation is achieved in each neighborhood of the data. These special patterns as well as some other bizarre results that may occur in more general situations are shown by numerical examples on the Swiss roll with a hole embedded in a high dimensional space. It is observed that all these bad results can be effectively prevented by using regularization.
翻译:我们证明,本地线性嵌入(LLE)在不使用正规化时,必然承认一些不想要的结果,即使是在原始算法中不应需要正规化的情况下也是如此。 一种特殊的结果(我们称之为“预测模式 ” ) 的存在从数学上证明,在数据每个邻近地区都实现了精确的本地线性关系。 这些特殊模式以及在更一般情况下可能发生的其他奇怪结果,在瑞士滚滚滚的数值示例中以高维空间中嵌入一个洞来说明。 人们注意到,使用正规化可以有效防止所有这些不良结果。