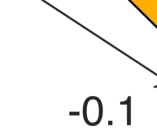
In this article, we focus on the error that is committed when computing the matrix logarithm using the Gauss--Legendre quadrature rules. These formulas can be interpreted as Pad\'e approximants of a suitable Gauss hypergeometric function. Empirical observation tells us that the convergence of these quadratures becomes slow when the matrix is not close to the identity matrix, thus suggesting the usage of an inverse scaling and squaring approach for obtaining a matrix with this property. The novelty of this work is the introduction of error estimates that can be used to select a priori both the number of Legendre points needed to obtain a given accuracy and the number of inverse scaling and squaring to be performed. We include some numerical experiments to show the reliability of the estimates introduced.
翻译:在本文中, 我们侧重于使用 Gaus- Legendre 等离值规则计算矩阵对数时发生的错误。 这些公式可以被解释为合适的高斯超几何函数的Pad\'e 近似值。 经验观测告诉我们, 当矩阵离身份矩阵不近时, 这些二次方位的趋同速度会缓慢, 从而表明使用反缩放和对齐方法获取此属性的矩阵。 这项工作的新颖之处是引入错误估计, 用于选择一个前置的图例点数量, 以获得一定的准确度, 以及要执行的反缩放和缩放数量。 我们包含一些数字实验, 以显示引入的估算的可靠性 。