Probabilistic Variational Causal Effect as A new Theory for Causal Reasoning

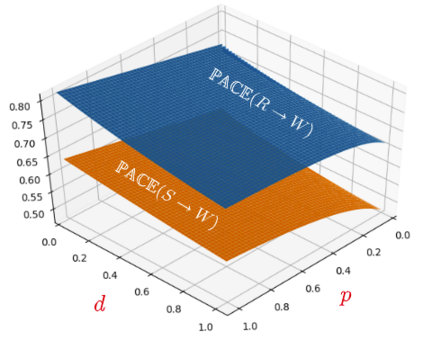
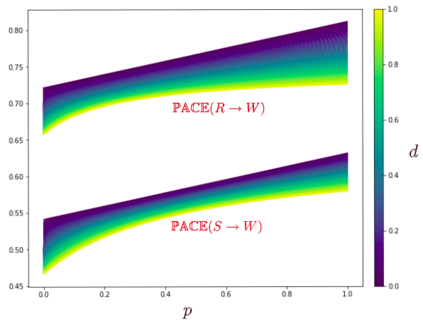
In this paper, we introduce a new causal framework capable of dealing with probabilistic and non-probabilistic problems. Indeed, we provide a formula called Probabilistic vAriational Causal Effect (PACE). Our formula of causal effect uses the idea of total variation of a function integrated with probability theory. PACE has a parameter $d$ determining the degree of being probabilistic. The lower values of $d$ refer to the scenarios that rare cases are important. In contrast, with the higher values of $d$, our model deals with the problems that are in nature probabilistic. Hence, instead of a single value for causal effect, we provide a causal effect vector by discretizing $d$. We also address the problem of computing counterfactuals in causal reasoning. We compare our model to the Pearl model, the mutual information model, the conditional mutual information model, and the Janzing et al. model by investigating several examples.