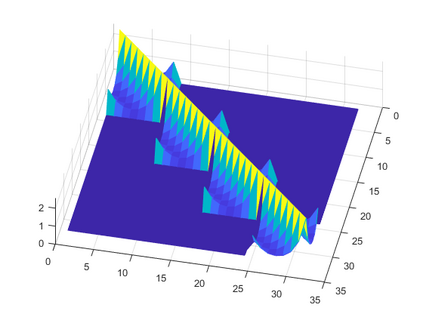
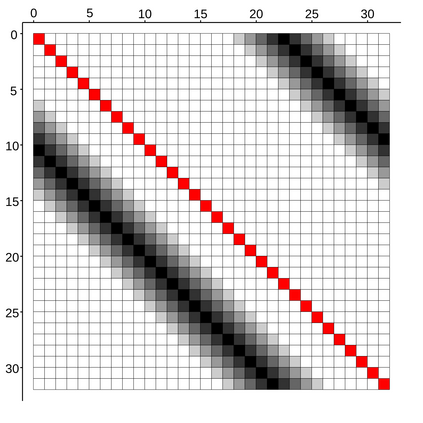
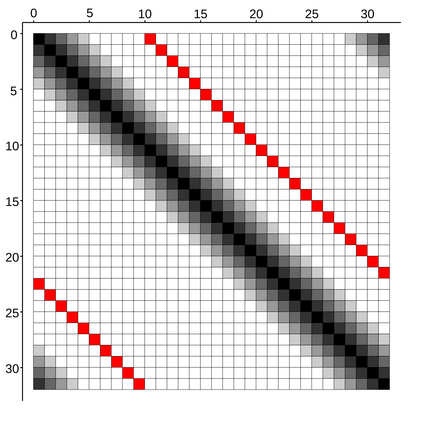
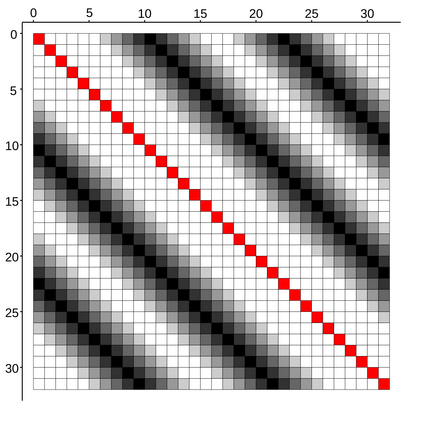
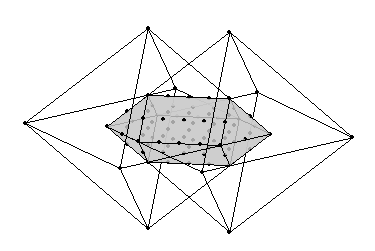
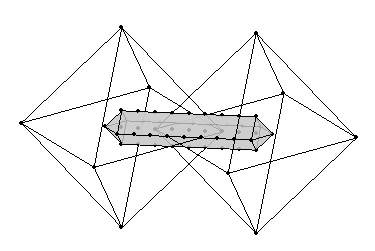
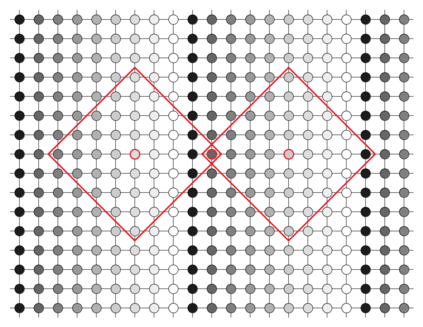
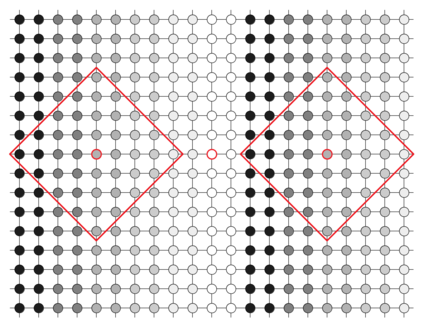
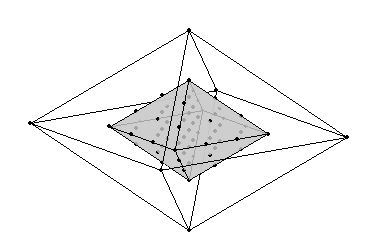
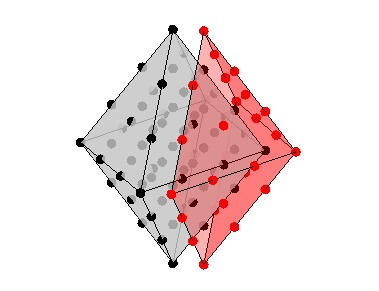
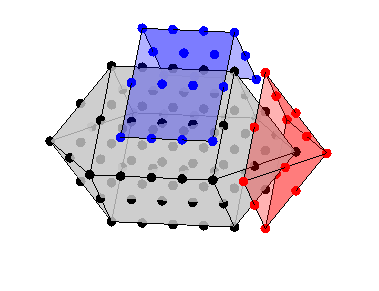
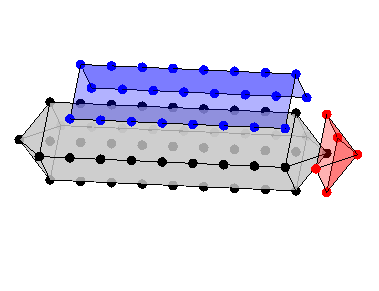
In this work, we study probing for the more general problem of computing the trace of a permutation of $A^{-1}$, say $PA^{-1}$. The motivation comes from Lattice QCD where we need to construct "disconnected diagrams" to extract flavor-separated Generalized Parton functions. In Lattice QCD, where the matrix has a 4D toroidal lattice structure, these non-local operators correspond to a $PA^{-1}$ where $P$ is the permutation relating to some displacement $\vec{p}$ in one or more dimensions. We focus on a single dimension displacement ($p$) but our methods are general. We show that probing on $A^k$ or $(PA)^k$ do not annihilate the largest magnitude elements. To resolve this issue, our displacement-based probing works on $PA^k$ using a new coloring scheme that works directly on appropriately displaced neighborhoods on the lattice. We prove lower bounds on the number of colors needed, and study the effect of this scheme on variance reduction, both theoretically and experimentally on a real-world Lattice QCD calculation. We achieve orders of magnitude speedup over the unprobed or the naively probed methods.
翻译:在这项工作中,我们研究如何调查计算美元-美元(即美元-美元-美元-美元-美元-美元-美元-美元-美元-美元-美元-美元-美元-美元-美元-美元-美元-美元-美元-美元-美元-美元-美元-美元-美元-美元-美元-美元-美元-美元-美元-美元-美元-美元-美元-美元-美元-美元-美元-美元-美元-美元-美元-美元-美元-美元-美元-美元-美元-美元-美元-美元-美元-美元-美元-美元-美元-美元-美元-美元-美元-美元-美元-美元-美元-美元-美元-美元-美元-美元-美元-美元-美元-美元-美元-美元-美元-美元-美元-美元-美元-美元-美元-美元-美元-美元-美元-美元-美元-美元-美元-美元-美元-美元-美元-美元-美元-美元-美元-美元-美元-美元-美元-美元-美元-美元-美元-美元-美元-美元-美元-美元-美元-美元-美元-美元-美元-美元-美元-美元-美元-美元-美元-美元-美元-美元-美元-美元-美元-美元-美元-美元-美元-美元-美元-美元-美元-美元-美元-美元-美元-美元-美元/美元-美元-美元-美元-美元-美元-美元-美元-美元-美元-美元-美元-美元-美元-美元-美元-美元-美元-美元-美元-美元-美元-美元-美元-美元-美元-美元-美元-美元-美元-美元-美元-美元-美元-美元-美元-美元-美元-美元-美元-美元-美元-美元-美元-美元-美元-美元-美元-美元-美元-美元-美元-美元-美元-美元-美元-美元-美元-美元-美元-美元-美元-美元-美元-美元-美元-美元-美元-美元-美元-美元-美元-美元-美元-美元-美元-美元-美元-美元-美元-美元-美元-美元-美元-美元-美元-美元-美元-美元-美元-美元-美元-美元-美元-美元-美元-美元-美元-美元-美元-美元-美元-美元-美元-美元-