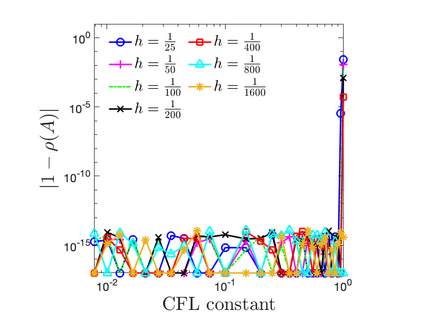
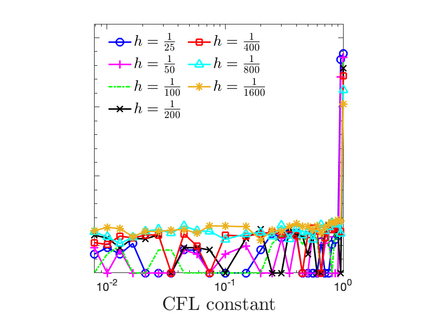
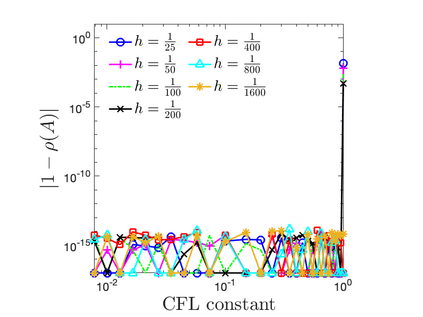
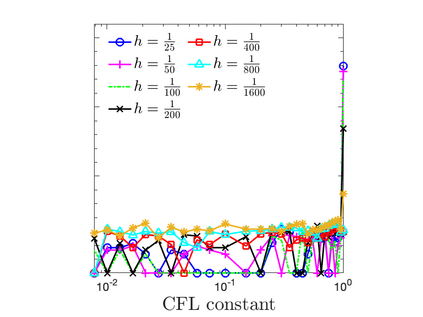
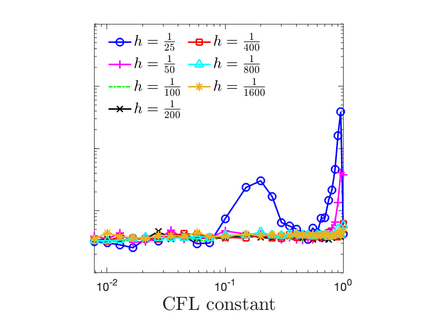
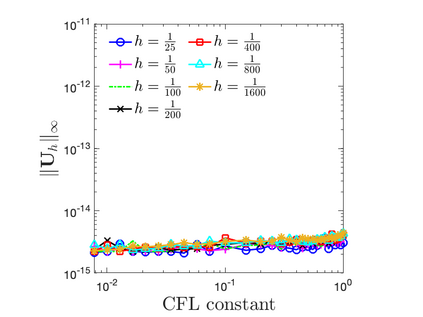
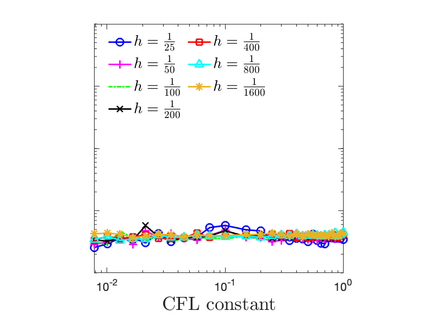
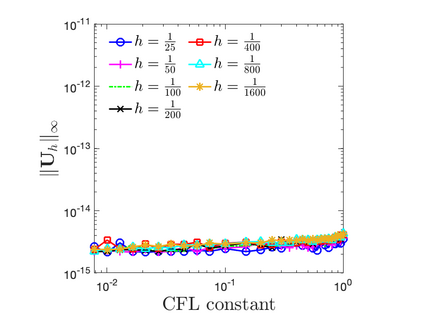
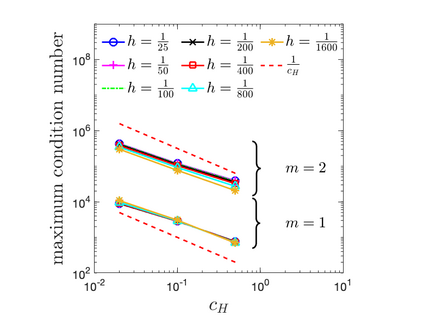
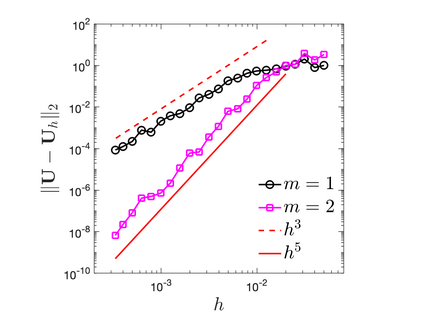
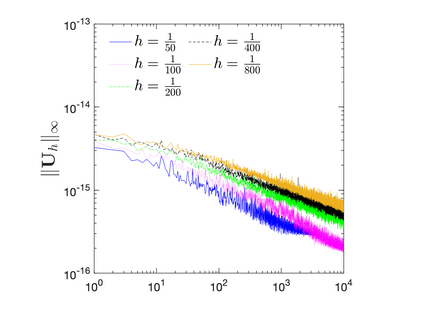
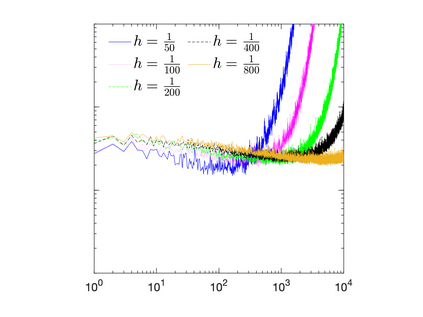
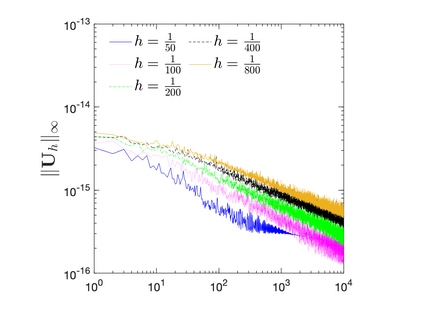
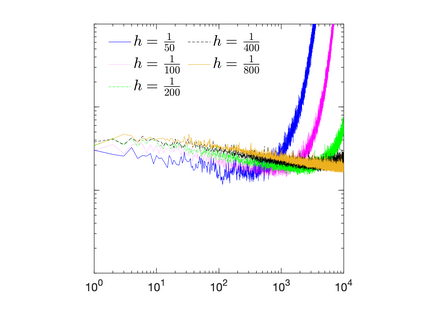
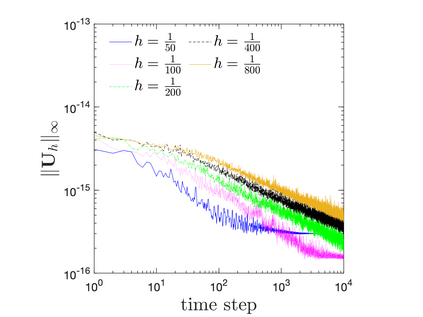
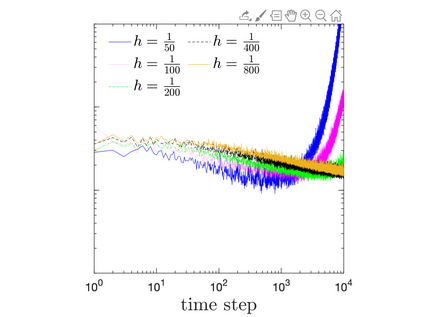
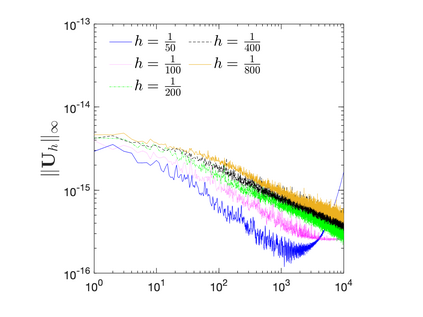
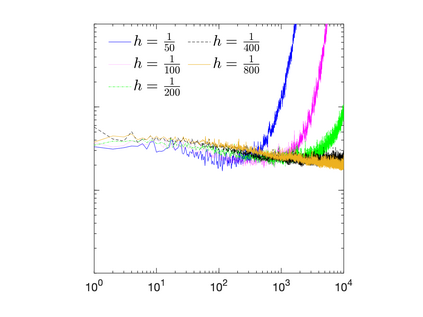
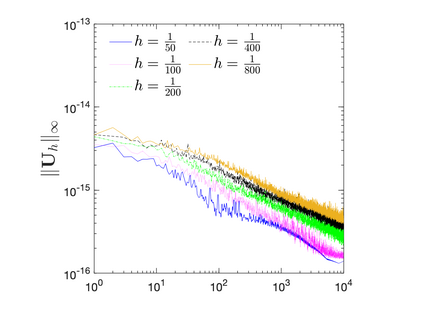
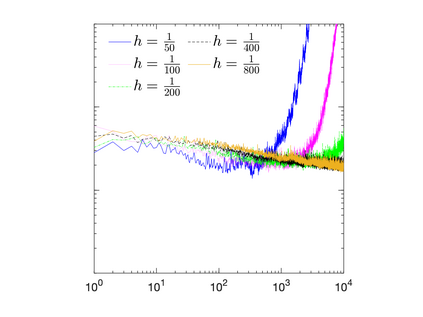
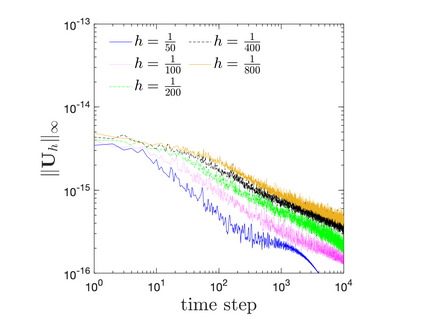
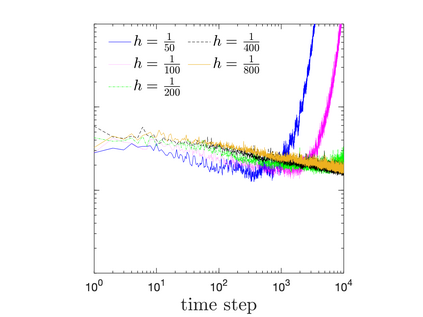
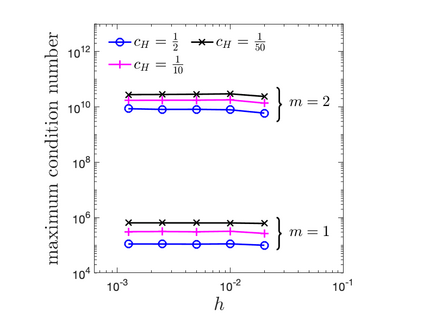
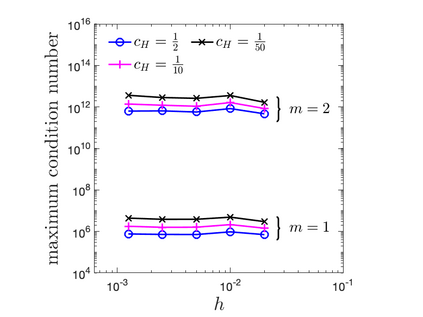
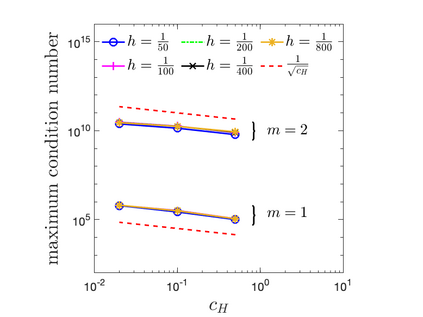
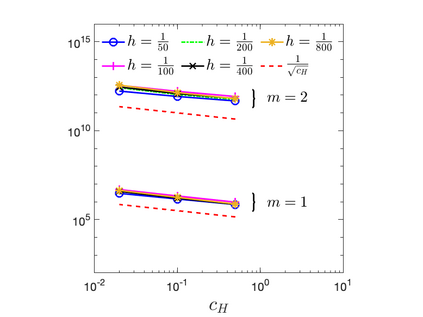
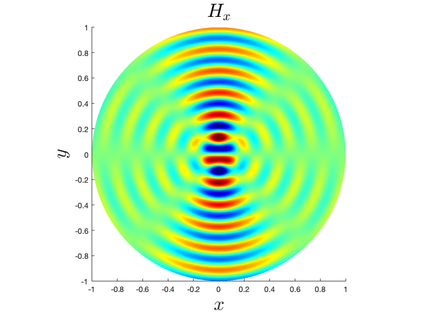
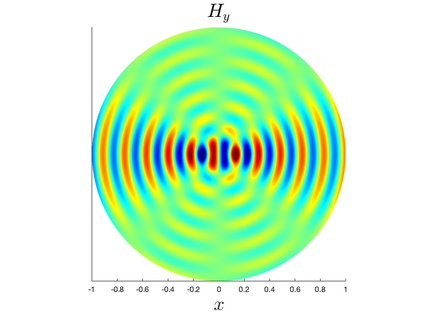
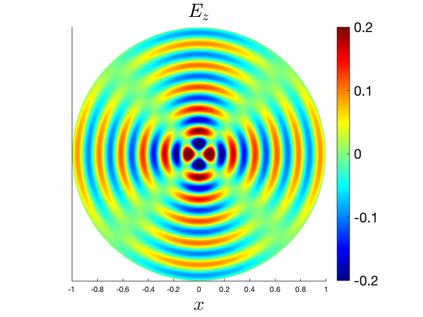
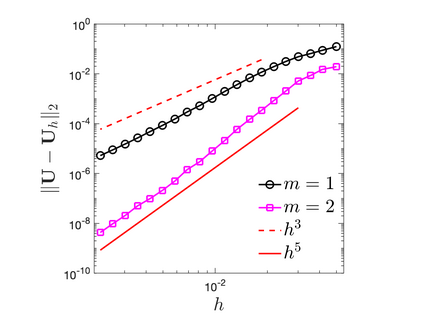
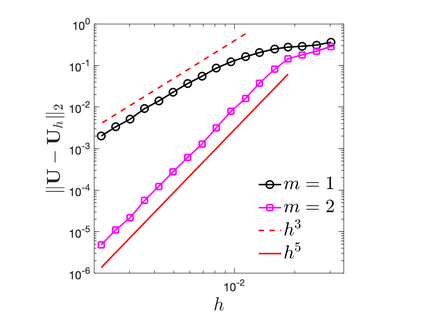
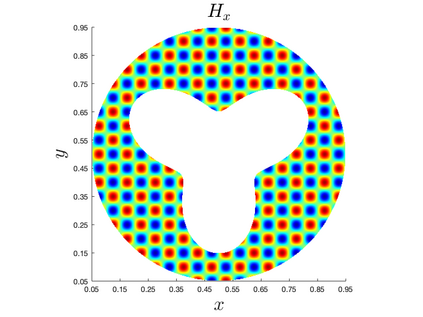
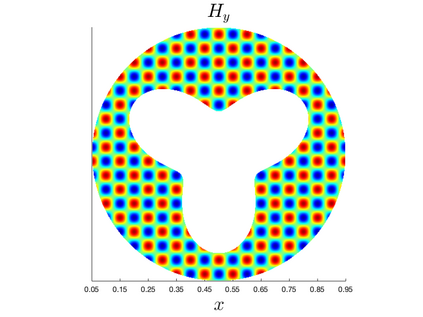
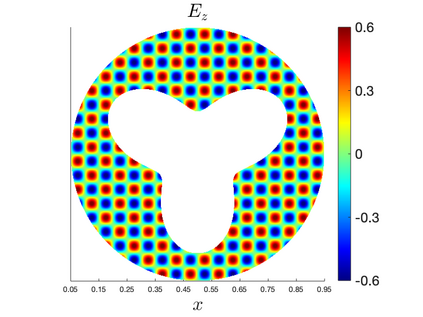
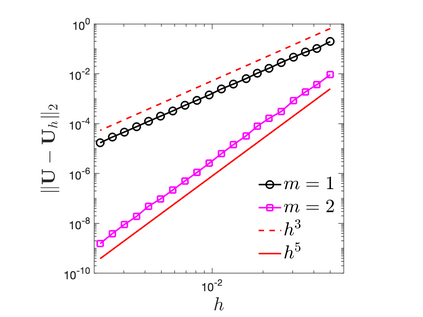
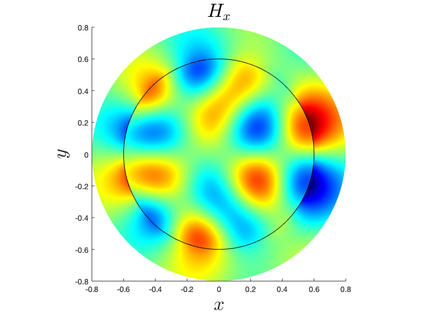
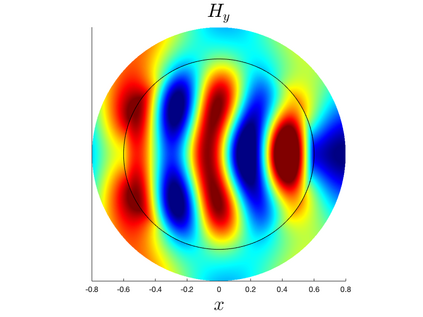
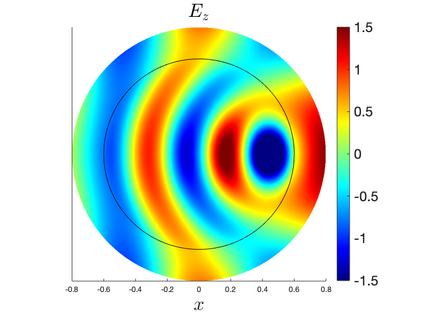
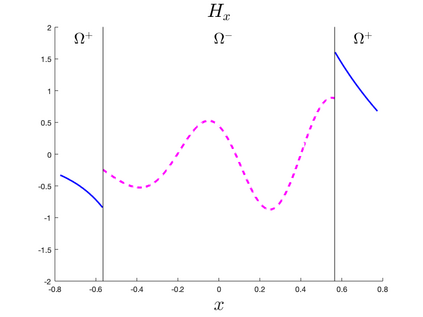
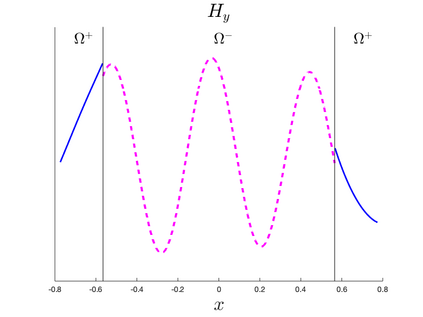
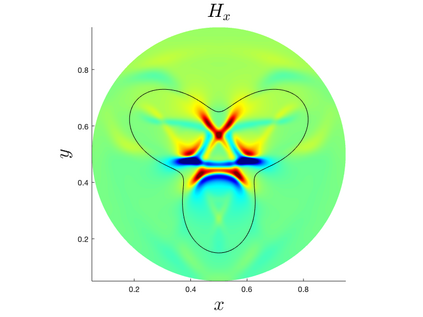
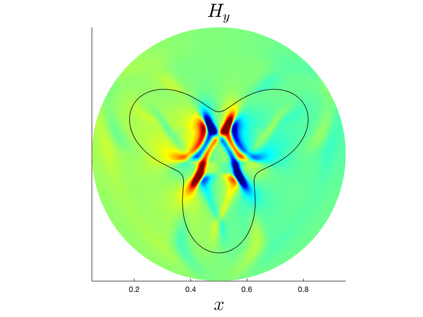
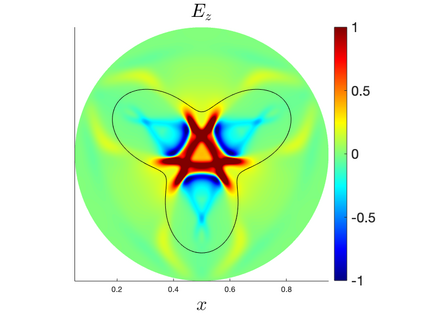
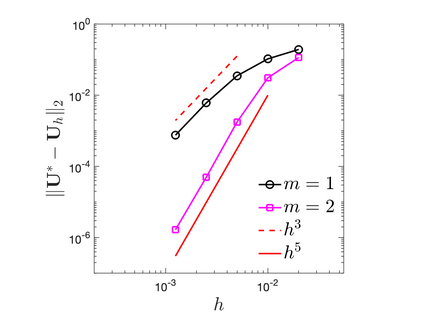
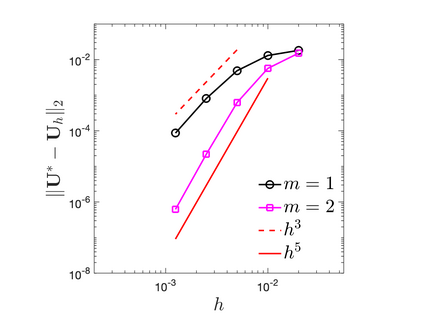
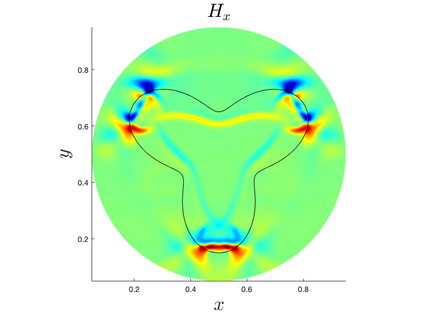
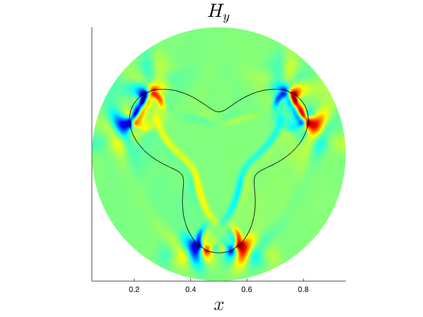
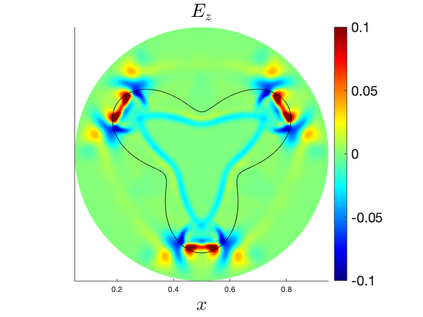
We propose a novel Hermite-Taylor correction function method to handle embedded boundary and interface conditions for Maxwell's equations. The Hermite-Taylor method evolves the electromagnetic fields and their derivatives through order $m$ in each Cartesian coordinate. This makes the development of a systematic approach to enforce boundary and interface conditions difficult. Here we use the correction function method to update the numerical solution where the Hermite-Taylor method cannot be applied directly. The proposed high-order method offers a flexible systematic approach to handle embedded boundary and interface problems, including problems with discontinuous solutions at the interface. This method is also easily adaptable to other first order hyperbolic systems.
翻译:我们建议使用新颖的Hermite-Taylor校正功能方法来处理Maxwell方程式的嵌入边界和界面条件。 Hermite-Taylor方法通过每个笛卡尔坐标点的顺序一百万美元,使电磁场及其衍生物演变成电磁场及其衍生物。 这就使得难以制定系统的方法来强制实施边界和界面条件。 我们在这里使用校正功能方法来更新无法直接应用Hermite-Taylor方法的数字解决方案。 拟议的高阶方法为处理嵌入边界和界面问题提供了灵活的系统方法, 包括界面不连续解决方案的问题。 这种方法也很容易适应其他第一顺序双曲系统 。