A Quasi-Optimal Factorization Preconditioner for Periodic Schrödinger Eigenstates in Anisotropically Expanding Domains

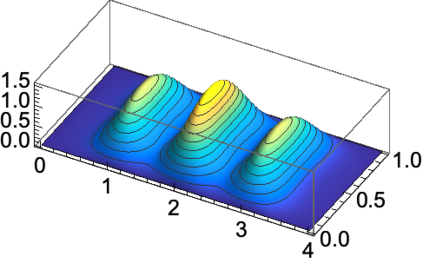
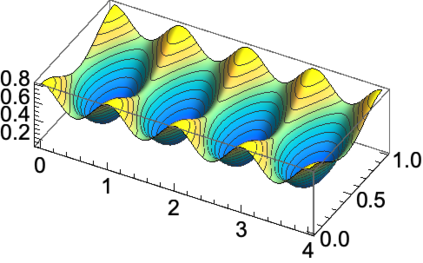
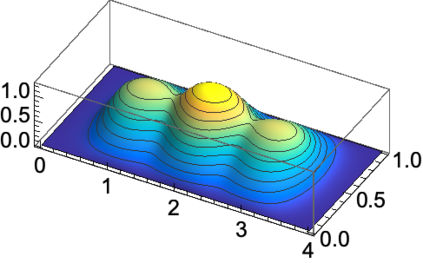
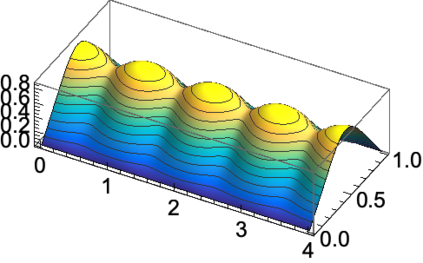
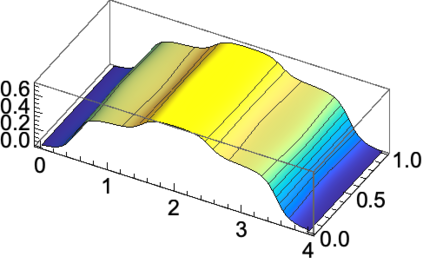
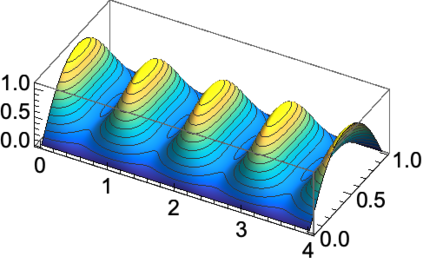
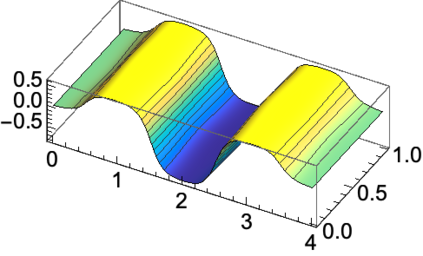
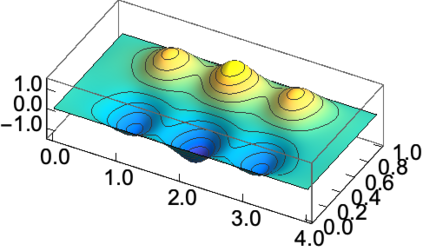
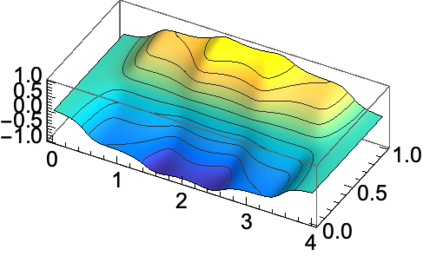
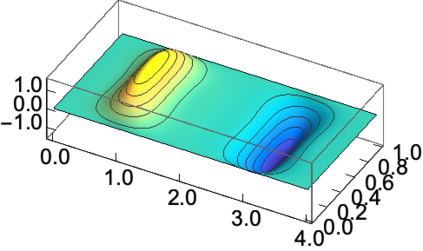
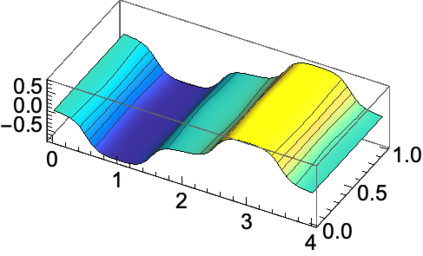
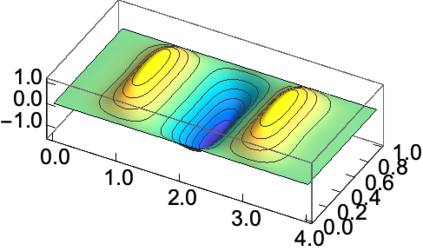
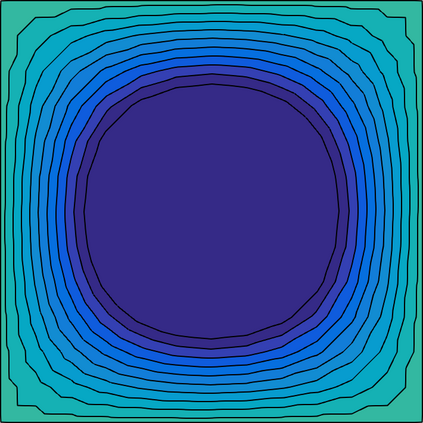
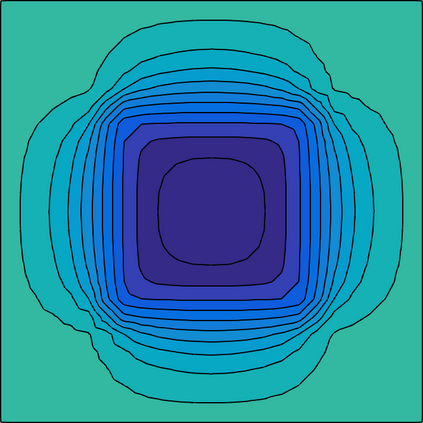
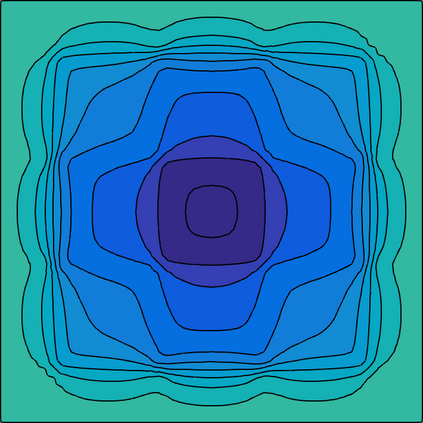
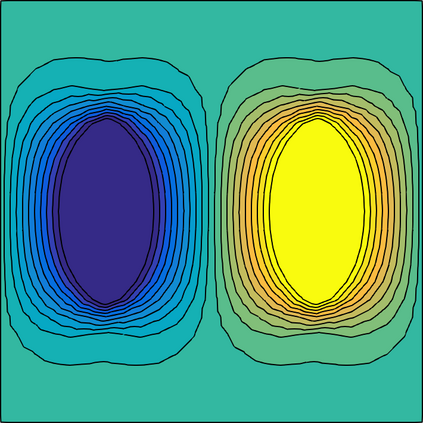
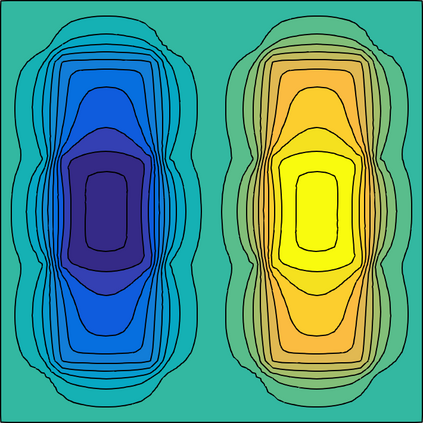
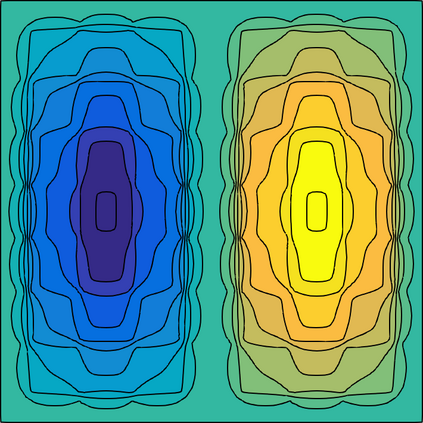
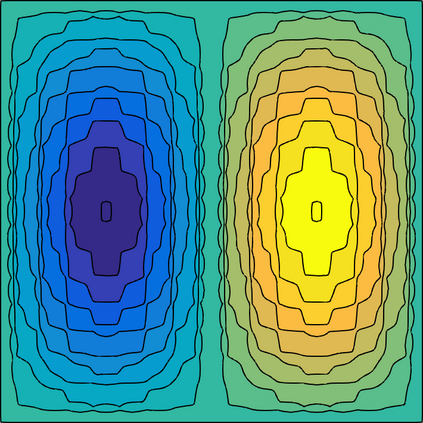
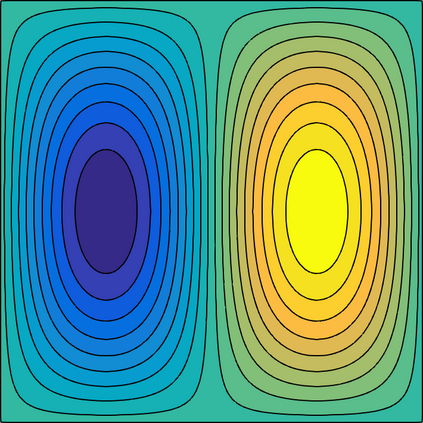
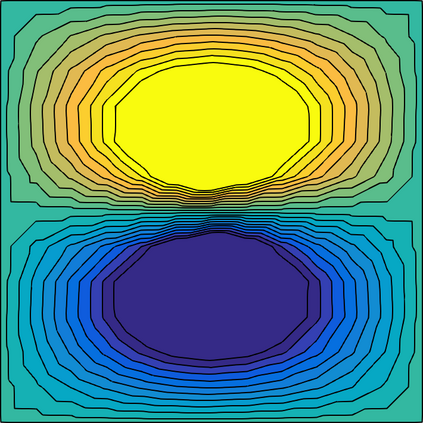
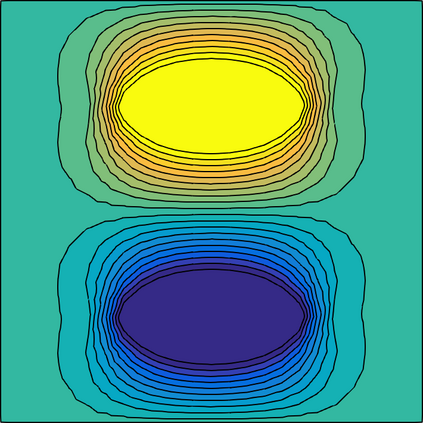
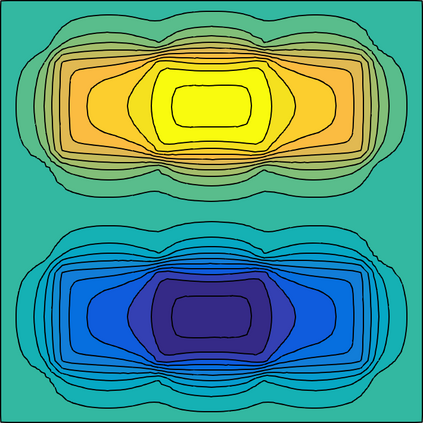
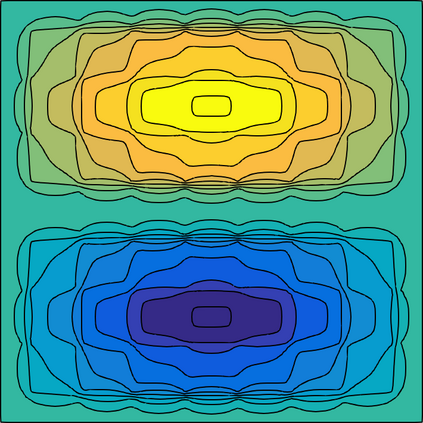
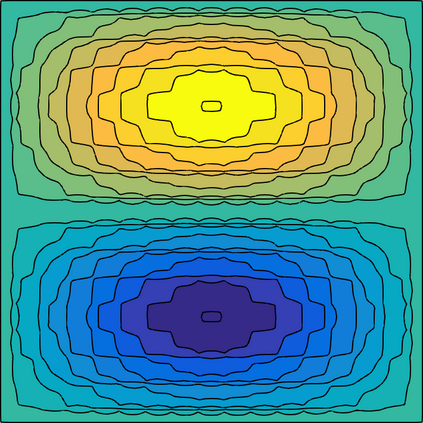
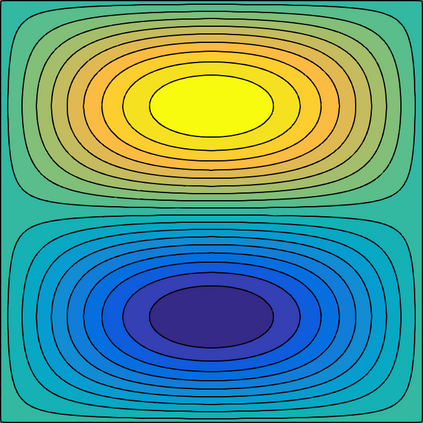
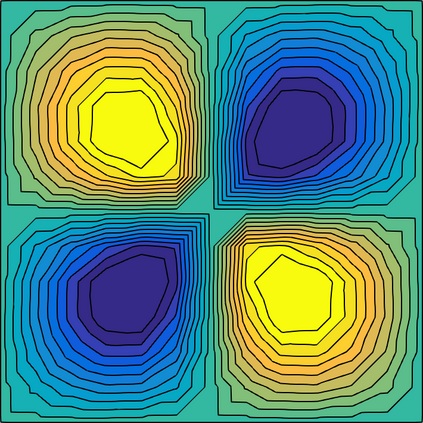
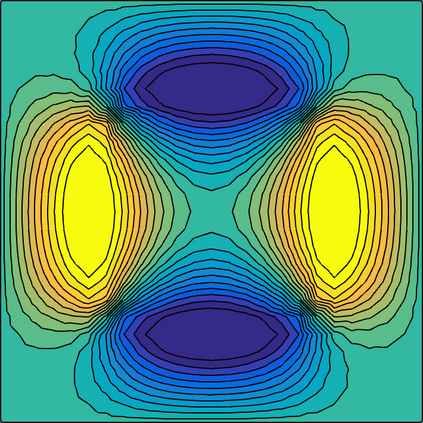
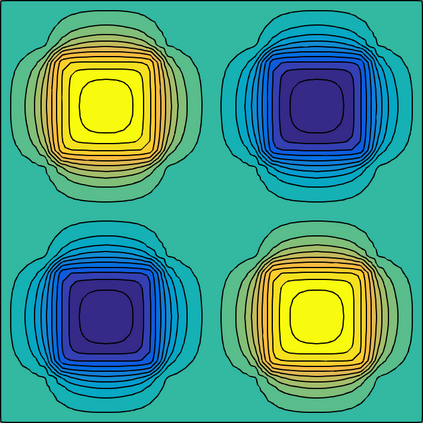
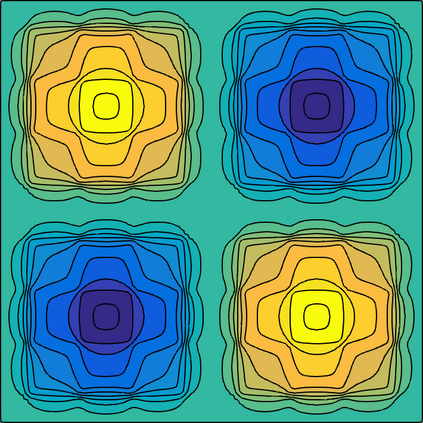
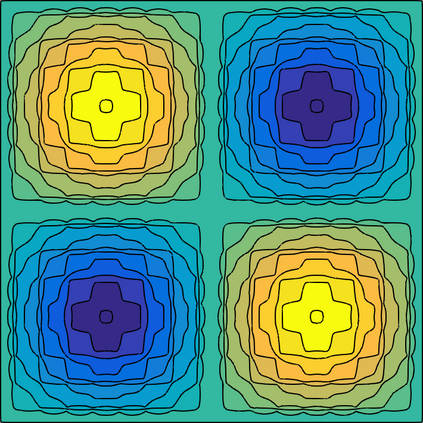
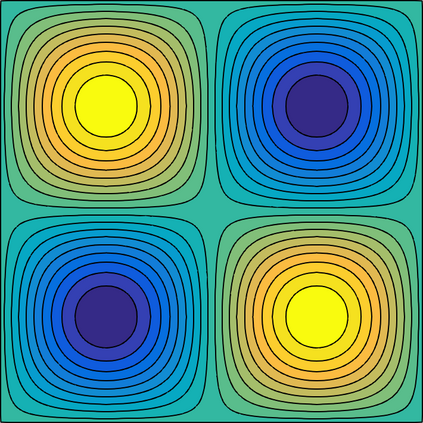
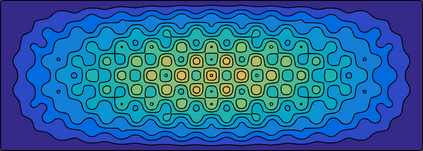
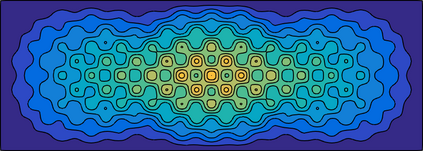
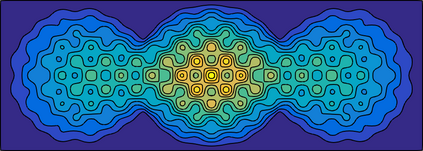
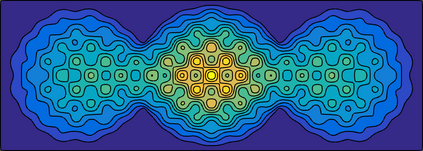
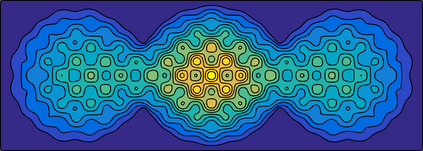
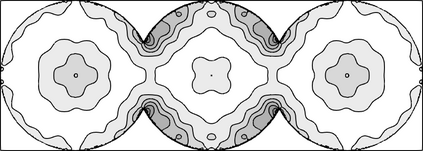
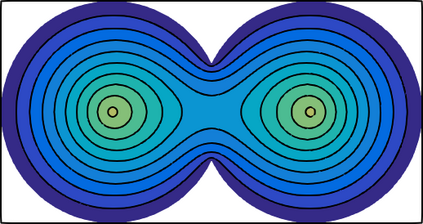
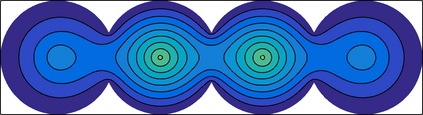
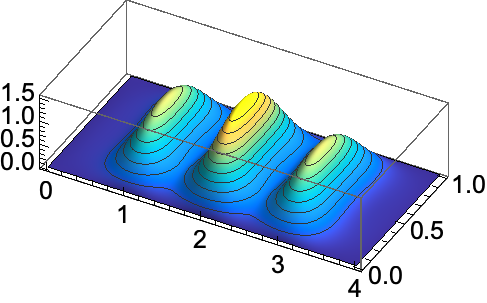
This paper provides a provably quasi-optimal preconditioning strategy of the linear Schr\"odinger eigenvalue problem with periodic potentials for a possibly non-uniform spatial expansion of the domain. The quasi-optimality is achieved by having the iterative eigenvalue algorithms converge in a constant number of iterations for different domain sizes. In the analysis, we derive an analytic factorization of the spectrum and asymptotically describe it using concepts from the homogenization theory. This decomposition allows us to express the eigenpair as an easy-to-calculate cell problem solution combined with an asymptotically vanishing remainder. We then prove that the easy-to-calculate limit eigenvalue can be used in a shift-and-invert preconditioning strategy to bound the number of eigensolver iterations uniformly. Several numerical examples illustrate the effectiveness of this quasi-optimal preconditioning strategy.