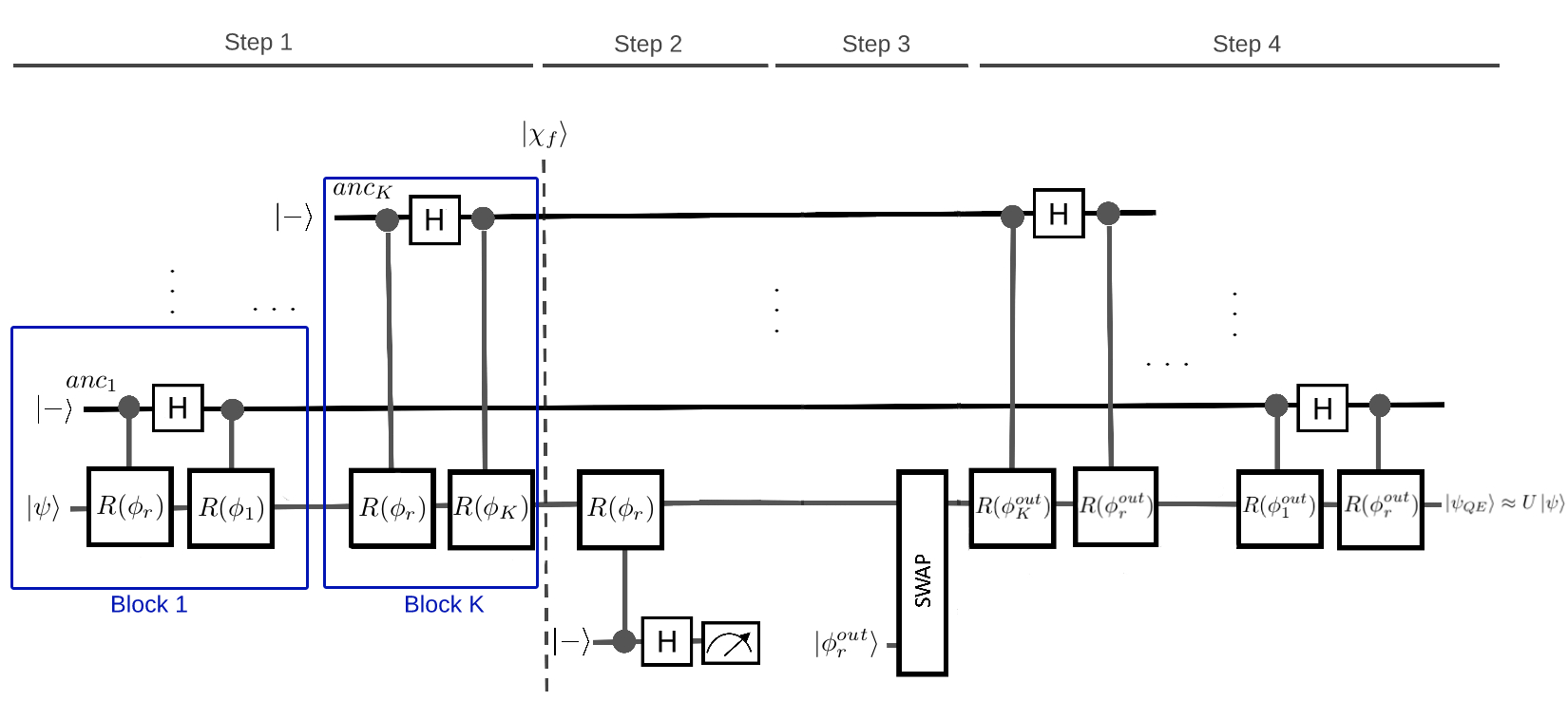
A Physical Unclonable Function (PUF) is a device with unique behaviour that is hard to clone hence providing a secure fingerprint. A variety of PUF structures and PUF-based applications have been explored theoretically as well as being implemented in practical settings. Recently, the inherent unclonability of quantum states has been exploited to derive the quantum analogue of PUF as well as new proposals for the implementation of PUF. We present the first comprehensive study of quantum Physical Unclonable Functions (qPUFs) with quantum cryptographic tools. We formally define qPUFs, encapsulating all requirements of classical PUFs as well as introducing a new testability feature inherent to the quantum setting only. We use a quantum game-based framework to define different levels of security for qPUFs: quantum exponential unforgeability, quantum existential unforgeability and quantum selective unforgeability. We introduce a new quantum attack technique based on the universal quantum emulator algorithm of Marvin and Lloyd to prove no qPUF can provide quantum existential unforgeability. On the other hand, we prove that a large family of qPUFs (called unitary PUFs) can provide quantum selective unforgeability which is the desired level of security for most PUF-based applications.
翻译:物理不协调函数(PUF)是一个独特的装置,其独特的行为举止很难克隆,因此很难提供安全的指纹。各种PUF结构和基于PUF的应用程序已经在理论上进行了探讨,并在实际环境中得到应用。最近,量子国家的内在不协调性被利用来得出PUF的量子类以及实施PUF的新建议。我们用量子加密工具对量子物理不协调函数(qPUF)进行首次全面研究。我们正式定义了qPUF, 包罗了古典PUF的所有要求,并引入了量子设置所固有的新的可测试性特征。我们使用量子游戏框架来界定qPUF的不同安全等级:量子指数不可行、量子生存不可预见性和量子选择性不可靠。我们根据量子模拟算法引入一种新的量子攻击技术,以证明QPUFF不能提供量子生存不易变性。另一方面,我们证明一个大型基于量子游戏的可选择性水平的量子组(所谓的PUFFFP)提供了最理想的安全性水平。