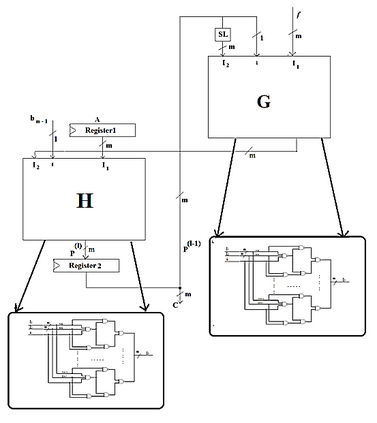
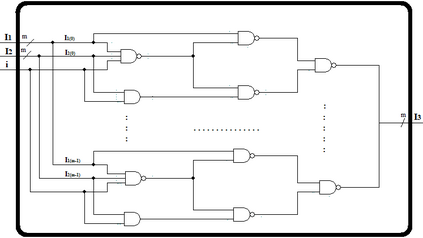
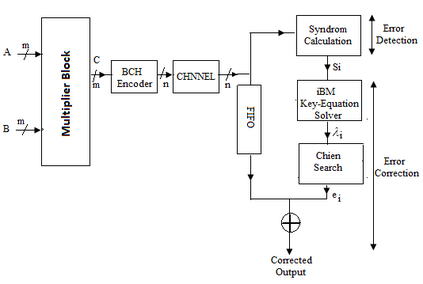
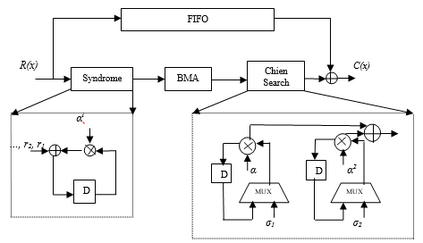
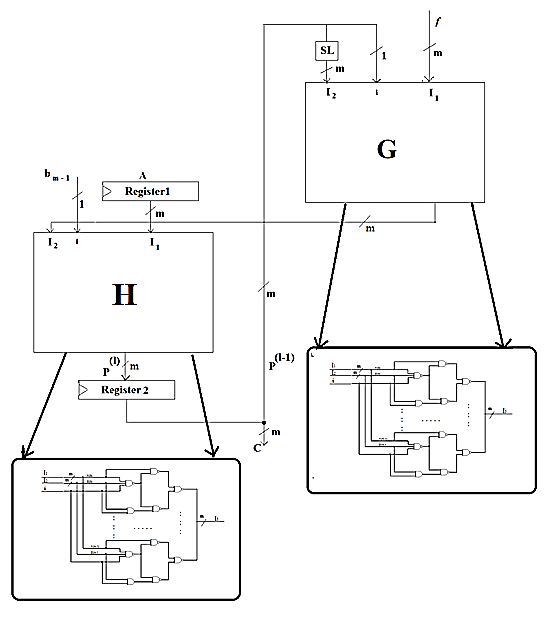
The finite field multiplier is mainly used in many of today's state of the art digital systems and its hardware implementation for bit parallel operation may require millions of logic gates. Natural causes or soft errors in digital design could cause some of these gates to malfunction in the field, which could cause the multiplier to produce incorrect outputs. To ensure that they are not susceptible to error, it is crucial to use a finite field multiplier implementation that is effective and has a high fault detection capability. In this paper, we propose a novel fault detection scheme for a recent bit-parallel polynomial basis multiplier over GF(2m), where the proposed method aims at obtaining high fault detection performance for finite field multipliers and meanwhile maintain low-complexity implementation which is favored in resource constrained applications such as smart cards. The proposed method is based on BCH error correction codes, with an area-delay efficient architecture. The experimental results show that for 45-bit multiplier with 5-bit errors the proposed error detection and correction architecture results in 37% and %49 reduction in critical path delay with compared to the existing method in [18]. Moreover, the area overhead for 45-bit multiplier with 5 errors is within 80% which is significantly lower than the best existing BCH based fault detection method in finite field multiplier [18].
翻译:有限字段乘数主要用于当今现代数字系统的许多状态,其硬件的平行操作的硬件实施可能要求数百万个逻辑门。数字设计中的自然原因或软差错可能导致某些这些门在外地出现故障,从而可能导致乘数产生错误产出。为了确保这些门不会出错,至关重要的是使用有限场乘数实施有效且有高错检测能力的5位错误检测能力。在本文件中,我们提议对最近比GF(2米)的比位平行多核基乘数采用新的故障检测机制,其中拟议方法的目的是为有限的外地乘数取得高错觉检测性能,同时保持低兼容性,这有利于资源受限制的应用程序,如智能卡。拟议方法以BCH误差校正代码为基础,并配有区域卸载效率结构。实验结果表明,45位乘数的测错和校正结构与[18] 现有方法相比,关键路径延迟率减少37%和%49。此外,45位高错位的域位代数执行率比现有5位标准差率率为80,而现有B级测法的误差率为5倍。