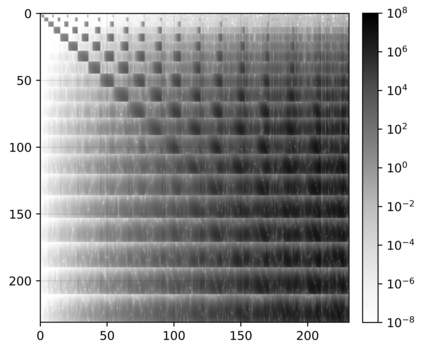
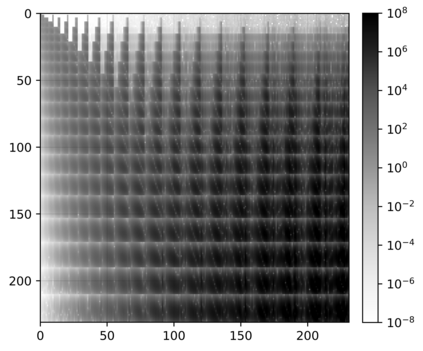
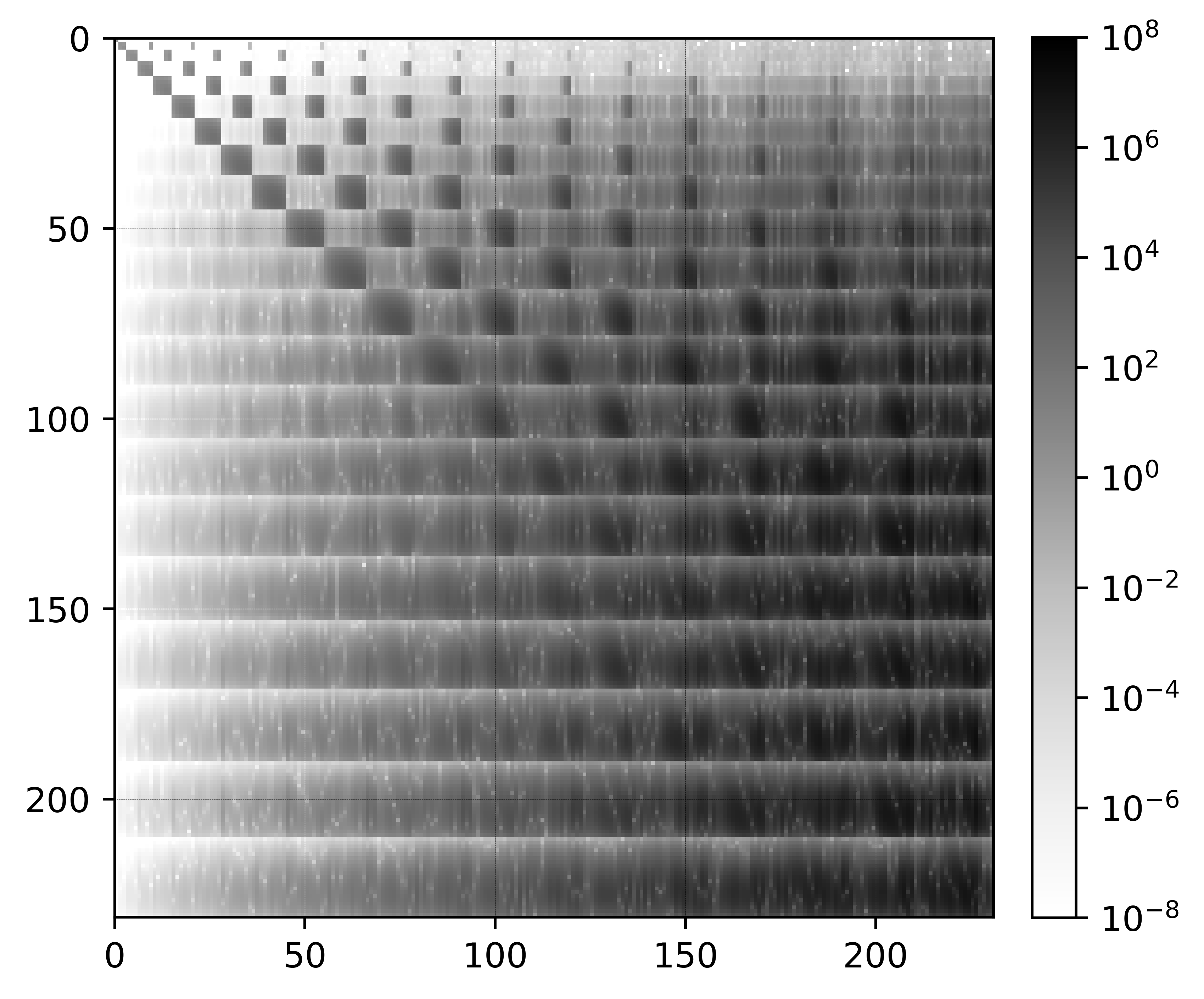
This paper introduces a theoretical framework for investigating analytic maps from finite discrete data, elucidating mathematical machinery underlying the polynomial approximation with least-squares in multivariate situations. Our approach is to consider the push-forward on the space of locally analytic functionals, instead of directly handling the analytic map itself. We establish a methodology enabling appropriate finite-dimensional approximation of the push-forward from finite discrete data, through the theory of the Fourier--Borel transform and the Fock space. Moreover, we prove a rigorous convergence result with a convergence rate. As an application, we prove that it is not the least-squares polynomial, but the polynomial obtained by truncating its higher-degree terms, that approximates analytic functions and further allows for approximation beyond the support of the data distribution. One advantage of our theory is that it enables us to apply linear algebraic operations to the finite-dimensional approximation of the push-forward. Utilizing this, we prove the convergence of a method for approximating an analytic vector field from finite data of the flow map of an ordinary differential equation.
翻译:暂无翻译