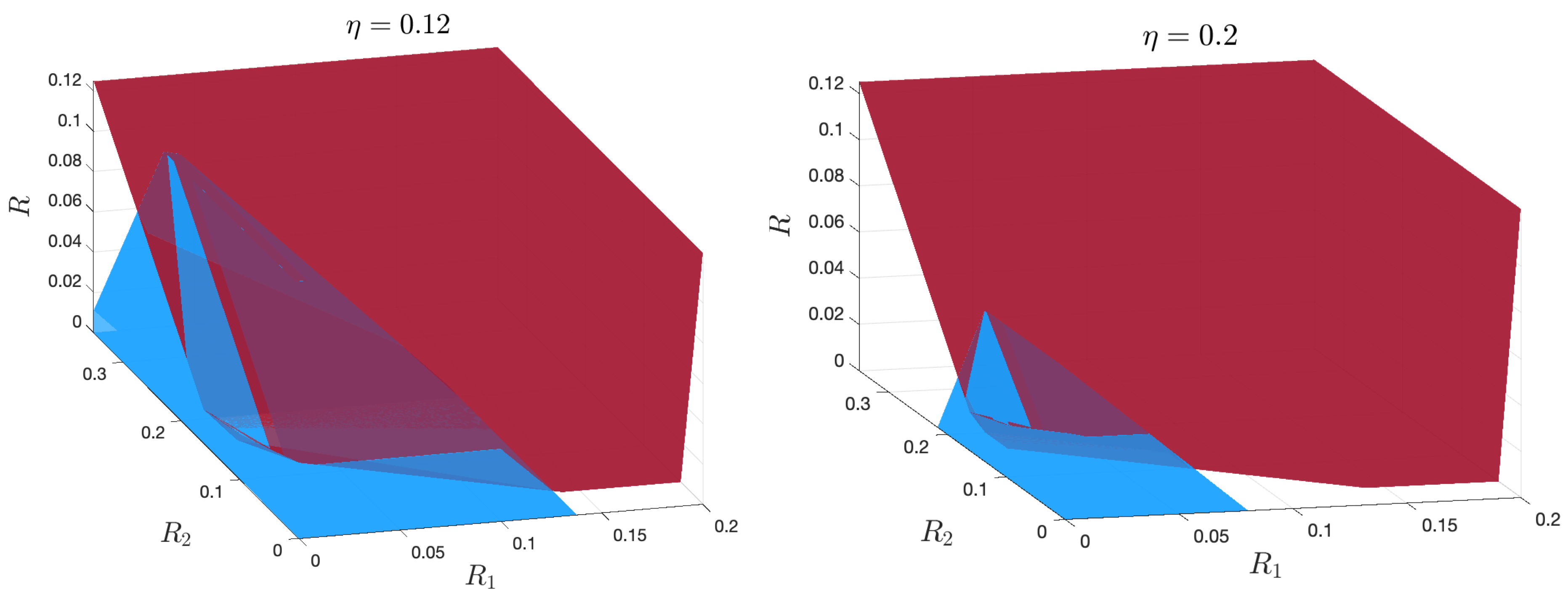
We consider the task of communicating a generic bivariate function of two classical sources over a Classical-Quantum Multiple Access Channel (CQ-MAC). The two sources are observed at the encoders of the CQ-MAC, and the decoder aims at reconstructing a bivariate function from the received quantum state. Inspired by the techniques developed for the analogous classical setting, and employing the technique of simultaneous (joint) decoding developed for the classical-quantum setting, we propose and analyze a coding scheme based on a fusion of algebraic structured and unstructured codes. This coding scheme allows exploiting both the symmetric structure common amongst the sources and the asymmetries. We derive a new set of sufficient conditions that strictly enlarges the largest known set of sources (capable of communicating the bivariate function) for any given CQ-MAC. We provide these conditions in terms of single-letter quantum information-theoretic quantities.
翻译:我们考虑的是用古典-量子多重存取通道(CQ-MAC)传递两种古典来源的通用双变量功能的任务。两种来源在CQ-MAC的编码器上观测到,解码器的目的是从接收量状态中重建一种双变量功能。受为类似古典环境开发的技术的启发,并采用为古典-量子设置开发的同步(联合)解码技术,我们提议和分析一种基于代数结构化和非结构化编码组合的编码办法。这种编码办法允许利用源和不对称的对称结构。我们产生了一套新的充分条件,严格扩大已知的最大源群(能够传输双变量函数),用于任何特定CQ-MAC。我们用单字母量量信息理论提供这些条件。