Superconvergence analysis of interior penalty discontinuous Galerkin method for a class of time-fractional diffusion problems

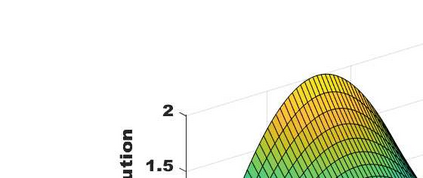
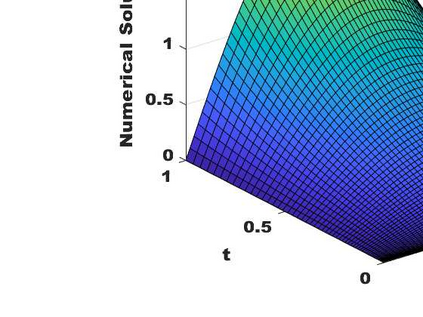
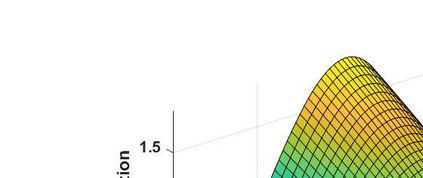
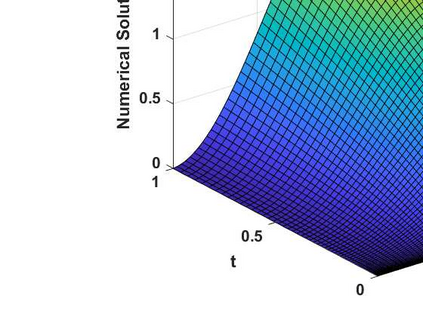
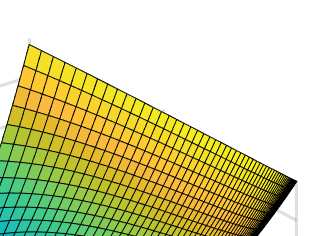
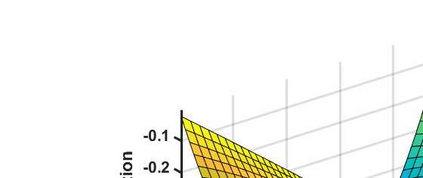
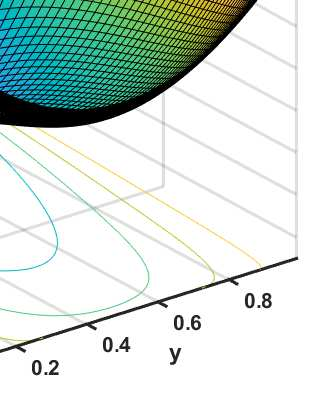
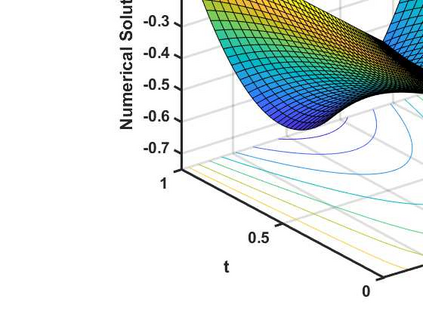
In this study, we consider a class of non-autonomous time-fractional partial advection-diffusion-reaction (TF-ADR) equations with Caputo type fractional derivative. To obtain the numerical solution of the model problem, we apply the non-symmetric interior penalty Galerkin (NIPG) method in space on a uniform mesh and the L1-scheme in time on a graded mesh. It is demonstrated that the computed solution is discretely stable. Superconvergence of error estimates for the proposed method are obtained using the discrete energy-norm. Also, we have applied the proposed method to solve semilinear problems after linearizing by the Newton linearization process. The theoretical results are verified through numerical experiments.