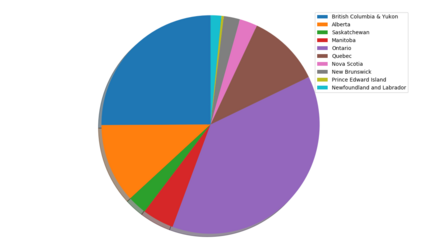
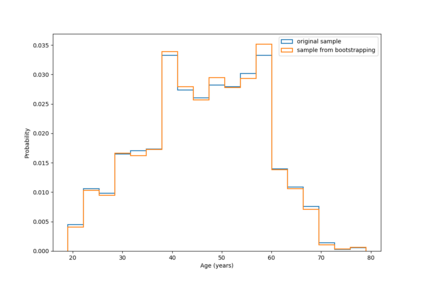
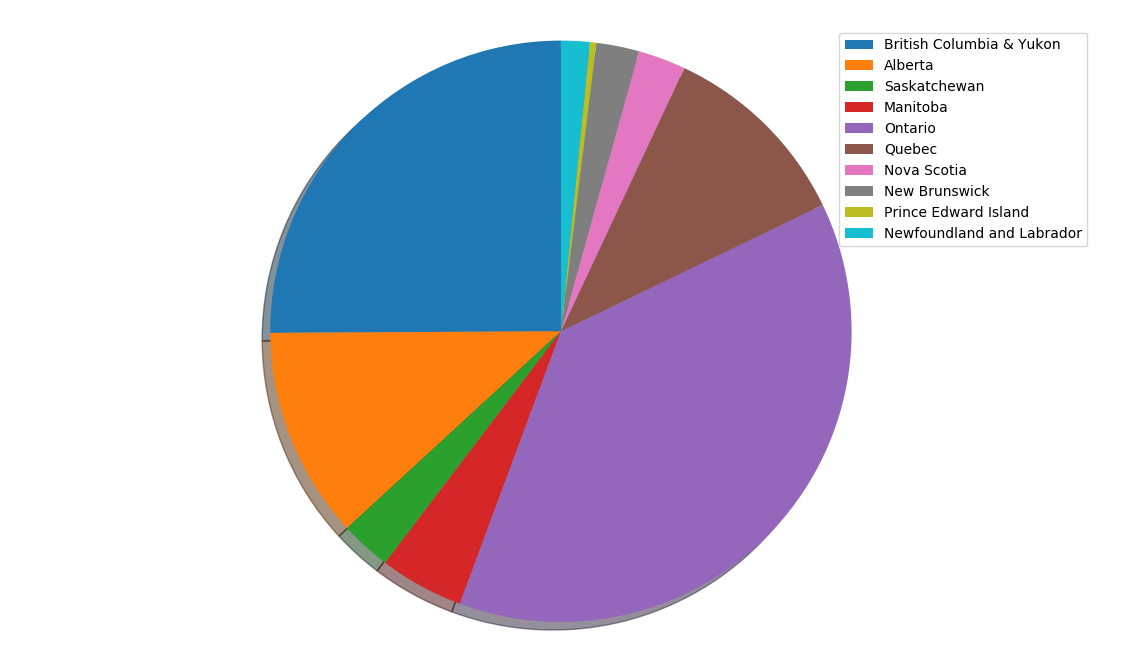
The goal of a kidney exchange program (KEP) is to maximize number of transplants within a pool of incompatible patient-donor pairs by exchanging donors. A KEP can be modelled as a maximum matching problem in a graph. A KEP between incompatible patient-donor from pools of several hospitals, regions or countries has the potential to increase the number of transplants. These entities aim is to maximize the transplant benefit for their patients, which can lead to strategic behaviours. Recently, this was formulated as a non-cooperative two-player game and the game solutions (equilibria) were characterized when the entities objective function is the number of their patients receiving a kidney. In this paper, we generalize these results for $N$-players and discuss the impact in the game solutions when transplant information quality is introduced. Furthermore, the game theory model is analyzed through computational experiments on instances generated through the Canada Kidney Paired Donation Program. These experiments highlighting the importance of using the concept of Nash equilibrium, as well as, the anticipation of the necessity to further research for supporting police makers once measures on transplant quality are available.
翻译:肾脏交换方案(KEP)的目标是通过交换捐赠者,在互换不相容的病人-捐赠者组合中最大限度地增加移植数量。KEP可以模拟成一个图中的最大匹配问题。来自若干医院、地区或国家组合的不相容病人-捐赠者组合中的KEP有可能增加移植数量。这些实体的目标是最大限度地增加其病人的移植好处,从而导致战略行为。最近,这是作为不合作的双人游戏制定的,当实体的客观功能是其接受肾的病人数量时,游戏解决方案(平衡)就被定性为不合作的双人游戏。在本文件中,我们将这些结果概括为美元支付者,并讨论在引入移植信息质量时对游戏解决方案的影响。此外,通过加拿大Kidney Paired Donation方案生成的案例中的计算实验分析游戏理论模型。这些实验强调使用纳什平衡概念的重要性,以及一旦有了关于移植质量的措施,就有必要进一步研究支持警察决策者。