Geographic-style maps for 2-dimensional lattices

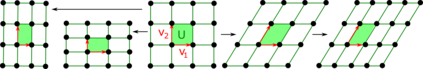
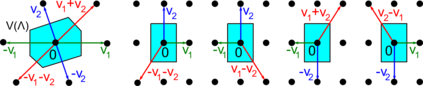
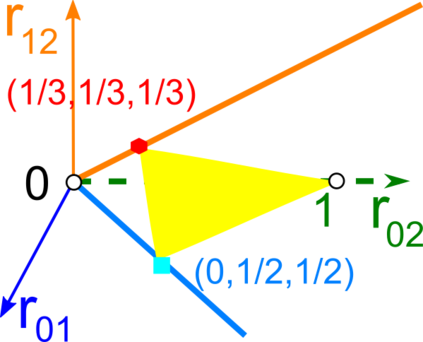
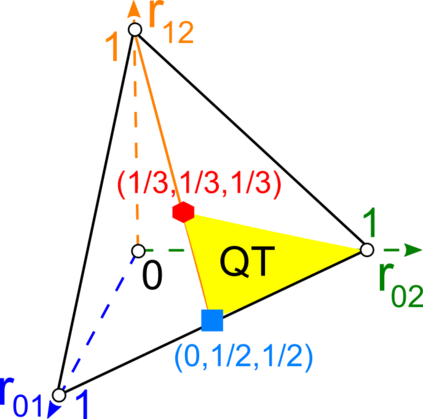
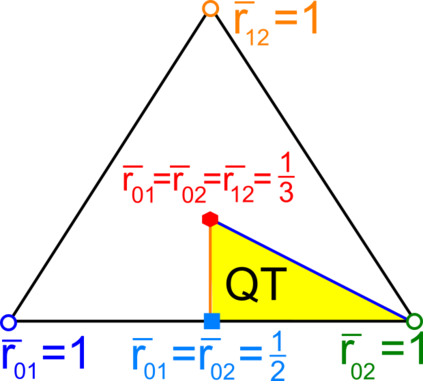
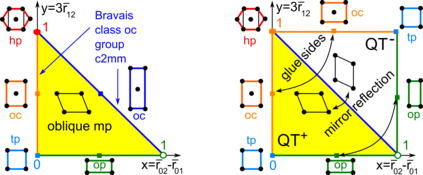
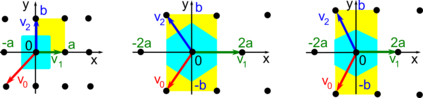
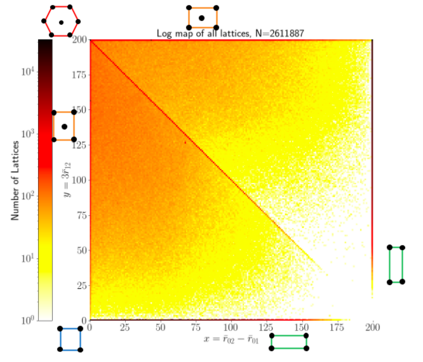
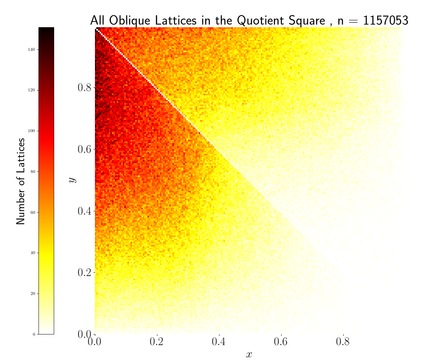
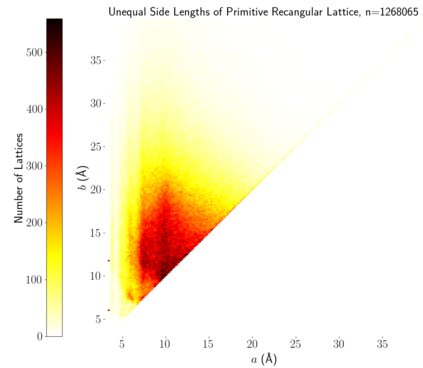
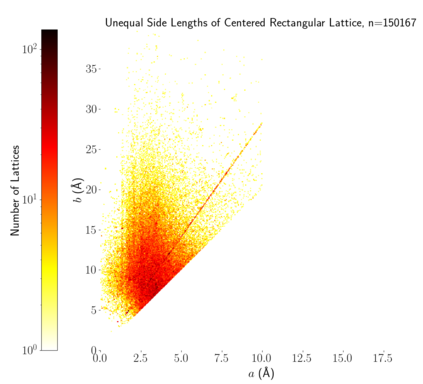
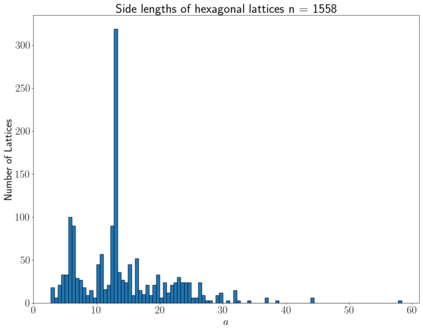
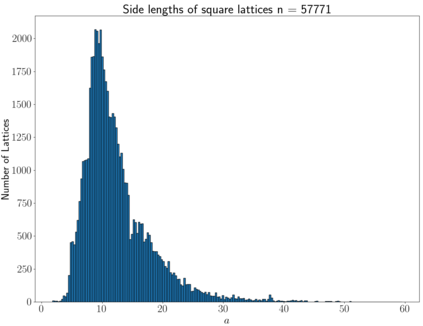
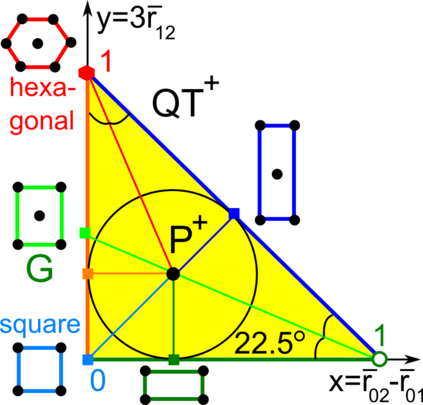
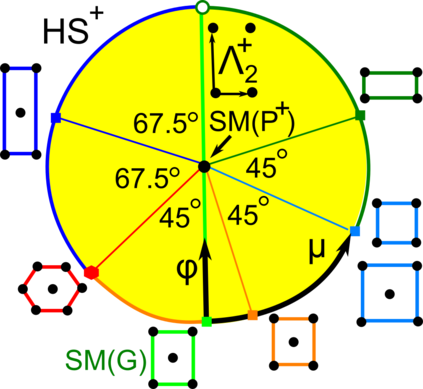
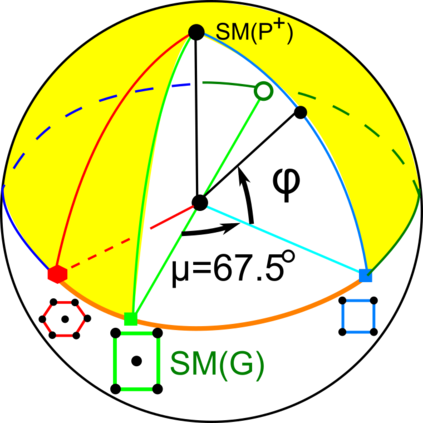
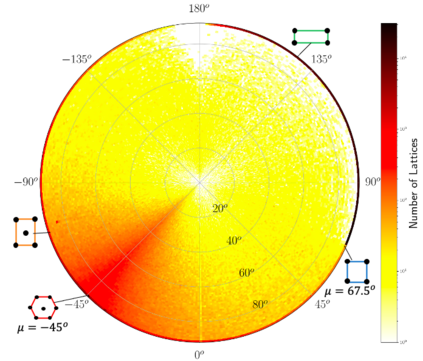
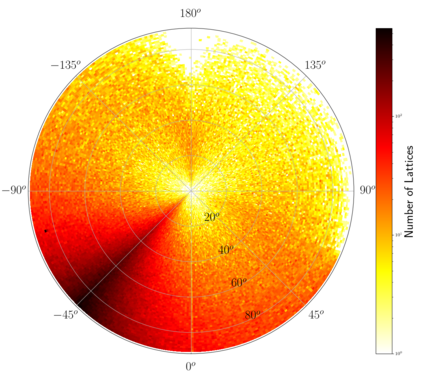
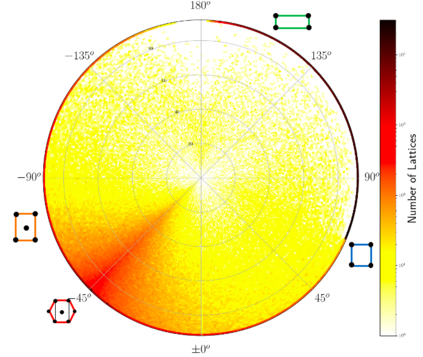
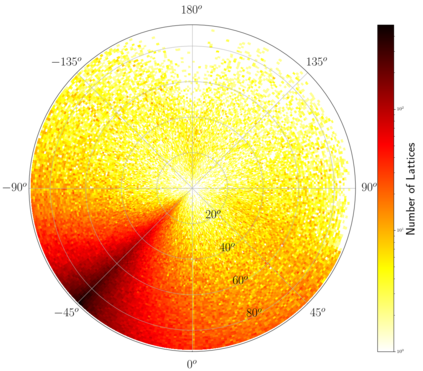
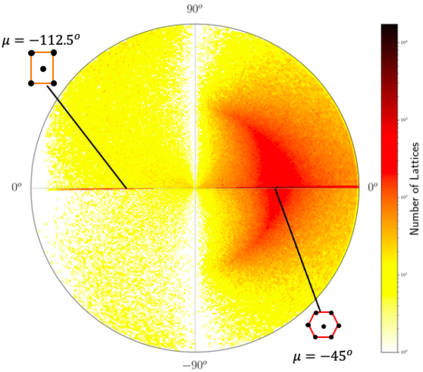
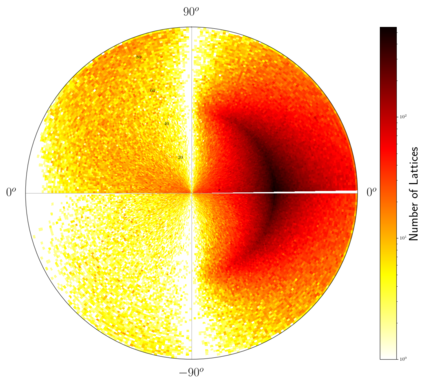
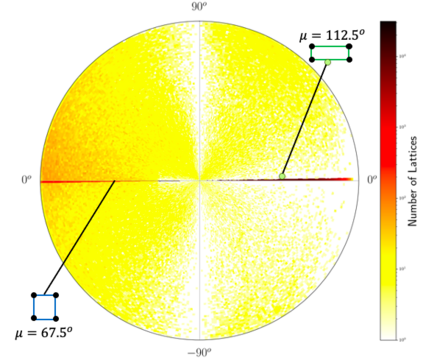
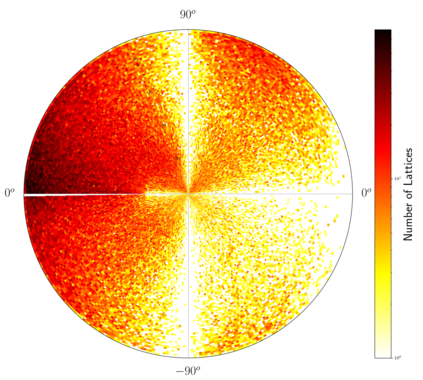
This paper develops geographic-style maps containing 2D lattices in all known crystals parameterised by recent complete invariants. Motivated by rigid crystal structures, lattices are considered up to rigid motion and uniform scaling. The resulting space of 2D lattices is a square with identified edges or a sphere without one point. The new continuous maps show all Bravais classes as low-dimensional subspaces, visualise hundreds of thousands of real crystal lattices from the Cambridge Structural Database, and motivate the development of continuous and invariant-based crystallography.