Multiplicative deconvolution under unknown error distribution

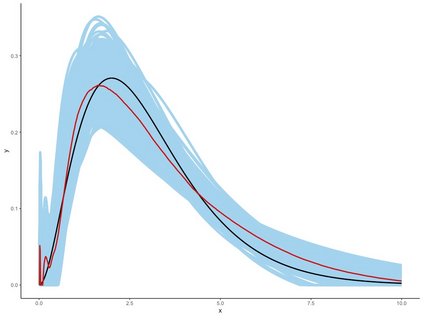
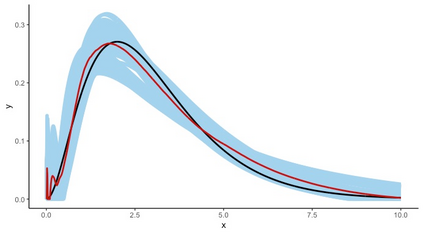
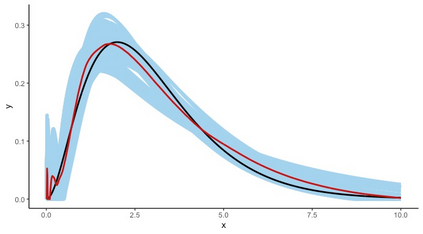
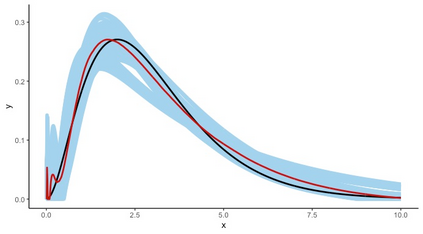
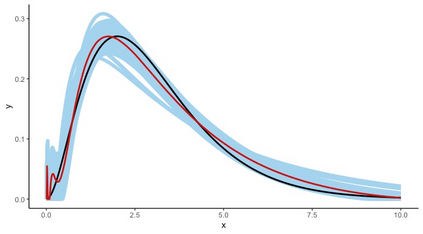
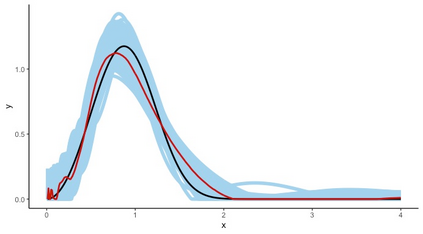
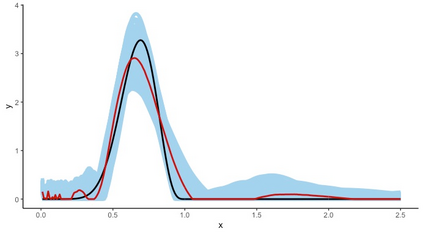
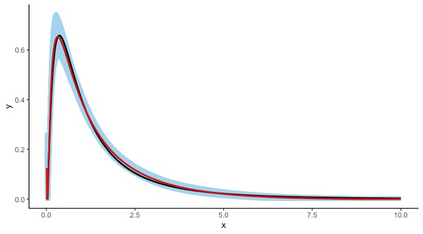
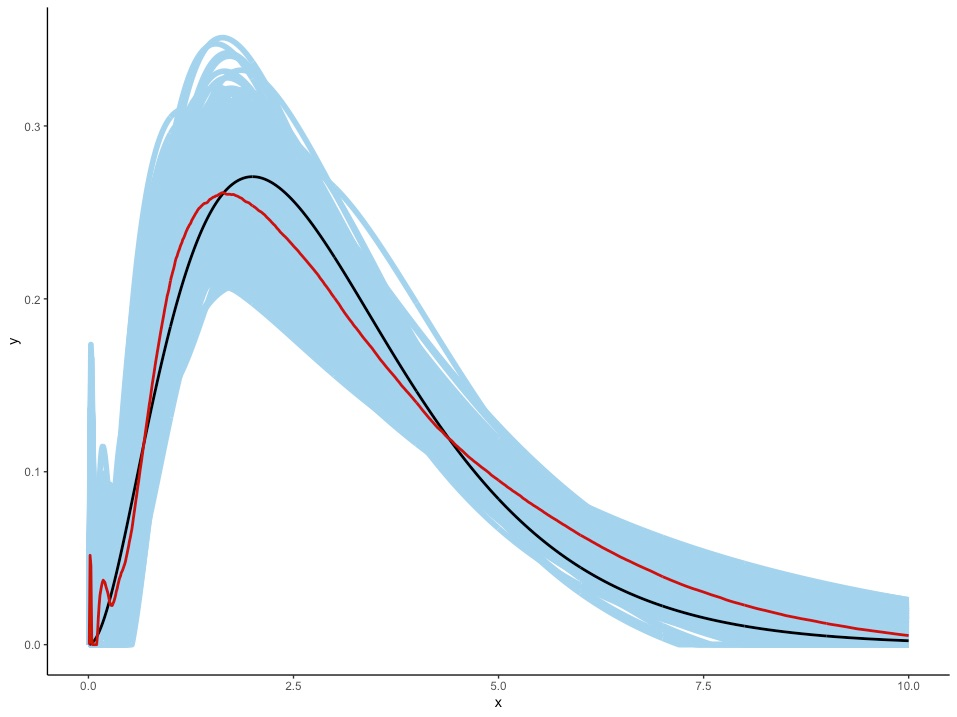
We consider a multiplicative deconvolution problem, in which the density $f$ or the survival function $S^X$ of a strictly positive random variable $X$ is estimated nonparametrically based on an i.i.d. sample from a noisy observation $Y = X\cdot U$ of $X$. The multiplicative measurement error $U$ is supposed to be independent of $X$. The objective of this work is to construct a fully data-driven estimation procedure when the error density $f^U$ is unknown. We assume that in addition to the i.i.d. sample from $Y$, we have at our disposal an additional i.i.d. sample drawn independently from the error distribution. The proposed estimation procedure combines the estimation of the Mellin transformation of the density $f$ and a regularisation of the inverse of the Mellin transform by a spectral cut-off. The derived risk bounds and oracle-type inequalities cover both - the estimation of the density $f$ as well as the survival function $S^X$. The main issue addressed in this work is the data-driven choice of the cut-off parameter using a model selection approach. We discuss conditions under which the fully data-driven estimator can attain the oracle-risk up to a constant without any previous knowledge of the error distribution. We compute convergences rates under classical smoothness assumptions. We illustrate the estimation strategy by a simulation study with different choices of distributions.