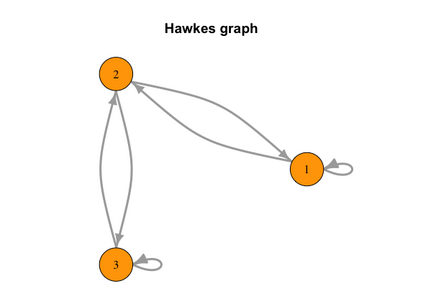
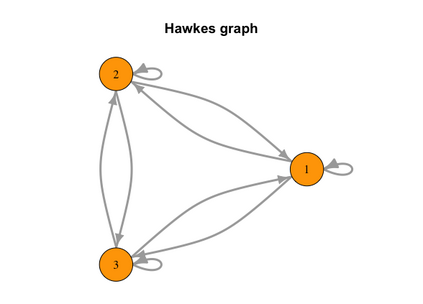
We have established a sparse estimation method for the generalized exponential marked Hawkes process by the penalized method to the ordinary method (P-O) estimator. Furthermore, we evaluated the probability of correct variable selection. In order to achieve this, we established a framework for a likelihood analysis and the P-O estimation when there might be nuisance parameters and the true value of the parameter could be realized at the boundary of the parameter space. Numerical simulations are given for several important examples.
翻译：为了达到这一目的,我们为普遍指数标记霍克斯进程确定了一种稀有的估计方法,即对普通方法(P-O)估测器采用处罚方法。此外,我们评估了正确选择变量的概率。为了达到这一目的,我们建立了一个可能性分析框架和P-O估计框架,当可能存在干扰参数时,参数的真实值可以在参数空间的边界上实现。对几个重要例子进行了数字模拟。
相关内容
Arxiv
0+阅读 · 2021年9月29日
Arxiv
0+阅读 · 2021年9月24日
Arxiv
0+阅读 · 2021年9月24日
Arxiv
0+阅读 · 2021年9月24日
Arxiv
15+阅读 · 2019年1月15日
相关主题
相关VIP内容
相关资讯
相关论文
Arxiv
0+阅读 · 2021年9月29日
Arxiv
0+阅读 · 2021年9月24日
Arxiv
0+阅读 · 2021年9月24日
Arxiv
0+阅读 · 2021年9月24日
Arxiv
15+阅读 · 2019年1月15日
微信扫码咨询专知VIP会员