Fast multipole accelerated solvers for the Laplace-Beltrami problem in three dimensions

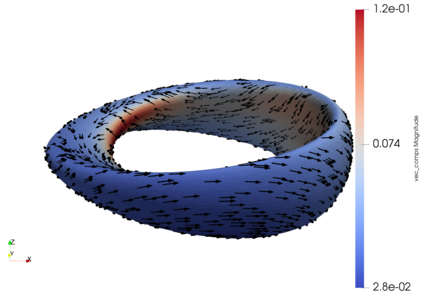
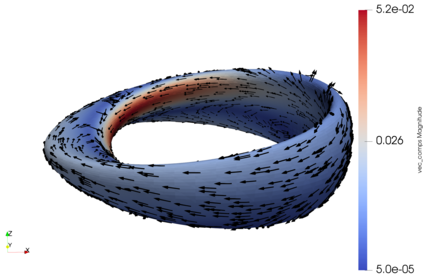
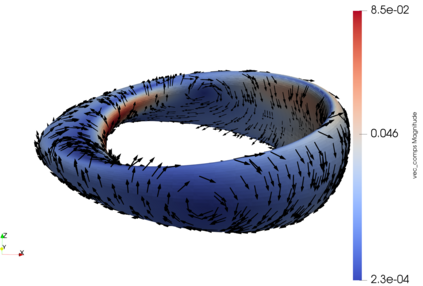
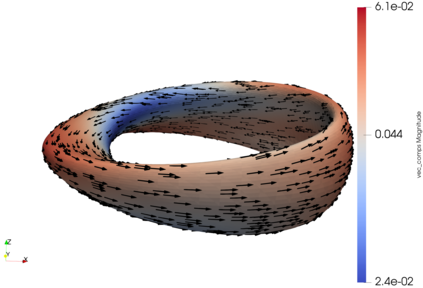
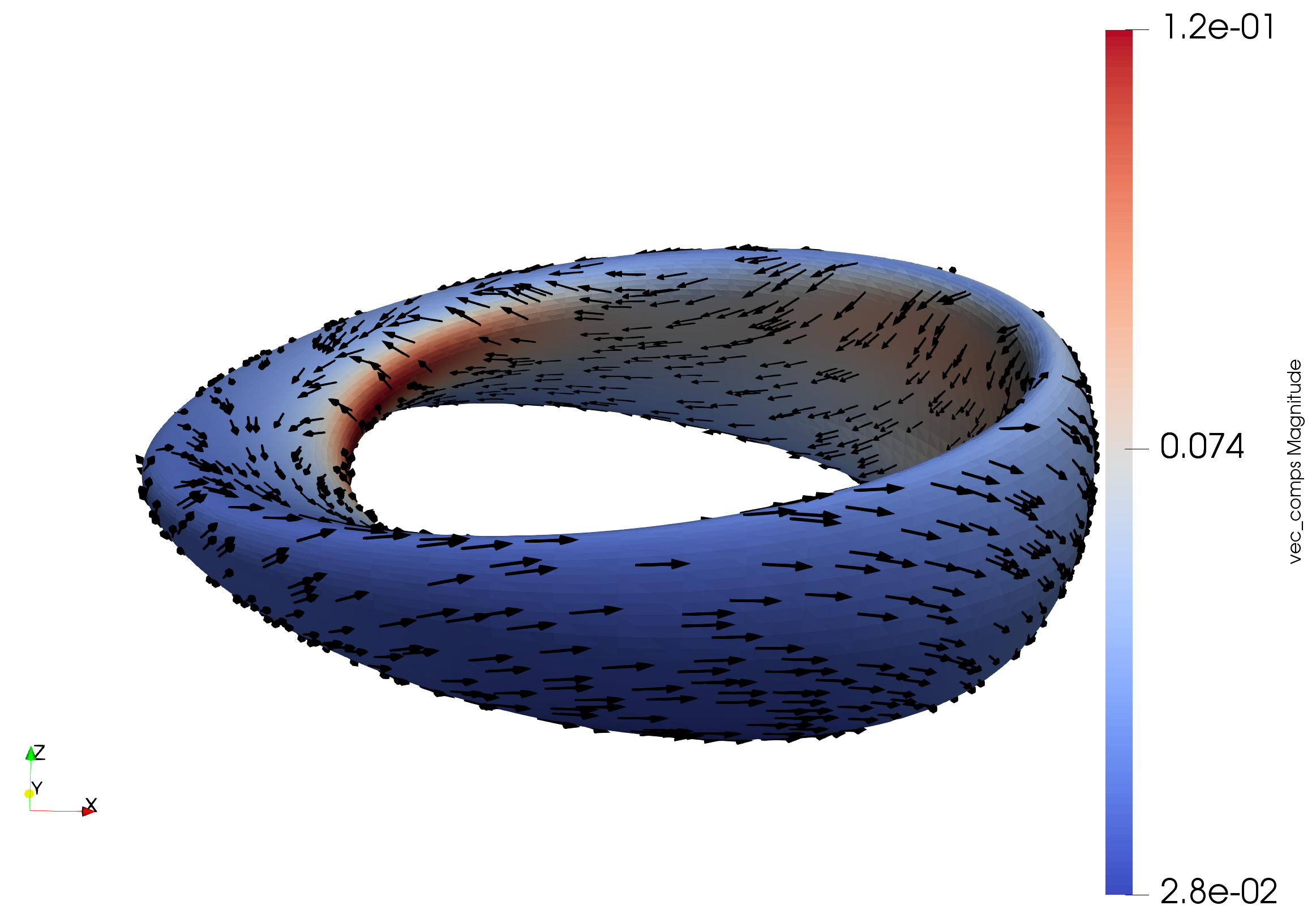
The Laplace-Beltrami problem on closed surfaces embedded in three dimensions arises in many areas of physics, including molecular dynamics (surface diffusion), electromagnetics (harmonic vector fields), and fluid dynamics (vesicle deformation). Using classical potential theory,the Laplace-Beltrami operator can be pre-/post-conditioned with integral operators whose kernel is translation invariant, resulting in well-conditioned Fredholm integral equations of the second-kind. These equations have the standard Laplace kernel from potential theory, and therefore the equations can be solved rapidly and accurately using a combination of fast multipole methods (FMMs) and high-order quadrature corrections. In this work we detail such a scheme, presenting two alternative integral formulations of the Laplace-Beltrami problem, each of whose solution can be obtained via FMM acceleration. We then present several applications of the solvers, focusing on the computation of what are known as harmonic vector fields, relevant for many applications in electromagnetics. A battery of numerical results are presented for each application, detailing the performance of the solver in various geometries.