Bounding the Probabilities of Benefit and Harm Through Sensitivity Parameters and Proxies

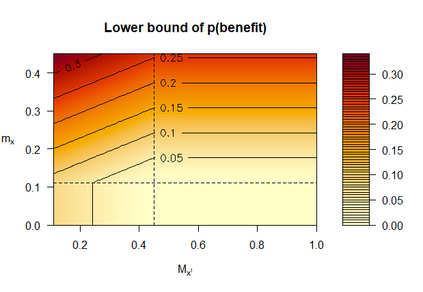
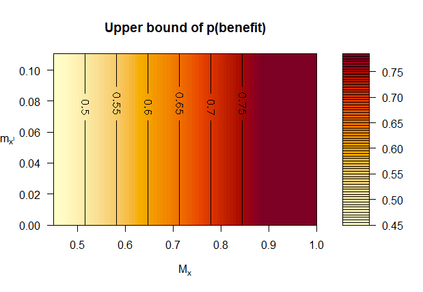
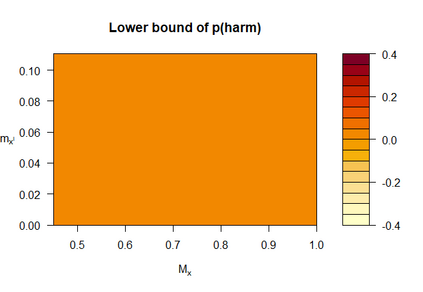
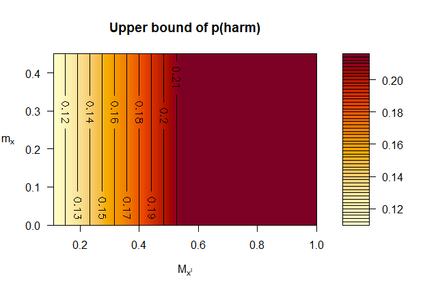
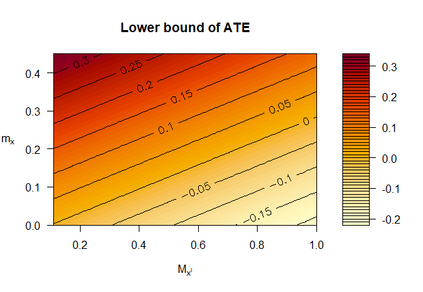
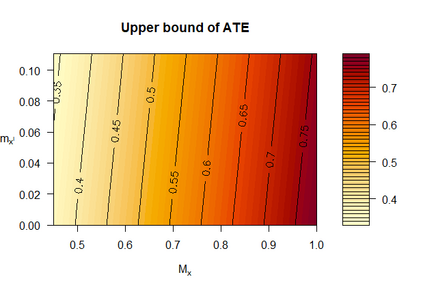
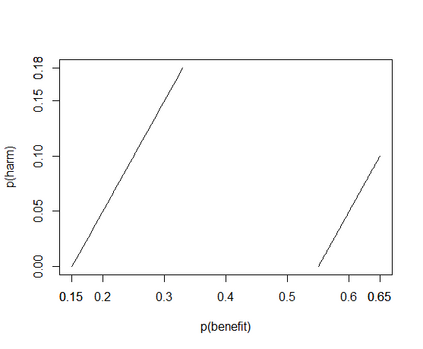
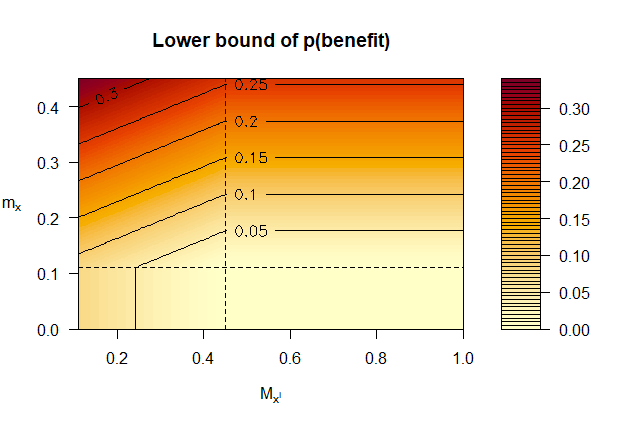
We present two methods for bounding the probabilities of benefit and harm under unmeasured confounding. The first method computes the (upper or lower) bound of either probability as a function of the observed data distribution and two intuitive sensitivity parameters which, then, can be presented to the analyst as a 2-D plot to assist her in decision making. The second method assumes the existence of a measured nondifferential proxy (i.e., direct effect) of the unmeasured confounder. Using this proxy, tighter bounds than the existing ones can be derived from just the observed data distribution.