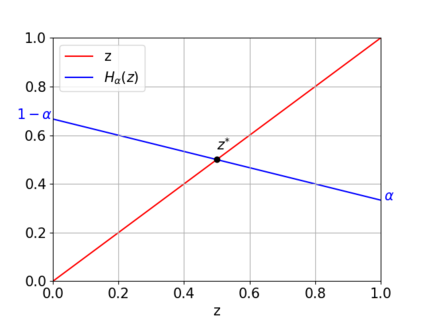
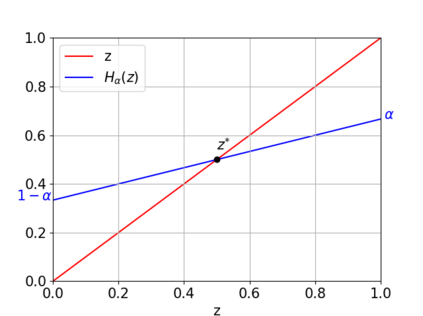
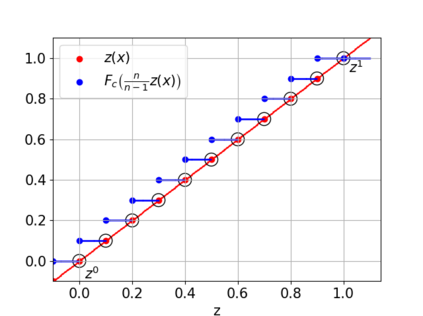
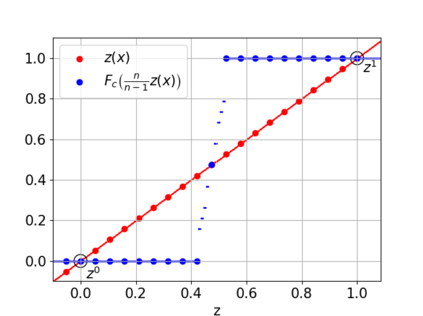
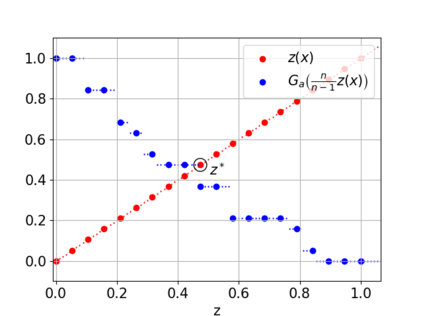
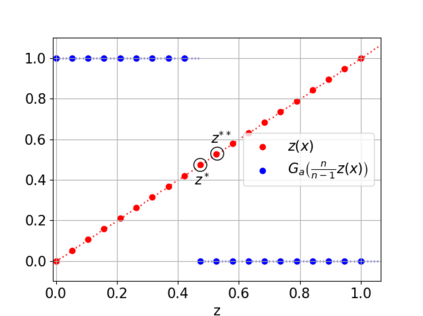
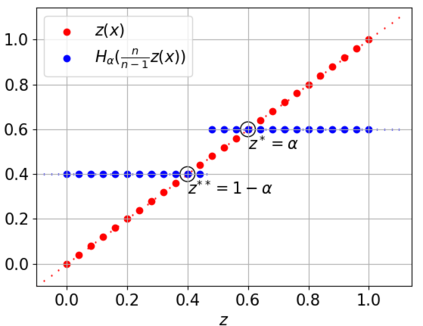
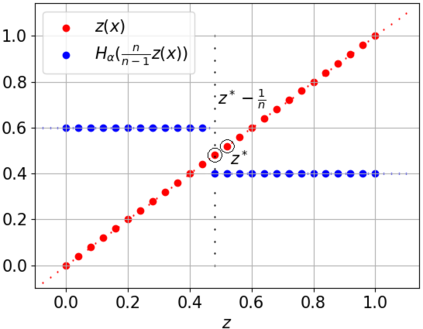
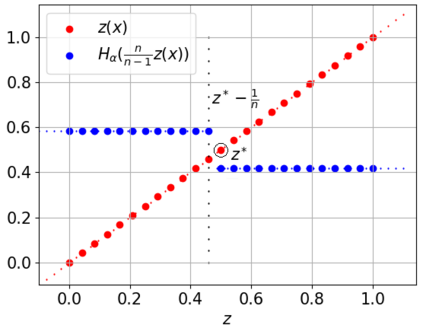
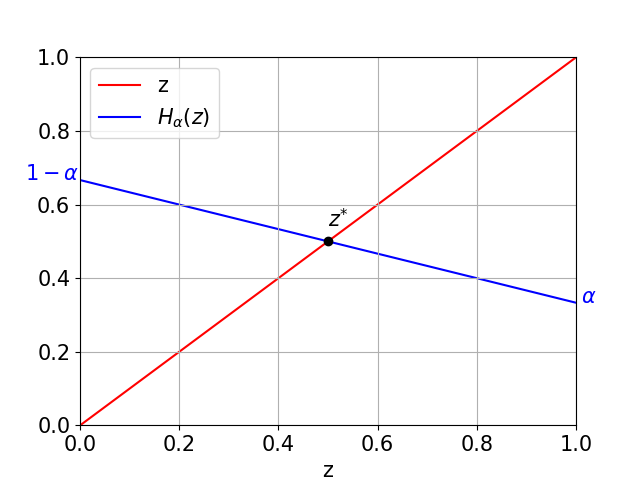
This work studies Nash equilibria for games where a mixture of coordinating and anti-coordinating agents, with possibly heterogeneous thresholds, coexist and interact through an all-to-all network. Whilst games with only coordinating or only anti-coordinating agents are potential, also in the presence of heterogeneities, this does not hold when both type of agents are simultaneously present. This makes their analysis more difficult and existence of Nash equilibria not guaranteed. Our main result is a checkable condition on the threshold distributions that characterizes the existence of Nash equilibria in such mixed games. When this condition is satisfied an explicit algorithm allows to determine the complete set of such equilibria. Moreover, for the special case when only one type of agents is present (either coordinating or anti-coordinating), our results allow an explicit computation of the cardinality of Nash equilibria.
翻译:这份工作研究对游戏的Nash平衡性进行了研究,在游戏中,协调和反协调的混合物剂(可能具有各种不同的临界值)通过一个全方位网络共存和互动。 虽然只有协调物剂或只有反协调物剂的游戏是可能的,但也存在差异性,但当这两种物剂同时存在时,这种平衡性并不有效。这使得它们的分析更加困难,纳什平衡性的存在得不到保障。我们的主要结果就是在这种混合物游戏中存在纳什平衡的临界值分布上有一个可核实的条件。如果满足这一条件,明确的算法就可以确定这种平衡的完整组合。此外,对于只有一种物剂存在的特殊情况(协调或反协调),我们的结果允许明确计算纳什平衡的基本性。