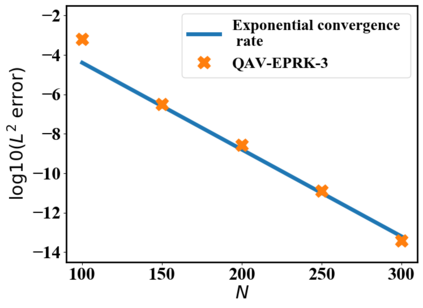
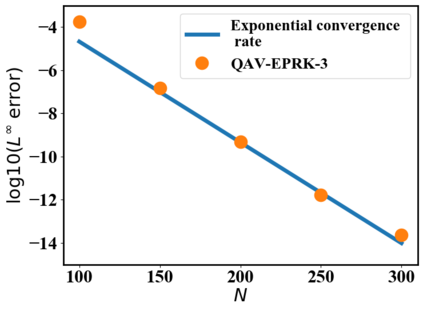
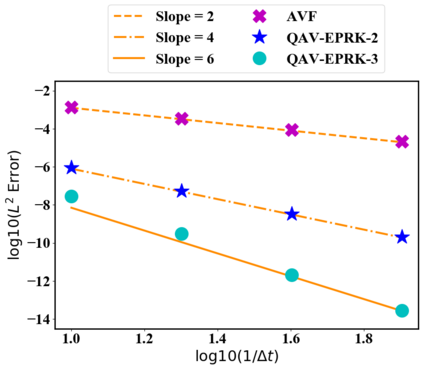
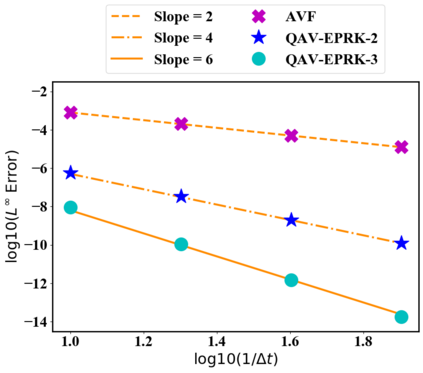
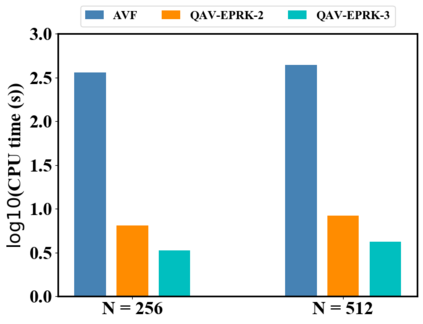
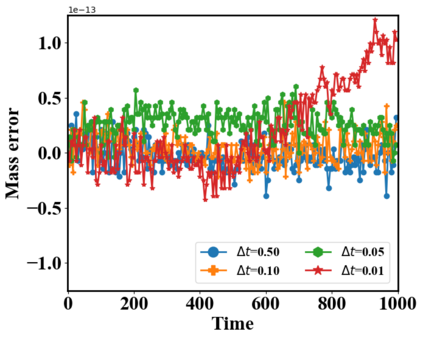
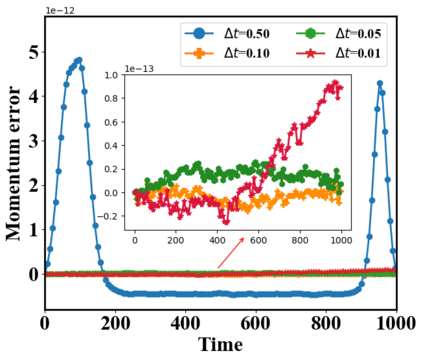
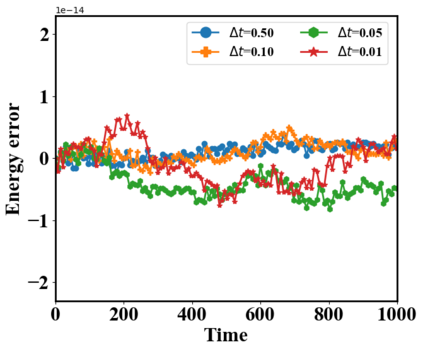
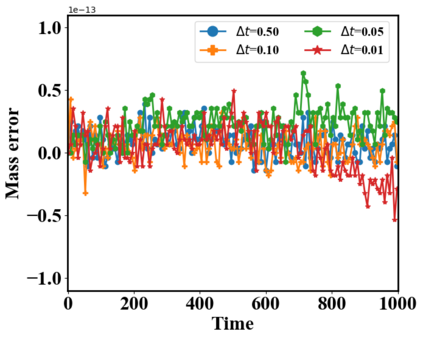
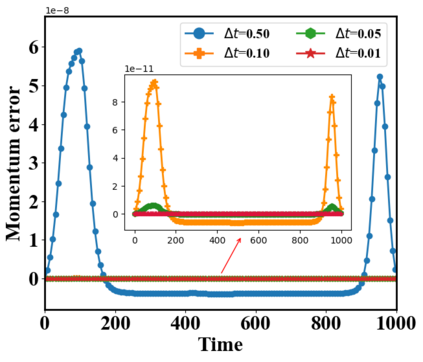
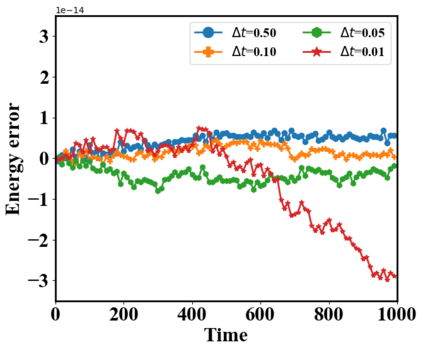
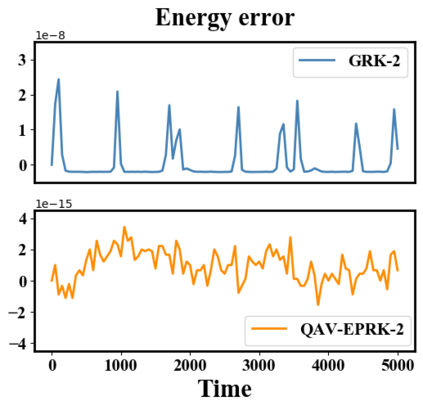
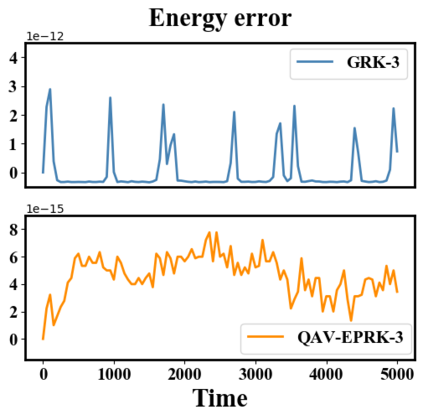
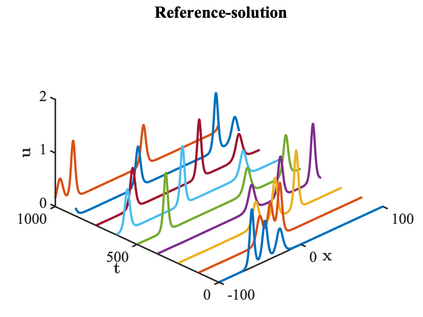
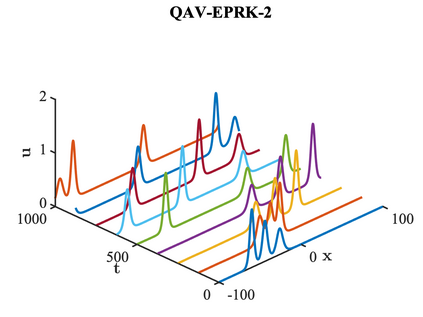
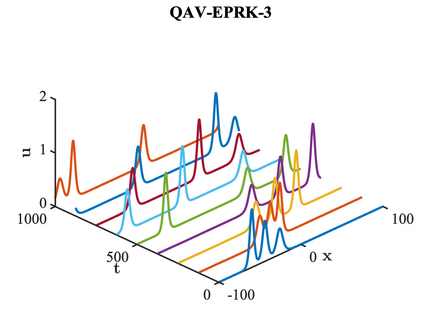
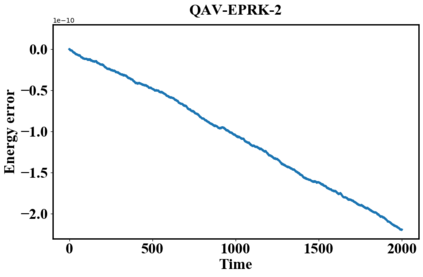
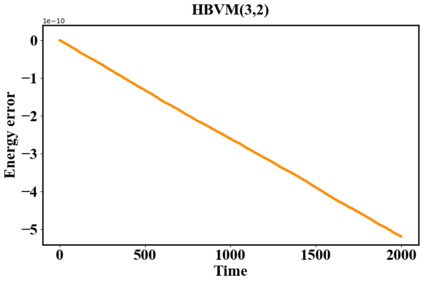
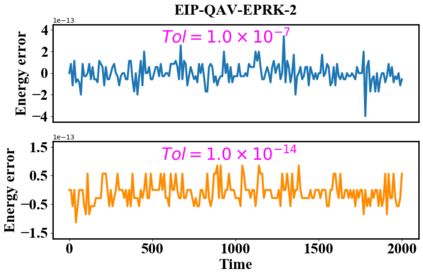
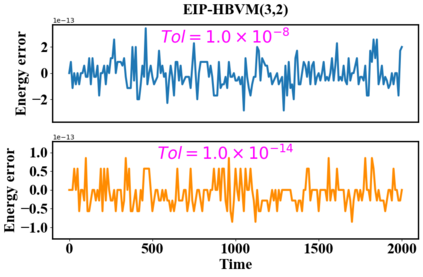
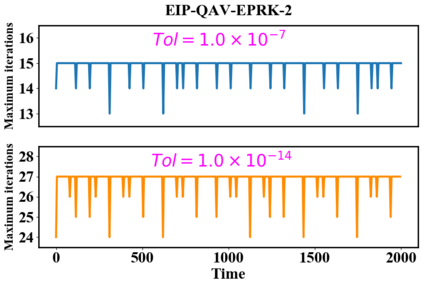
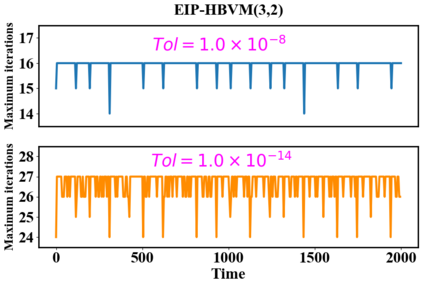
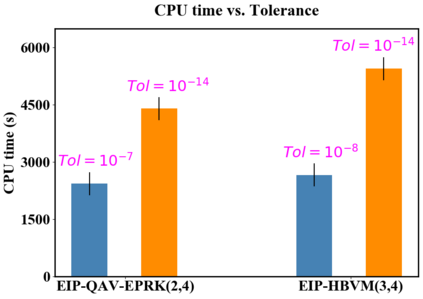
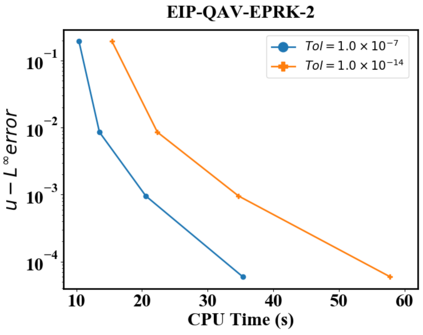
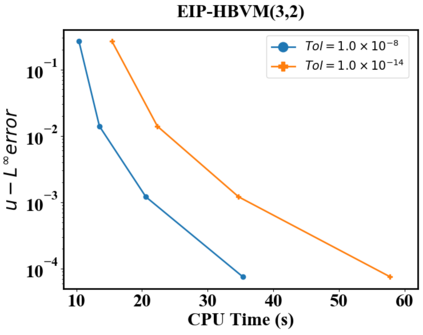
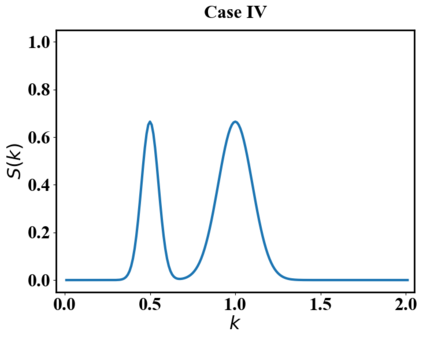
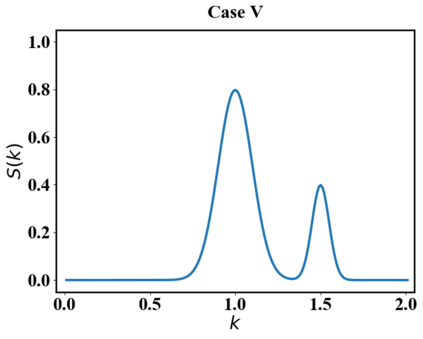
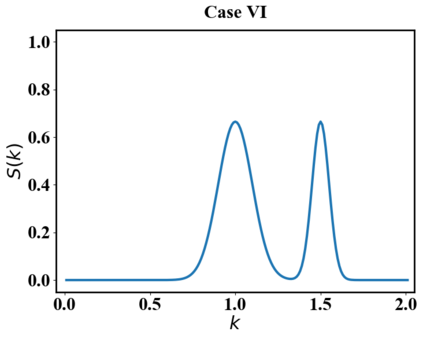
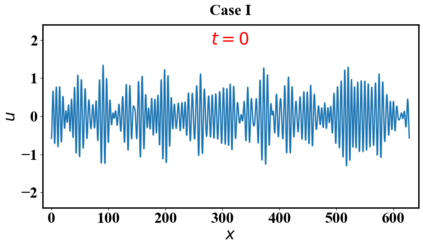
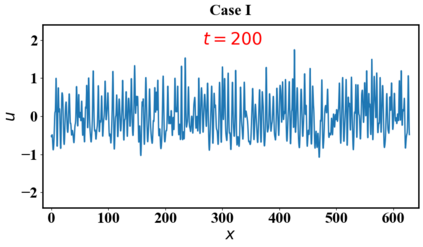
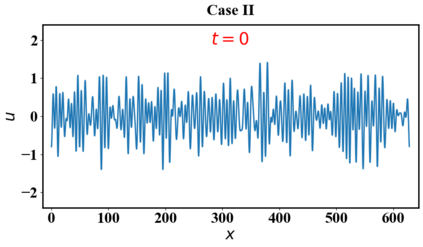
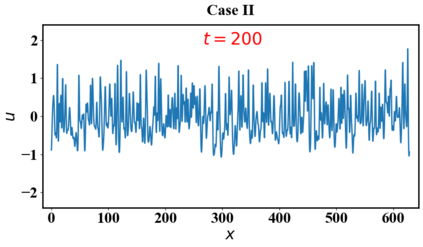
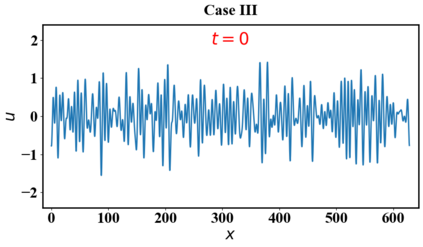
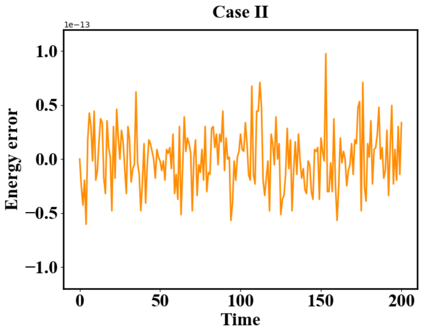
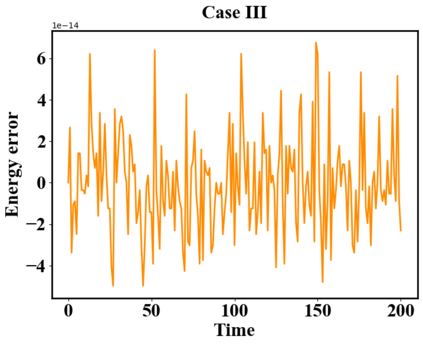
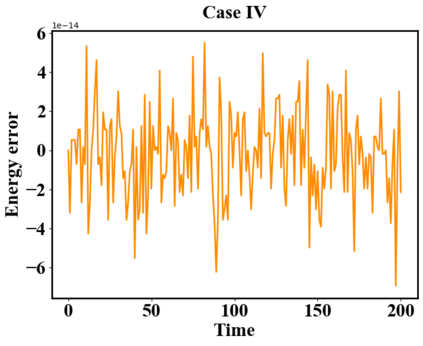
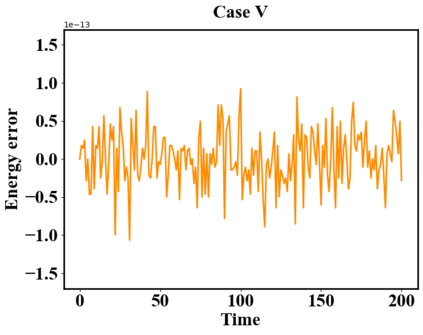
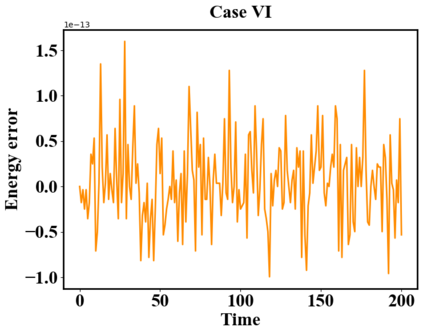
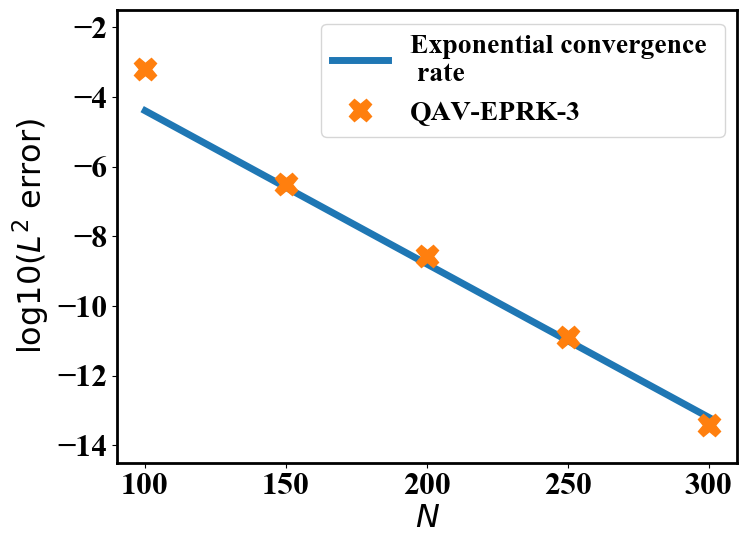
In this paper, we present a quadratic auxiliary variable approach to develop a new class of energy-preserving Runge-Kutta methods for the Korteweg-de Vries equation. The quadratic auxiliary variable approach is first proposed to reformulate the original model into an equivalent system, which transforms the energy conservation law of the Korteweg-de Vries equation into two quadratic invariants of the reformulated system. Then the symplectic Runge-Kutta methods are directly employed for the reformulated model to arrive at a new kind of time semi-discrete schemes for the original problem. Under the consistent initial condition, the proposed methods are rigorously proved to maintain the original energy conservation law of the Korteweg-de Vries equation. In addition, the Fourier pseudo-spectral method is used for spatial discretization, resulting in fully discrete energy-preserving schemes. To implement the proposed methods effectively, we present a very efficient iterative technique, which not only greatly saves the calculation cost, but also achieves the purpose of practically preserving structure. Ample numerical results are addressed to confirm the expected order of accuracy, conservative property and efficiency of the proposed algorithms.
翻译:在本文中,我们提出了一个二次辅助变量方法,用于为Korteweg-de Vries等方程式开发新的一类节能龙格-库塔方法。 二次辅助变量方法首先被提议将原模型重塑为一个等效系统, 将Korteweg-de Vries等方程式的节能法转换成两个全离散的节能办法。 然后, 中位龙格- 库塔方法直接用于重塑模型, 以便为最初的问题达成一种新的半分解时间计划。 在一致的初步条件下, 提议的方法被严格证明维持了Korteweg- del Vries等方程式的原始节能法。 此外, 四重的假光谱法用于空间离散化, 从而形成完全离散的节能办法。 为了有效执行拟议的方法, 我们提出了一种非常高效的迭代技术, 不仅大大节省了计算成本, 而且还实现了实际保存结构的目的。 宽度数字结果将用来证实预期的精确性、 保守性、 和 效率。