Convexification for a CIP for the RTE]{Convexification Numerical Method for a Coefficient Inverse Problem for the Radiative Transport Equation

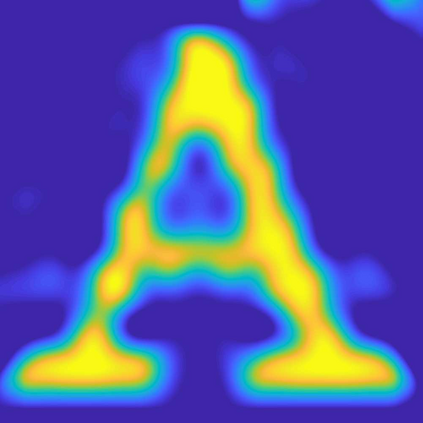
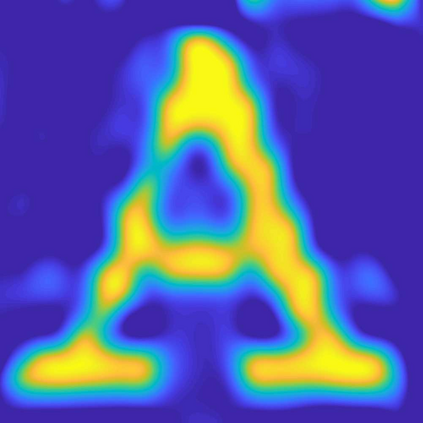
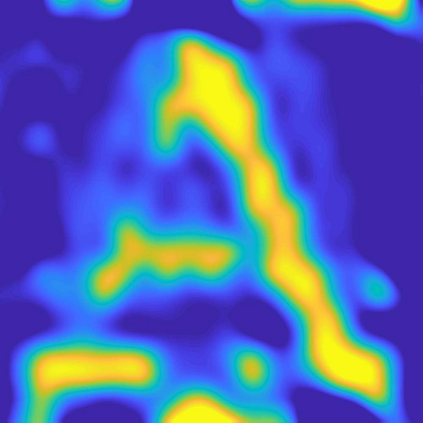
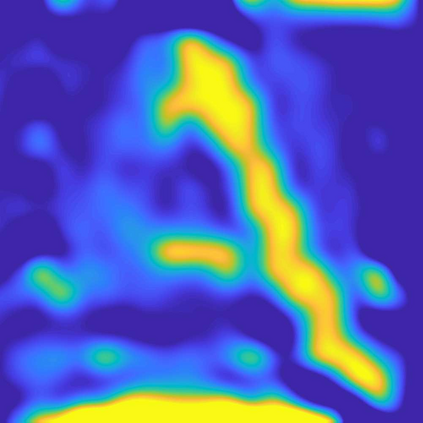
An $\left( n+1\right) -$D coefficient inverse problem for the radiative stationary transport equation is considered for the first time. A globally convergent so-called convexification numerical \ method is developed and its convergence analysis is provided. The analysis is based on a Carleman estimate. In particular, convergence analysis implies a certain uniqueness theorem. Extensive numerical studies in the 2-D case are presented.