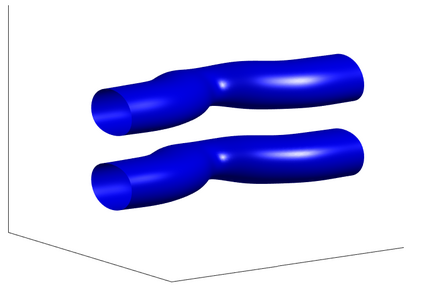
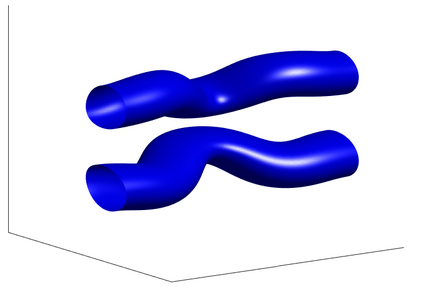
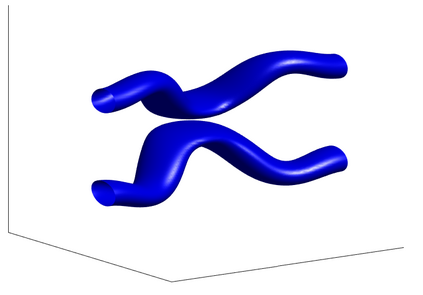
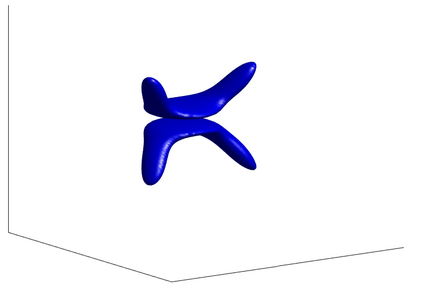
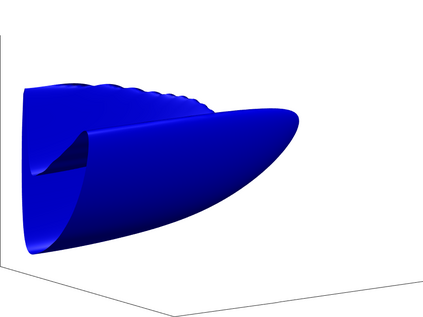
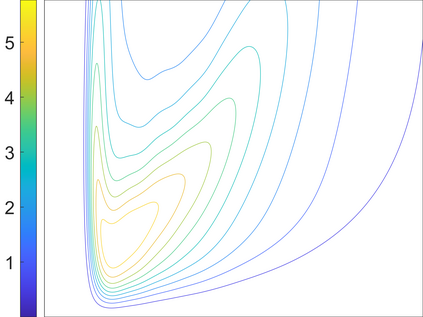
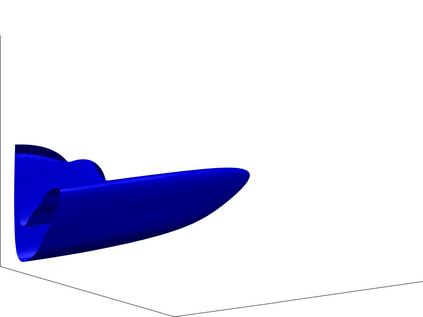
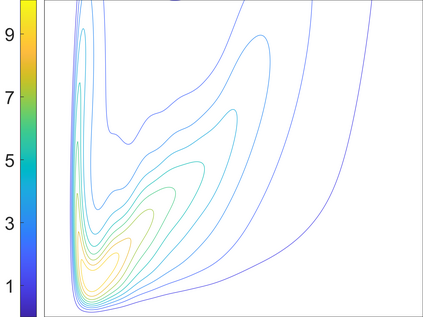
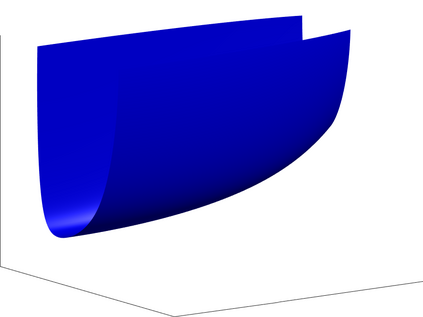
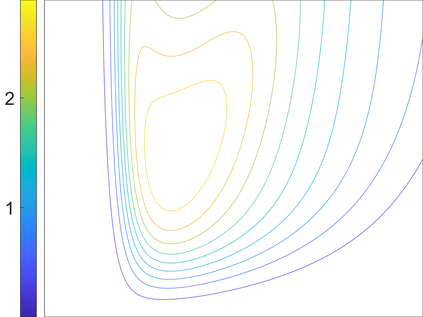
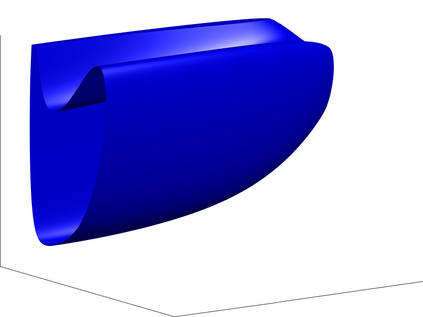
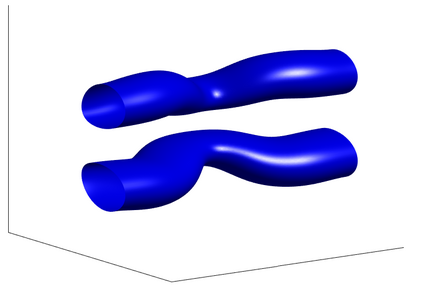
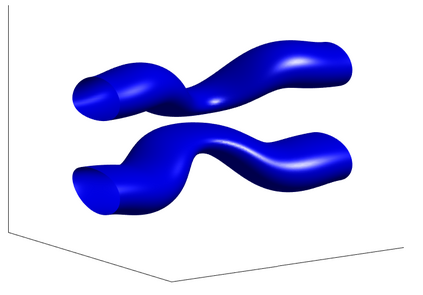
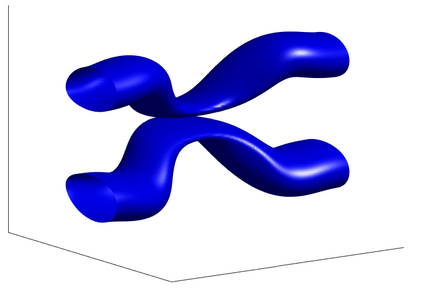
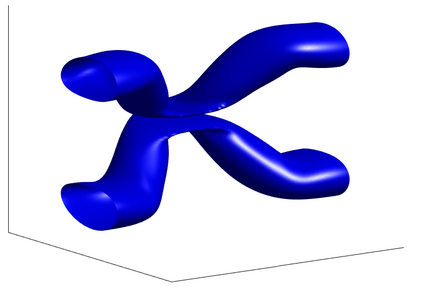
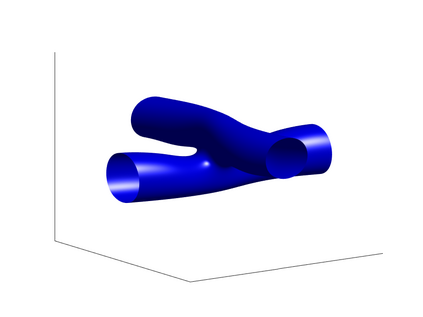
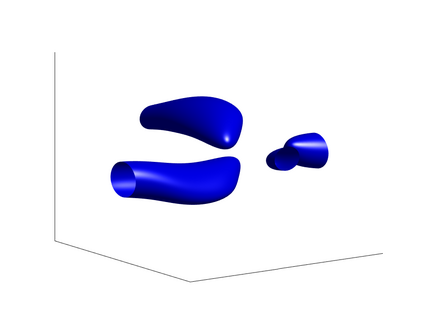
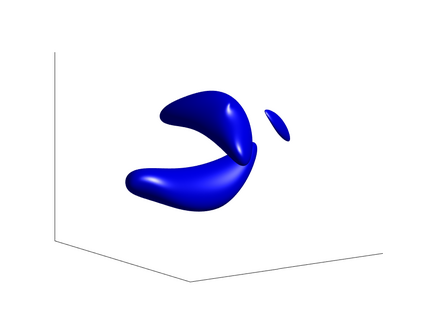
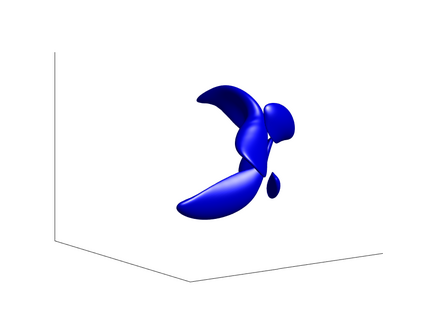
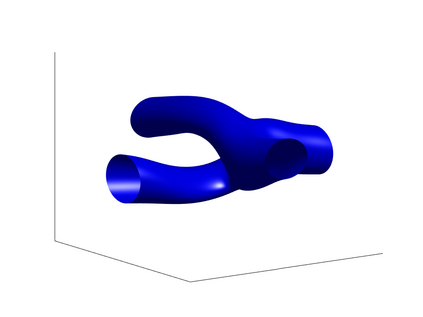
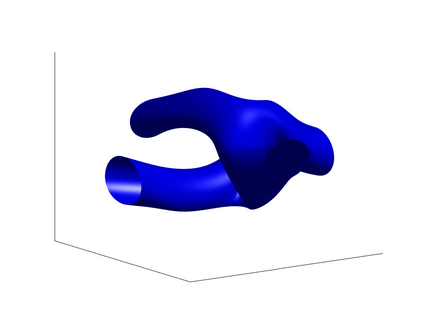
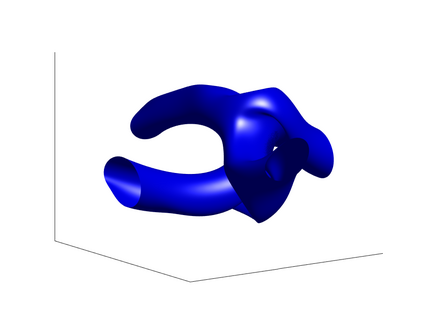
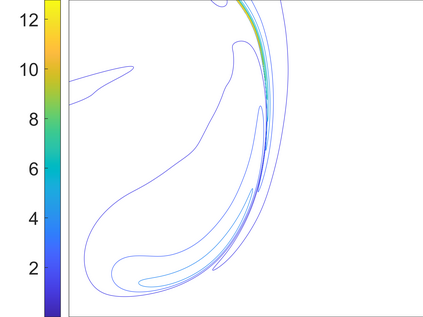
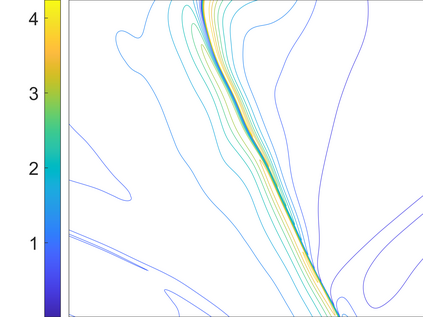
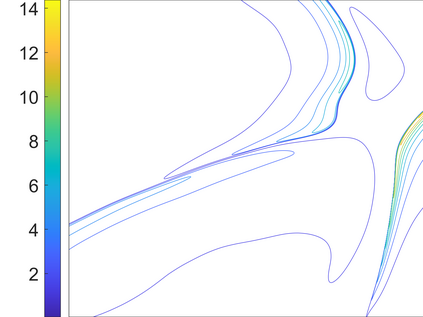
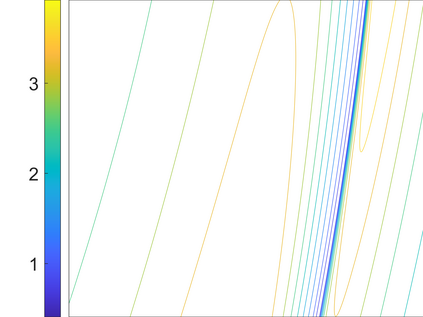
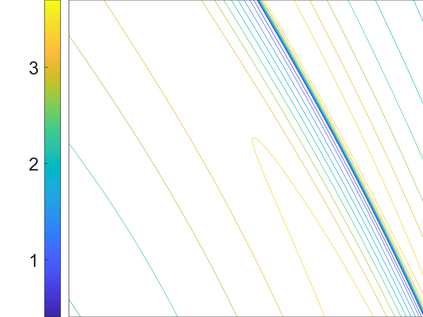
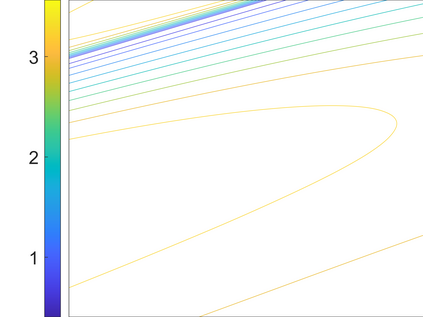
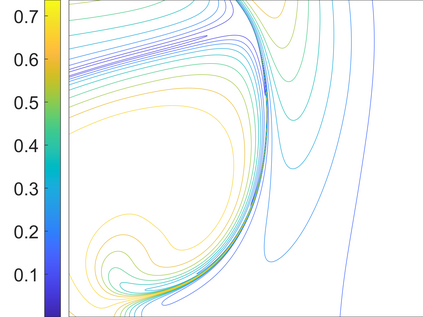
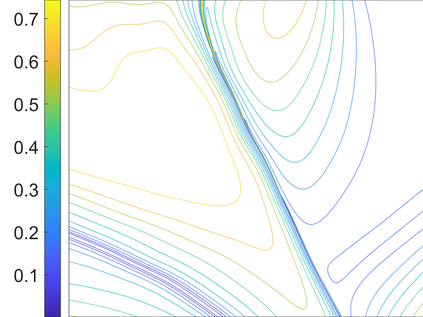
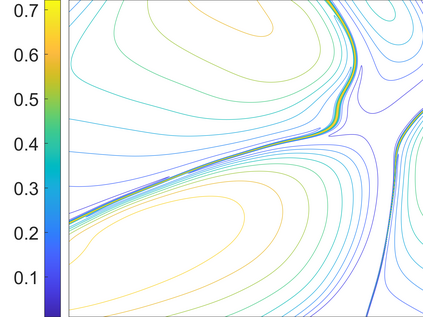
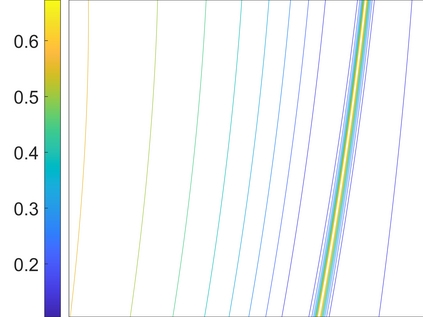
We propose an efficient semi-Lagrangian Characteristic Mapping (CM) method for solving the three-dimensional (3D) incompressible Euler equations. This method evolves advected quantities by discretizing the flow map associated with the velocity field. Using the properties of the Lie group of volume preserving diffeomorphisms SDiff, long-time deformations are computed from a composition of short-time submaps which can be accurately evolved on coarse grids. This method is a fundamental extension to the CM method for two-dimensional incompressible Euler equations [51]. We take a geometric approach in the 3D case where the vorticity is not a scalar advected quantity, but can be computed as a differential 2-form through the pullback of the initial condition by the characteristic map. This formulation is based on the Kelvin circulation theorem and gives point-wise a Lagrangian description of the vorticity field. We demonstrate through numerical experiments the validity of the method and show that energy is not dissipated through artificial viscosity and small scales of the solution are preserved. We provide error estimates and numerical convergence tests showing that the method is globally third-order accurate.
翻译:我们建议一种有效的半Lagrangian 特征映射(CM) 方法,用于解决三维(3D)不压缩的 Euler 方程式。 这种方法通过将与速度场相关的流动图分解而演变成斜体数量。 使用保存二异形的体积的立方体群的特性, 长期变形是从短时间子映射组的构成中计算出来的, 可以在粗差网格上精确地演化。 这种方法是二维不压缩的 Euler 方程式 [51] CM 的根本性延伸。 我们在三维方程式中采用几何方法, 即“ 单体” 不是一个斜体的倾斜体, 而是通过特征图初始条件的拉回, 可以用差2形来计算。 这一配方基于 Kelvin 循环的原体形图, 并给出对园艺域的点性描述。 我们通过数字实验来证明该方法的有效性, 并表明能源不会通过人工反射度和小尺度的聚合法的精确度测量。 我们提供了全球解决方案的第三个尺度的数值测试。
相关内容
- Today (iOS and OS X): widgets for the Today view of Notification Center
- Share (iOS and OS X): post content to web services or share content with others
- Actions (iOS and OS X): app extensions to view or manipulate inside another app
- Photo Editing (iOS): edit a photo or video in Apple's Photos app with extensions from a third-party apps
- Finder Sync (OS X): remote file storage in the Finder with support for Finder content annotation
- Storage Provider (iOS): an interface between files inside an app and other apps on a user's device
- Custom Keyboard (iOS): system-wide alternative keyboards
Source: iOS 8 Extensions: Apple’s Plan for a Powerful App Ecosystem