Conforming, nonconforming and DG methods for the stationary generalized Burgers- Huxley equation

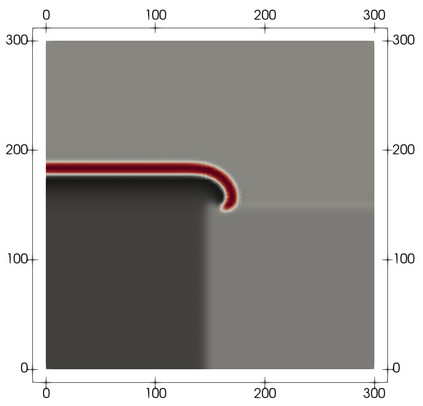
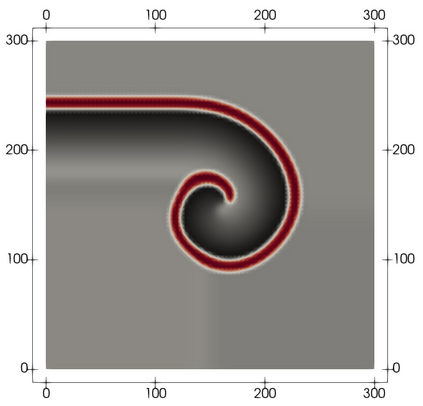
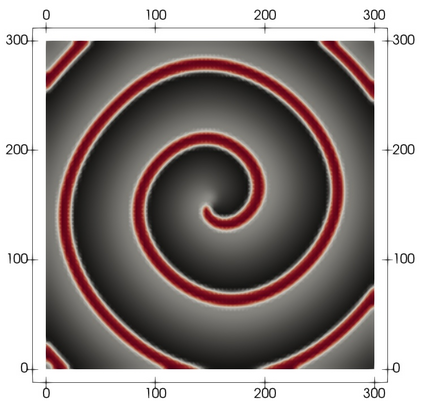
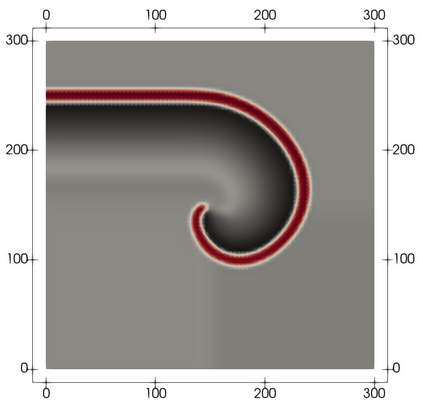
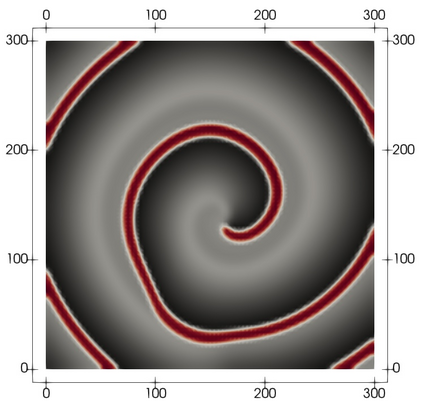
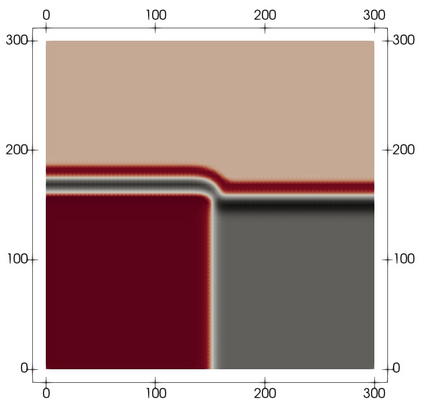
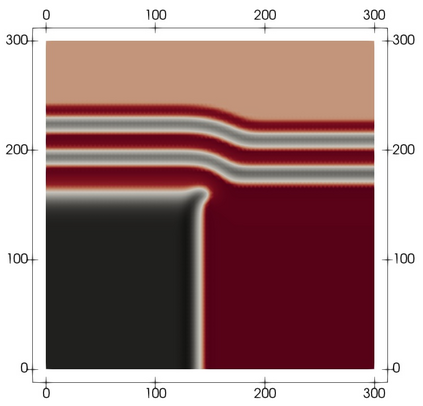
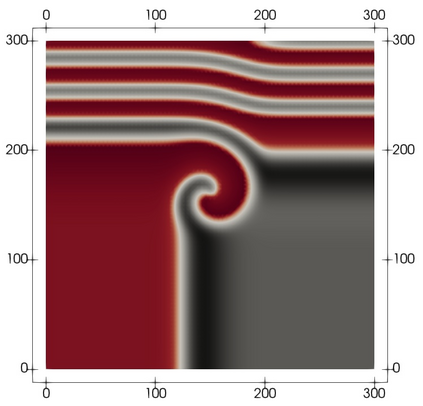
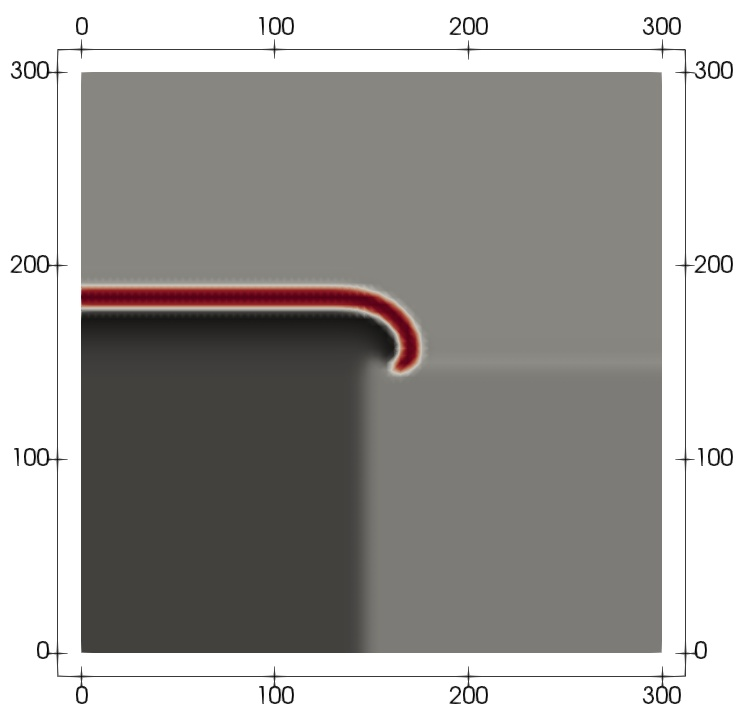
In this work we address the analysis of the stationary generalized Burgers-Huxley equation (a nonlinear elliptic problem with anomalous advection) and propose conforming, nonconforming and discontinuous Galerkin finite element methods for its numerical approximation. The existence, uniqueness and regularity of weak solutions is discussed in detail using a Faedo-Galerkin approach and fixed-point theory, and a priori error estimates for all three types of numerical schemes are rigorously derived. A set of computational results are presented to show the efficacy of the proposed methods.