Combining p-multigrid and multigrid reduced in time methods to obtain a scalable solver for Isogeometric Analysis

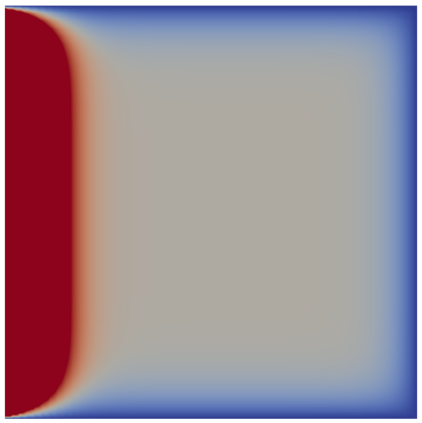
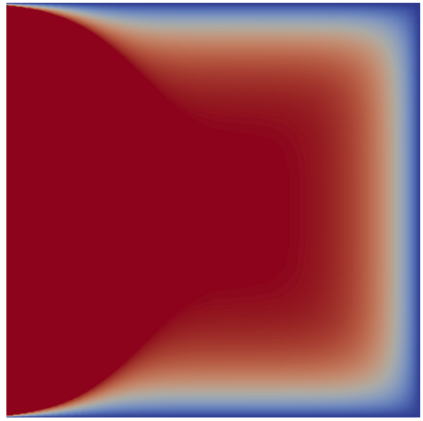
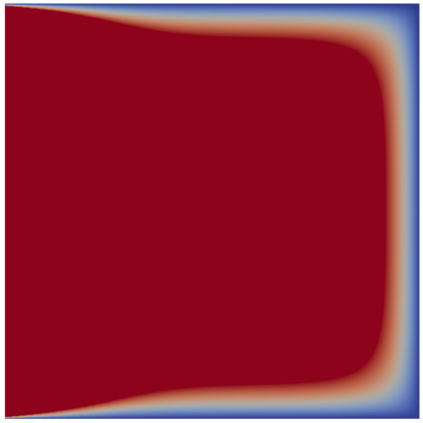
Isogeometric Analysis (IgA) has become a viable alternative to the Finite Element Method (FEM) and is typically combined with a time integration scheme within the method of lines for time-dependent problems. However, due to a stagnation of processors clock speeds, traditional (i.e. sequential) time integration schemes become more and more the bottleneck within these large-scale computations, which lead to the development of parallel-in-time methods like the Multigrid Reduced in Time (MGRIT) method. Recently, MGRIT has been succesfully applied by the authors in the context of IgA showing convergence independent of the mesh width, approximation order of the B-spline basis functions and time step size for a variety of benchmark problems. However, a strong dependency of the CPU times on the approximation order was visible when a standard Conjugate Gradient method was adopted for the spatial solves within MGRIT. In this paper we combine MGRIT with a state-of the-art solver (i.e. a p-multigrid method), specifically designed for IgA, thereby significantly reducing the overall computational costs of MGRIT. Furthermore, we investigate the performance of MGRIT and its scalability on modern copmuter architectures.